Talk:Truncated 24-cells
| This is the talk page for discussing improvements to the Truncated 24-cells article. This is not a forum for general discussion of the article's subject. |
Article policies
|
| Find sources: Google (books · news · scholar · free images · WP refs) · FENS · JSTOR · TWL |
| This article is rated Start-class on Wikipedia's content assessment scale. It is of interest to the following WikiProjects: | |||||||||||
| |||||||||||
The Dual to the Bitruncated 24-cell
[edit]Has somebody seen the following semiregular polychoron ?
It seems to be the dual of the Bitruncated 24-cell. It has 288 congruent cells (tetrahedra), 576 congruent isosceles triangles, 192 long and 144 short edges, and 48 vertices. Just the other way around as the Bitruncated 24-cell.
The vertices are the binary octahedral group and as quaternions lie on the 3-sphere. According to the usual nomenclation it should be called 288-cell. But this cannot be googled nor wikied. -- http://de.wikipedia.org/wiki/User:Nomen4Omen — Preceding unsigned comment added by Nomen4Omen (talk • contribs) 15:31, 24 March 2012 (UTC)
Stella4D (software) can construct the dual of the bitruncated 24-cell, and show orthogonal and perspective projections. I could generate an image, but no clear view choice. Secondly, it is not called a 288-cell, or traditionally only the six convex regular polychorons are named this way. So the only name I'd have is dual of the bitruncated 24-cell. Tom Ruen (talk) 22:21, 24 March 2012 (UTC)
That's really great. In any case, I would be interested in such an image, maybe in perspective projection. Preferably in a style as it is found in other 4-polytope articles.
Of course, it should be entered in the article as a separate section. But so sorry, as long as we do not find it in the literature, it is Wikipedia:No original research. The same is true for baptizing.
I summarize my research in the following, where some entries in the table are not yet consolidated.
- I edited a bit. It's not a uniform polychoron, since that would require regular facets, not just congruent. I think it could make sense to add information about the dual in a subsection here. This is done on other articles, like snub_heptagonal_tiling#Dual_tiling. Tom Ruen (talk) 23:18, 25 March 2012 (UTC)
p.s. Jonathan Bowers calls the bitruncated 24-cell as tetracontaoctachoron for 48-cell, given it is cell-transitive, so by the greek naming, the dual would be dihectaoctacontaoctachoron. (No google matches, but there's one match for dihectaoctacontaoctagon for a 288-gon.) Tom Ruen (talk) 21:18, 1 April 2012 (UTC)
Hi, no name for you but I believe the polychoron you are talking about is quite interesting. Firstly, as a quaternion it represents all the 90 degree angles, i.e. axis aligned orientations (which you can confirm are 48 on the quat double cover). Tom Lowe.
- We've been discussing this on Google+ (https://plus.google.com/u/0/117663015413546257905/posts/9we8M5MDXfs) and I thought I'd add these remarks, which should someday be incorporated into Wikipedia's information about this very nice 48-vertex polychoron:
- I think there's a bit about this 48-vertex polytope in Coxeter's Regular Polytopes (even though it's not a regular polytope). Unfortunately I didn't bring that book with me to Singapore this summer.
- I think he points out that these 48 vertices can be grouped into two bunches of 24, each of which form the vertices of a 24-cell. The idea of the proof goes like this. The tetrahedron has 12 rotational symmetries, so if we take the double cover of this we get a group of 24 unit quaternions. These are the vertices of the 24-cell. But the group of rotational symmetries of the tetrahedron is a subgroup of that for the cube, which is twice as big. Taking double covers, the vertices of the 24-cell become a subgroup of the 48-element group that double covers the rotational symmetry group of the cube.
- So, we can take the 48 vertices of this shape and think of them as the vertices of the 24-cell, together with the vertices of a rotated 24-cell. To get this (4d) rotation, we just take any rotational symmetry of the cube that's not a symmetry of the tetrahedron, take a unit quaternion that represents is, and left multiply by that. User:John Baez 6:02, 8 July 2013 (UTC)
288-cell (so far a working title)
[edit]288-cell Orthogonal projections in B2 and B3 Coxeter planes | ||
|---|---|---|
| Type | perfect[1] polychoron | |
| Symbol | f1,2F4[1] (1,0,0,0)F4 ⊕ (0,0,0,1)F4[2] | |
| Coxeter | ||
| Cells | 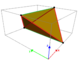 288 congruent tetragonal disphenoids | |
| Faces | 576 congruent isosceles (2 short edges) | |
| Edges | 336 | 192 of length 144 of length |
| Vertices | 48 | |
| Vertex figure |  48 triakis octahedron, | |
| Dual | Bitruncated 24-cell | |
| Coxeter group | Aut(F4), [[3,4,3]], order 2304 | |
| Orbit vector | (1, 2, 1, 1) | |
| Properties | convex, isochoric | |
The 288-cell, or dihectaoctacontaoctachoron, is the dual of the bitruncated 24-cell. It is a 4-dimensional polytope (or polychoron) derived from the 24-cell. It is constructed by doubling and rotating the 24-cell, then constructing the convex hull.
The vertices of the 288-cell are precisely the 24 Hurwitz unit quaternions with norm squared 1, united with the 24 vertices of the dual 24-cell with norm squared 2, projected to the unit 3-sphere. These 48 vertices correspond to the binary octahedral group.
Thus, the 288-cell is the only non-regular 4-polytope which is the convex hull of a quaternionic group, disregarding the infinitely many dicyclic (same as binary dihedral) groups; the regular ones are the 24-cell (≘ 2T) and the 120-cell (≘ 2I). (The 16-cell corresponds to the binary dihedral group 2D2.)
Being the dual of a uniform polychoron, it is cell-transitive, consisting of 288 congruent tetragonal disphenoids. In addition, it is vertex-transitive under the group Aut(F4)[2].
The inscribed 3-sphere has radius 1/2+√2/4 ≈ 0.853553 and touches the 288-cell at the centers of the 288 tetrahedra which are the vertices of the dual bitruncated 24-cell.
The vertices can be coloured in 2 colours, say red and yellow, with the 24 Hurwitz units in red and the 24 duals in yellow, the yellow 24-cell being congruent to the red one. Thus the product of 2 equally coloured quaternions is red and the product of 2 in mixed colours is yellow.
There are 192 long edges with length 1 connecting equal colours and 144 short edges with length √2–√2 ≈ 0.765367 connecting mixed colours. 192*2/48 = 8 long and 144*2/48 = 6 short, that is together 14 edges meet at any vertex.
The 576 faces are isosceles with 1 long and 2 short edges, all congruent. The angles at the base are arccos(√4+√8/4) ≈ 49.210°. 576*3/48 = 36 faces meet at a vertex, 576*1/192 = 3 at a long edge, and 576*2/144 = 8 at a short one.
The 288 cells are tetrahedra with 4 short edges and 2 antipodal and perpendicular long edges, one of which connects 2 red and the other 2 yellow vertices. All the cells are congruent. 288*4/48 = 24 cells meet at a vertex. 288*2/192 = 3 cells meet at a long edge, 288*4/144 = 8 at a short one. 288*4/576 = 2 cells meet at a triangle.
| Region | Layer | Latitude | red | yellow |
|---|---|---|---|---|
| Northern hemisphere | 3 | 1 | 1 | 0 |
| 2 | √2/2 | 0 | 6 | |
| 1 | 1/2 | 8 | 0 | |
| Equator | 0 | 0 | 6 | 12 |
| Southern hemisphere | –1 | –1/2 | 8 | 0 |
| –2 | –√2/2 | 0 | 6 | |
| –3 | –1 | 1 | 0 | |
| Total | 24 | 24 |
Placing a fixed red vertex at the north pole (1,0,0,0), there are 6 yellow vertices in the next deeper “latitude” at (√2/2,x,y,z), followed by 8 red vertices in the latitude at (1/2,x,y,z). The next deeper latitude is the equator hyperplane intersecting the 3-sphere in a 2-sphere which is populated by 6 red and 12 yellow vertices.
Layer 2 is a 2-sphere circumscribing a regular octahedron whose edges have length 1. A tetrahedron with vertex north pole has 1 of these edges as long edge whose 2 vertices are connected by short edges to the north pole. Another long edge runs from the north pole into layer 1 and 2 short edges from there into layer 2.
References
[edit]- ^ a b On Perfect 4-Polytopes Gabor Gévay Contributions to Algebra and Geometry Volume 43 (2002), No. 1, 243-259 ] Table 2, page 252
- ^ a b Quaternionic Construction of the W(F4) Polytopes with Their Dual Polytopes and Branching under the Subgroups W(B4) and W(B3) × W(A1) Mehmet Koca 1, Mudhahir Al-Ajmi 2 and Nazife Ozdes Koca 3 Department of Physics, College of Science, Sultan Qaboos University P. O. Box 36, Al-Khoud 123, Muscat, Sultanate of Oman, p.18. 5.7 Dual polytope of the polytope (0, 1, 1, 0)F4 = W(F4)(ω2+ω3)
Prior discussions of the dual of the 48-cell
[edit]75.137.36.66 (talk) 22:04, 24 September 2012 (UTC)Loren Eric Sepulveda75.137.36.66 (talk) 22:04, 24 September 2012 (UTC)24 September 201275.137.36.66 (talk) 22:04, 24 September 2012 (UTC) The polychoron which you describe has been the subject of several papers by Professor Gabor Gevay, somewhere in a Central European obscure journal, but his articles describing the "48-vertex" stayed on the internet for several years. Professor Branko Grunbaum has also dealt with this polychoron in several papers. Your polychoron has been of acute interest to several theoretical physics people, including Tony Smith, Garrett Lisi, and Professor John Baez. You will have better luck in accessing prior art if you use Google/scholar rather than simple Google. Best regards. So yes, many people have written about your 48-vertex, and many more soon will because it is highly relevant to primordial unification and a variant of the special unitary group SU(7) as well as the related unification group F4. These unification groups were discussed at length in the "Last Workshop on Grand Unifica-tion", held in Chapel Hill, NC.
- I found one paper. Tom Ruen (talk) 20:35, 25 September 2012 (UTC)
- On Perfect 4-Polytopes Gabor G´evay Contributions to Algebra and Geometry Volume 43 (2002), No. 1, 243-259
- Listed in Table 2: f1,2F4 288 tetragonal disphenoids: (48, 336, 576, 288) elements, (1, 2, 1, 1) orbit vector
- @Tom Ruen: That's really great advance.
- But still a christian name is missing. Gévay calls it simply f1,2F4.
- I also saw that the tokens of the CDD-diagrams are your work. Good job!
- --Nomen4Omen (talk) 13:25, 28 September 2012 (UTC)
- Here's another paper March 2012 Quaternionic Construction of the W(F4) Polytopes with Their Dual Polytopes and Branching under the Subgroups W(B4) and W(B3) × W(A1) Mehmet Koca1, Mudhahir Al-Ajmi2 and Nazife Ozdes Koca3 Department of Physics, College of Science, Sultan Qaboos University P. O. Box 36, Al-Khoud 123, Muscat, Sultanate of Oman. 5.7 Dual polytope of the polytope (0, 1, 1, 0)F4 = W(F4)(w2+w3) Tom Ruen (talk) 00:49, 1 October 2012 (UTC)
The dual of the bitruncated 24-choron
[edit]Octagonny 'o3x4x3o' is my name for the bi-truncated 24choron, since its faces represent one of the polyhedra that can be used like the poincare dodecahedron, except that you get 48 truncated cubes. It's a class two system, which means that it's the solution of quadratic equations. The other solution is the conjucate 'octagrammy' o3x4/3x3o. This is a starry thing of density 73.
The dual of octagonny is unsupprisingly, the wythoff-mirror-edge polytope, o3m4m3o, which has 48 vertices (belonging to dual x3o4o3o), and 288 faces. Its isomorph is o3m4/3m4o, which has the same 48 vertices, but 288 (twe 2.48) faces. These two are topologically identical, but the edges of one, but one of the x3o4o3o are inverted relative to the other, so all octagons appear as octagrams.
The o3m4m3o is a catalan polychoron, in that all faces are identical, and all margin-angles (between the faces) are equal. This comes from being the dual of a uniform. The faces of a o3m4m3o correspond to the vertex figure of o3x4/3x3o, and the o3m4/3m3o correspond to the vertex figure of a o3x4x3o. Correspondingly, they tile 4-space, in the same way that octagons tile 2-space.
Importantly, as the tilings of octagons in the 2d complex plane represent the cyclotomic numbers CZ4, the tiling of o3x4x3o in the quarterion plane represent the span of a set of closed quarterions, of class 2. We should note that their units, like the units that represent x3o3o5o, represent closed sets that have as their cells the poincare dodecahedron and 'truncated cube'.
The o3m4m3o also has cells whose vertices represent the vertex-figure of a bi-octagonal prism, and in four dimensions, there is a pair of uniform tilings of my discovery, that are formed by bi-octagonal prisms, 288 (twe 2.48) to a vertex, and its dual of o3x4x3o, of which 64 make a bi-octagonal tegum (dual of bi-octagonal prism) as vertex figure. This corresponds to none of the wythoff groups, since it admits an octagonal mirror-group.
There are references in polygloss and in my paper on the subject. Wendy.krieger (talk) 10:06, 5 October 2013 (UTC)
Name suggestion?
[edit]What this does not miss is correct. It's a frightfully interesting figure.
The name for it is 'bi-apiculated 24choron'. Bi-apiculation is the dual of bi-truncation, and consists of creating faces around an edge of one of the dual regulars, with the N-3 element of the other of the regulars. Its edges fall into hexagons and octagons, there being 48*6/8 = 36 octagons, and 48*8/6 = 64 hexagons, or the squares of 6 and 8 (the vertices of the octahedron). This is no co-incidence, since the space of great arrows is a 3sphere-3-sphere prism (in 6 dimensions), the vertices fall at the 36 vertices of an octahedron-octahedron prism, and the 64 at the cube-cube prism.
It is itself a unit group of a class-2 quarterion system (i designate this 'hr'), which like class-2 cyclotomic numbers is simply dense (a different theory of infinity applies, fraid). The other class-2 group is formed by the {3,3,5} vertices, these lead to the poincare tC and dodecahedron.
It tiles 4-space in a piecewise discrete manner: that is, like the octagon, it is possible to complete its vertex-figure. Like the octagon, the tiling of o3x4x3o has the dual of o3x4/3x3o.
The bi-apiculated 24choron appears as the vertex-figure of a hyperbolic tiling in H4, of bi-octagonal prisms.
Wendy.krieger (talk) 10:57, 5 October 2013 (UTC)
- I see Mathworld has Cumulation as the dual of truncation [1]. Maybe apiculation has a similar origin? ... I see Cumulation or apiculation are the duals of truncation, adding a new point at the center a polyhedron face. So bi-cumulation or bi-apiculation can be defined as the dual of bitruncation as well.
- The Catalan solids use kis prefix for the truncation duals, like triakis as dividing triangular faces into 3 triangles by adding a center point. So the 4D equivalent for the truncated 24-cell dual could be icosikaitetrakis 24-cell.
- And along with the Catalans wouldn't name the duals by their vertex count like bi-apiculated 24-cell. I see this "middle" truncated form






 is like the middle rectified polyhedra, like
is like the middle rectified polyhedra, like 



 is the icosidodecahedron and dual
is the icosidodecahedron and dual 



 is a rhombic triacontahedron (30-sided rhombus-faced polyhedron), similarly for
is a rhombic triacontahedron (30-sided rhombus-faced polyhedron), similarly for 



 and
and 



 as rhombic n-hedra, even if rhombic hexahedron is also a cube. So I see this highly suggests
as rhombic n-hedra, even if rhombic hexahedron is also a cube. So I see this highly suggests 





 can be called a disphenoidal 288-cell, given the vertex figure of the bitruncates are tetragonal disphenoids, which are self-dual.
can be called a disphenoidal 288-cell, given the vertex figure of the bitruncates are tetragonal disphenoids, which are self-dual. - I would proprose these descriptive names as unique and consistent:
- bitruncated 5-cell






 --> dual as disphenoidal 30-cell
--> dual as disphenoidal 30-cell 





 (tetragonal disphenoidal cells)
(tetragonal disphenoidal cells) - bitruncated 24-cell






 --> dual as disphenoidal 288-cell
--> dual as disphenoidal 288-cell 





 (tetragonal disphenoidal cells)
(tetragonal disphenoidal cells) - bitruncated tesseract






 --> dual as disphenoidal 96-cell
--> dual as disphenoidal 96-cell 





 (digonal disphenoidal cells)
(digonal disphenoidal cells) - bitruncated 120-cell






 --> dual as disphenoidal 3600-cell
--> dual as disphenoidal 3600-cell 





 (digonal disphenoidal cells)
(digonal disphenoidal cells)
- bitruncated 5-cell
Attacking the higher dimensions by guessing what happens in the lower ones is not a good idea, and one is probably better off trying to think in terms of higher-dimensional operators to form these names. For this one needs to devise a wordlist where the etymologies are kept to their general root meanings, and not let flights of fantasy about seeing 4d ruin 5d and higher. For example, Bowers term 'prismo' correctly describes forming prisms between the faces of duals in 4d, but this term needs to be kept moving with the dimensions, (ie 0, N-1), rather than (0,3).
I'm not terribly fussed with this way of doing things. Names that work well in 2d and 3d, generally fall apart as one goes to higher dimensions. kis in triakis and so on, means the same as ce in once, twice, thrice. It just means 'multiply by'. A triakis octahedron has 3×8 faces, to distinguish it from the 4×6 face tetrakis hexahedron.
Cumulate already has a meaning in english in the sense of 'to amass', 'to gather', 'to sum', 'to end in', the sense of cumulate given by mathworld is that the sun cumulates to its highest point. In any case, pushing cumulate in the sense of the dual of truncate is not what is called for here, because most people are not going to guess that a word that is related to 'accumulate wealth', ought mean the peak of a pyramid. 'apiculate' is in the sense of to raise to peaks is a better option.
So a 'bi-apiculated X' in four dimensions has as its faces, the edges of X, and the edges of dX as opposite bases of a pyramid. The body of the pyramid is formed by every point on lines that begin in one base, and end at the other, ie a disphenoid tetrahedron.
Naming the duals of the truncates by their faces is a bad idea, because you have this series of truncates to all dimensions, but you have to cast the dual-names in every dimension. The series of apiculates can be directly constructed by supposing that the dual is pushing out the skin of a figure (as the dual increases), exposing the vertex, edge, etc of the dual at (ap, bi-ap, etc), and that the faces of the appropriate apiculate are formed by the M-1 element of the dual, by the N-M-2 element of the figure in trackem (or pyramid) product. You then have the dual of the x-truncated A is the x-apiculated dualA.
rhombus does not translate well in higher dimensions: the reflex of a square in 3d is variously the cube (ie parallelotope of equal edges), and the octahedron (what you get by putting vertices at (1,0,0,..), (0,1,0,..), etc). Likewise rhombus suffers this. The higher dimension reflex of the faces formed by o3m5o etc, tells us that the faces are tegums (duals of prisms), of the crossing surtope and orthosurtope.
So i'd stick with 'bi-apiculated 24choron', becuase it's the direct dual of 'bi-truncated 24choron' without further fuss, and just as truncation can be described generally, so can apiculation. Wendy.krieger (talk) 08:47, 6 October 2013 (UTC)
- I'm still comfortable with the simple 288-cell if it was was unique, used for regular polychora, and sensible for cell-transitives as well, so there's just ambiguity if the cell count is repeated. From the uniform polychorons, only the cantellated 24-cell has 288 vertices, but it has a triangular prism vertex figure, and dual has triangular bipyramidal cells. So it is unique to say disphenoidal 288-cell for this, and triangular bipyramidal 288-cell for the cantellated dual form.
- Oh, one other example, 3D honeycombs are topologically similar to polychora, and dual of bitruncated cubic honeycomb is currently called disphenoid tetrahedral honeycomb, although I see tetrahedral is redundant. I also see Conway calls that dual an 'Oblate tetrahedrille, not that it helps here. Tom Ruen (talk) 10:38, 6 October 2013 (UTC)
- p.s. On ambiguity of 288-cell, I see there's also a p,q duopyramid has pq disphenoid cells, so a 16,18 duopyramid COULD be called disphenoidal 288-cell, (or any of (1,288),(2,144), (3,96), (4,72 ), (6,48) (8,36), (9,32) or 12,24 as well) although it would be silly since they have special names. Tom Ruen (talk) 13:09, 6 October 2013 (UTC)
- When you look at the 'pentagonal bipyramid' in 3d, it is usually concieved as "two pentagonal pyramids, base to base". You can just as readily see it as "five disphenoid tetrahedra around the polar axis". In fact, in the apiculation process, as the edge of 3,3,5 crosses the dodecahedral faces of 5,3,3, the pentagonal pyramids of the apiculated 5,3,3 merge into a pentagon-line tegum, and then divide into "five disphenoid tetrajehra around the edge of 3,3,5", as that edge rises above the pentagons.
- It's pretty straight forward to read 'bi (* -> o) + apiculated ~mm* + 24choron 3,4,3 as follows. 1: replace * by a single 'o', ie ~mmo. 2: fill out ~ with 'o' to meet the dimension (ie ommo), 3, put digits of regular figure in, ie o3m4m3o, whence it is apparent that the faces have o o symmetry, and therefore a quarter of the group, ie 972 / 4 = 248 = dec 288, the faces are line-line pyramids. The rules are kept here: http://teamikaria.com/hddb/forum/viewtopic.php?f=25&t=1726&p=19113#p19113 Wendy.krieger (talk) 07:33, 7 October 2013 (UTC)
- I also like very much Tom Ruen's disphenoidal 288-cell. It points out the most important characteristics: 288-disphenoid. In the sense that it is not an n-cell, because it's not regular, and the "cells" are disphenoids. --Nomen4Omen (talk) 15:07, 30 April 2014 (UTC)
- It's pretty straight forward to read 'bi (* -> o) + apiculated ~mm* + 24choron 3,4,3 as follows. 1: replace * by a single 'o', ie ~mmo. 2: fill out ~ with 'o' to meet the dimension (ie ommo), 3, put digits of regular figure in, ie o3m4m3o, whence it is apparent that the faces have o o symmetry, and therefore a quarter of the group, ie 972 / 4 = 248 = dec 288, the faces are line-line pyramids. The rules are kept here: http://teamikaria.com/hddb/forum/viewtopic.php?f=25&t=1726&p=19113#p19113 Wendy.krieger (talk) 07:33, 7 October 2013 (UTC)
Equator of the Disphenoidal 288-cell
[edit]Strangely enough, I am not able to find the polyhedron which lies in the equator-hyperplane of the disphenoidal 288-cell. It is closely related to the octahedron, but at the place of 1 face (a regular triangle) there are 4 triangles so that it is a 32-hedron. The vertices are 6 of type (±1,0,0) and 12 of type (±√2/2,±√2/2,0). --Nomen4Omen (talk) 18:32, 26 May 2014 (UTC)
- This polyhedron is a cuboctahedron, whose 6 square faces are replaced by pyramids. The hight of these pyramids is determined by the condition that the 18 vertices are all on one sphere. Thus, the faces are the 8 equilateral triangles of the cuboctahedron plus the 6•4 congruent isosceles triangles of the pyramids. Rolfdieter Frank (talk) 11:18, 6 October 2021 (UTC)
Orthogonal projection of the disphenoidal 288-cell onto the Coxeter plane F4
[edit]I think the picture of this projection in the article is not correct. Namely, it shows the vertices of a 24-cell (red) and the centers of its cells (yellow). But the 48 vertices of a 288-cell are vertices of two congruent 24-cells. Thus, in a correct projection, the yellow vertices should be on the same two circles as the red vertices are. In other words, the yellow vertices are obtained from the red ones by a rotation through 15 degrees.
Thanks for the otherwise very nice article Rolfdieter Frank (talk) 14:16, 22 September 2021 (UTC)
- I agree. As quaternionic group the 24-cell is:
- (red).
- The group is the union of with
- (yellow).
- In any projection, this would mean that the vertices of and are almost undistinguishable, have the same number of connections etc, but in the picture in the article I count for a red vertice 6 edges to red vertices and 2 edges to yellow vertices or 8 edges to red vertices and 2 edges to yellow vertices, whereas for a yellow vertice I count 8 edges to yellow vertices and 4 edges to red vertices or 10 edges to yellow vertices and 0 edges to red vertices. In fact, the red vertice has 6 edges of length to the red vertices and 8 edges of length to the red vertices and 4 edges of length
- to the yellow vertices
- plus 8 edges of length
- to the yellow vertices
- in the northern hemisphere of
- is isomorhic to the binary octahedral group 2O.
- But I can't imagine that the factor could result in something like a rotation of 15 degrees. Isn't it ? –Nomen4Omen (talk) 18:59, 22 September 2021 (UTC)
- Since the picture has a 30 degree rotational symmetry, a 15 degree rotation and a 45 degree rotation have the same effect. Rolfdieter Frank (talk) 12:18, 23 September 2021 (UTC)
- Thanks! I did understand rotation in 4D – and possibly don't know what "projection onto the Coxeter plane F4" means. So if rotation means 2D rotation you are right, of course.
Btw, the number of edges I gave above are not correct. Correct ones are given in the article. –Nomen4Omen (talk) 17:08, 23 September 2021 (UTC)
- Thanks! I did understand rotation in 4D – and possibly don't know what "projection onto the Coxeter plane F4" means. So if rotation means 2D rotation you are right, of course.
- I see that the picture in the article is missleading in another aspect: It shows only the edges of the red 24-cell. The incidences of the yellow vertices (and even of some red vertices) with these edges are only caused by the projection. Rolfdieter Frank (talk) 19:22, 23 September 2021 (UTC)
- I agree. The vertices in the picture are far too thick. It would be desirable to have all edges somehow distinguishable in the picture. But there are 192 long edges with length 1 connecting equal colours and 144 short edges; this makes up 336 edges. Too many ? An incidence should be marked by a vertex. –Nomen4Omen (talk) 19:36, 23 September 2021 (UTC)
- A solution can be to pick one vertex from each of the two circles and then draw only the 28 (or 27 if the vertices are connected) edges through these two vertices. Then all the other edges are given by the 15 degree rotational symmetry of the picture. Rolfdieter Frank (talk) 08:34, 29 September 2021 (UTC)
Simple coordinates for the bitruncated 24-cell
[edit]One can simplify the coordinates given in the article by multiplying them with sqrt{2}-1. The new simpler coordinates are all permutations of coordinates and sign of (0,sqrt{2},sqrt{2},2) and (1-sqrt{2},1,1,1+sqrt{2}).Rolfdieter Frank (talk) 10:38, 27 September 2021 (UTC) Rolfdieter Frank (talk) 19:45, 23 September 2021 (UTC)


















