Talk:Monty Hall problem/Archive 10

This is an archive of past discussions about Monty Hall problem. Do not edit the contents of this page. If you wish to start a new discussion or revive an old one, please do so on the current talk page.

Archive 5

←

Archive 8

→

New archive

This page has been quiet for a few weeks, so I created a new archive at Talk:Monty Hall problem/Archive 9 for the discussions from mid Oct 2008 through Feb 2009 (indicated as "archived" above). Several of the issues aren't exactly resolved (they will perhaps never be resolved to everyone's satisfaction), but the period of relative calm seemed like an opportune time to create a new archive. I added a new section (post archiving) much like this one. user:Glkanter has reverted these changes, suggesting we talk about it first. OK. Since we're at a lull in the discussion here, and the talk page is huge, I suggest now is an appropriate time to archive. -- Rick Block (talk) 15:14, 15 March 2009 (UTC)

Agree with archiving now. The talk page is monster-sized, and nobody is going to read through it all anyway. –Henning Makholm (talk) 15:29, 15 March 2009 (UTC)

I don't agree there has been a 'lull'. It's just been re-directed to the arguments page. I assume most readers (not necessarily editors) do NOT read archives. If they're interested, maybe they get as far as a talk page. Anyway, I think that the existence and vehement nature of the MHP article disagreement would not be evident if the entire thing were archived. A reader might think that there was universal satisfaction with the construction of the article. That would be an inaccurate impression to give. Glkanter (talk) 15:55, 15 March 2009 (UTC)

This thread will remain here, and it already says there are unresolved issues. If you'd like to enumerate them here that will remain here as well. Good enough? -- Rick Block (talk) 17:37, 15 March 2009 (UTC)

I didn't know there was an "arguments" page, so I too thought it had settled down. It would be very useful to have a concise list here of what the ongoing issues about the article are, phrased as neutrally as possible, with links to where some discussion about them may be found. Dicklyon (talk) 21:38, 15 March 2009 (UTC)

The main one continues to be around whether it's necessary for the problem to be approached as a problem in conditional probabilities (i.e. whether an "unconditional solution" is sufficient). I wrote a FAQ section on this (referenced from the top of this page, see Talk:Monty_Hall_problem/FAQ). -- Rick Block (talk) 21:53, 15 March 2009 (UTC)

Thanks; I just read it; well done. But why is that something we need to argue about or have a consensus on? Can't we just report on who says it needs to be approached that way, and who says it doesn't? Dicklyon (talk) 22:24, 15 March 2009 (UTC)

The issue is there's a faction here (Glkanter and Martin Hogbin, perhaps others) who think the conditional analysis is completely unnecessary and that the problem as generally stated is appropriately addressed by an unconditional solution. They strongly object to Morgan et al.'s characterization of unconditional solutions as "false" solutions. There are others (Nijdam, for one, and I believe most participants in WP:WikiProject Mathematics willing to comment) who object to presenting an unconditional solution as the solution, since (in their view) an unconditional solution isn't actually addressing the problem as stated (and think whether or not the problem is stated to force a 2/3 answer, the unconditional solution is basically wrong). I've been suggesting the folks in favor of presenting an unconditional solution offer up a reference for such a solution from a source that understands the difference between conditional and unconditional. So far, no source has been offered. Most popular sources (notably vos Savant) ignore this issue - one is tempted to conclude most popular sources do not understand it. -- Rick Block (talk) 22:47, 15 March 2009 (UTC)

So, lacking such other sources, we should obviously present the "usual" unconditional solution, with sources, and also the "conditional" solution and the fact of who says it's necessary and that the other is inadequate. Or does someone argue for more than this, or less than this? Dicklyon (talk) 23:28, 15 March 2009 (UTC)

The specific problem with the current article is that it offers a somewhat wordy unconditional solution as the first solution. But then immediately follows that by saying "Although the reasoning above is correct it doesn't answer the precise question posed by the problem,...". Then the lengthy remainder of the article addresses this so-called deficiency. So, if I'm trying to settle a bet (of course using the unconditional solution, just try using the other stuff in a bar), the other guy says to me, 'but Wikipedia says that's not the solution'. So, as long as any claims that the unconditional solution is inadequate remain in the article, I am not for a two-tier solution. Glkanter (talk) 03:29, 16 March 2009 (UTC)

But aren't the "claims that the unconditional solution is inadequate" well sourced? Or are you suggesting that Morgan et al. 1991 should be treated as just an opinion? And are you saying that the "one tier" or unconditional solution is adequate? Based on a source that says so? Or based on the fact that it comes up with the same answer, in terms of player strategy and average probability of winning, even though it's not an air-tight proof? Or based on the "standard analysis" where the host must "open one of the remaining two doors randomly if the player initially picked the car (Barbeau 2000:87)"? In this "standard" interpretation, is the idea that the host's action offers no new information about the probability of the originally chosen door have a car, and therefore it's a non-event and you don't need a conditional analysis? Or what? Could we resolve this by having a section the "standard interpretation" and its standard simple solution, and another section on other interpretations that require the more elaborate analysis? Dicklyon (talk) 04:32, 16 March 2009 (UTC)

As you know, I reject the argument that the unconditional solution is inadequate. Interestingly, you bring up the point that Monty's decision only happens when the contestant chooses the car. Rick mentioned the same thing tonight. I just can't get past the idea that Monty choosing between two losing doors has any affect on the probability of winning. Check out the last section of the Arguments page for the latest and greatest unconditional solution. It contains no goats and no Monty. And, it has been approved as an unconditional solution to the MHP by a Wikipedia guru.

http://wiki.riteme.site/wiki/User_talk:Tsirel#Is_this_a_valid_unconditional_proof_of_the_Monty_Hall_Problem.3F It's the 3rd section from the bottom. Glkanter (talk) 05:43, 16 March 2009 (UTC)

Well, I think we can all agree that "Monty choosing between two losing doors" doesn't have any affect on the probability of winning, normally; but your "proof" is just an assertion with no proof logic, so hardly helpful at convincing anyone that it can be made rigorous. If you add some condition, like that Monty reveals no extra information about the prob of the first door having a car behind it, then it could be made rigorous pretty easily. On the other hand, if you allow Monty to reveal extra info, either by being known to always choose the left-most goat, or by stopping to flip a coin when the contestant has chosen a car, then you've got a different problem, requiring a different solution (in this latter case, the contestant's probability of winning a car is 100% if he follows the optimal strategy of switching only if Monty doesn't flip a coin). Dicklyon (talk) 05:52, 16 March 2009 (UTC)

If you agree that ""Monty choosing between two losing doors" doesn't have any affect on the probability of winning, normally;..." then we're done here. Maybe I don't speak such good probability, but I'm sure you agree that 1/3 of the time the contestant picks the car. And that the probability of 'car' or 'not car' = 1. And that 1 - 1/3 = 2/3. And you just agreed that ""Monty choosing between two losing doors" doesn't have any affect on the probability of winning, normally;...". What's the problem? Why is this unconditional solution inadequate? Please note, the solution makes no mention of a 'Monty' or any 'goats'. What do you make of the comment at the above link? And I agree, the contestant knowing of a left door Monty bias, or flipping a coin is a new constraint to a different problem than the MHP. Glkanter (talk) 06:22, 16 March 2009 (UTC)

I think it reduces to just defining "normally"; and then allowing those who want to pursue the "not so normal" interpretation and variation of the problem to have their say as well. Dicklyon (talk) 06:31, 16 March 2009 (UTC)

There's a problem here, which is that the "proof" is not showing what it purports to show. What it does show is that if the player decides to switch before the host opens a door she has a 2/3 chance of winning. However, the question is what is the chance of winning when deciding to switch after the host opens a door. The problem can be (but often isn't) sufficiently constrained to make these the same. The whole point of the Morgan et al. paper (and the Gillman paper, and this point is echoed in the Grinstead and Snell book and others) is that an unconditional approach is fundamentally the wrong way to approach this kind of problem and using one without qualification is unsound at best (Morgan considers these "false" solutions). My understanding is that Glkanter does not agree with this, and does not want anything like it in the article - no matter how many reliable sources say it. The bottom line is that Morgan et al., and Gillman, and others basically discredit the unconditional approach which means (to me) that Wikipedia should either not present an unconditional "solution" at all, or is obligated to follow it up with the very sorts of words Glkanter objects to. -- Rick Block (talk) 14:45, 16 March 2009 (UTC)

I sort of agree; not because the "proof is not showing what it purports to show", but because we have reliable sources that say so. The simple proof can even be made "correct", based on those same sources I suspect, by showing that the "chance of winning when deciding to switch after the host opens a door" can not change from the "chance of winning when deciding to switch before the host opens a door" under some reasonable and normal set of assumptions. They can both be correct, and both rigorous, if the problem is appropriately formulated. Dicklyon (talk) 16:16, 16 March 2009 (UTC)

As to the idea that one POV should not be presented because it's discredited by another POV (both in reliable sources), not that would violate NPOV. Dicklyon (talk) 16:18, 16 March 2009 (UTC)

The value of the unconditional solution is that it explains the underlying logic (why 2/3 instead of 1/2) in a nice, simple way. If it were offered as an entirely valid analysis of the basic veridical paradox, then followed up with a "however, the problem as stated requires a more complicated solution", we'd have a good article. We're not willing to do this: instead, we say "here's the unconditional solution, which is wrong". We need to say, with no neurosis, "the unconditional solution is right, albeit in a constrained manner". And furthermore we need to focus on offering the unconditional solution in the clearest possible way in order to make the article as useful as possible to the general reader: right now, it's statisticiancruft.

Unfortunately, the "problem as stated" has the attention of this article's editors to a degree that harms our ability to offer a solid, simple explanation to our readers. During the time that I've been involved with this article, I've seen it constantly degrade in quality. There's a pedantry at work that forces every explanation of the unconditional solution we offer to mutate into something that straddles the unconditional and conditional solutions, compromising both accuracy and clarity. We've got to ditch this neurosis and be willing to offer both solutions robustly, while noting the limitations of each.

Drop the textbooks for a second and think about how you would write this article to be useful to an audience of 10-year-olds. Then write that article, and add to it whatever is needed to make it useful to a statistician as well.--Father Goose (talk) 16:47, 16 March 2009 (UTC)

Sounds right to me. Do we have anyone who disagrees? Dicklyon (talk) 18:13, 16 March 2009 (UTC)

What would the "solid, simple explanation" be an explanation of? If an explanation of the "problem as stated," then the editors' attention is correctly directed. If it is an explanation of something else, what is it? The problem as usually understood? Please clarify. Visualaudio (talk) 04:21, 28 March 2009 (UTC)

I agree with a lot that Father Goose said, but not 100%. I refuse to focus on anything but the original 1/3 car and 2/3 not car. And nobody has ever said how the 1/3 can possibly change, so the 2/3 can't change either. There's an old saying, 'Are you interested, or committed? The hen, who provided the eggs, is interested in your breakfast, the pig, who provided the bacon, is committed to your breakfast.' I'm committed to the unconditional solution as being valid, rigorous, complete, etc. My goal all along has been to eliminate any statements in the Article, let alone the Solutions section, that undercuts this. The heck with the 10 year old. Try getting paid off on a bet by explaining the 'equal goat door constraint'. Glkanter (talk) 19:51, 16 March 2009 (UTC)

I more or less agree with that. That's not to say that we need to drop the conditional explanations, the Bayesian analysis, or anything else. It's just that we need to have an absolutely pristine explanation of the unconditional analysis (such as the one diagrammed here) that is wholly untouched by conditional gremlins.

The fact that every unconditional explanation present in the article keeps getting butchered into a conditional explanation (or worse still, a pseudo-conditional one) is a source of anguish to me. I'm serious, it anguishes me. It results in us having an article that is of negative value to the overwhelming majority of our readers, as it fails to give them a nice, simple, accurate, easy-to-understand solution to the paradox in its most general form, and misleads them into thinking that that solution is for some reason an invalid way to analyze the paradox -- which it is not. It's an invalid way to analyze the question-as-usually-asked -- but the question-as-usually-asked is not the subject of the article -- the paradox is. The question-as-usually-asked is merely one of the "sources of confusion", which has led our explanation of the paradox to be a confused one as well.--Father Goose (talk) 22:06, 16 March 2009 (UTC)

Rick, would you be willing to consider a rewrite, with my help if need be, that would allow both the "conditional" and "unconditional" explanations to be fully and clearly presented, without introducing any inaccuracies?--Father Goose (talk) 22:10, 16 March 2009 (UTC)

Propose away. I think this version has the exact structure you're suggesting. I'll also note that even the current version doesn't say the unconditional solution is wrong, just that it doesn't answer the precise question that is asked (which is what the reliable sources actually say). I'd suggest anyone consider rewording the solution consider whether the wording holds for slight variants - like the "host forgets" variant (where the correct answer is probability of winning by switching is 1/2, not 2/3) or the "host opens leftmost door if possible" variant (where the correct answer is probability of winning by switching is 1/2 or 100% depending on which door the host opens). The point of thinking about such variants is to explore how "robust" the solution is. If your solution only works for one carefully constructed version of the problem, it probably doesn't explain anything at all about the underlying logic. -- Rick Block (talk) 01:43, 17 March 2009 (UTC)

That version still contaminates the simple solution section with the statement "Although the reasoning above is correct it doesn't answer the precise question posed by the problem, which is whether a player should switch after being shown a particular open door." It might be better to present a problem that this is the exactly correct solution to, and then treat the other problem where's it's not separately. A simple way to do this is to introduce the condition that the host choose randomly when there are two goats left, in a way that reveals no additional information to the contestant (since, as I pointed out, if he chooses random by flipping a coin only when he has to, and the contestant can see that, then it's a completely different ball game), so that the contestant's strategy of always switching is clearly going to lead to 2/3 chance of winning, without any conditionalizing on which door the host opens. In the diagram that Father Goose linked, I think one can rigorously show that "The player has an equal chance of initially selecting the car, Goat A, or Goat B. Switching results in a win 2/3 of the time." as it says in the caption, and that no strategy of maybe not switching based on which door the host opens can beat that. Dicklyon (talk) 02:25, 17 March 2009 (UTC)

Contaminates? That wording is sourced - the exact quote is "F1's [one unconditional solution's] beauty as a false solution is that is is a true statement! It just does not solve the problem at hand". If someone can come up with a reference for a version of the MHP for which this solution (or any unconditional solution) is the exactly correct solution that'd be great - although one of the points of the Morgan et al. and Gillman papers is that the problem is about conditional probabilities (so I'm kind of skeptical that anyone is going to find such a reference). Per the question I've added to Talk:Monty Hall problem/FAQ, Gillman suggests an unconditional solution would be appropriate for a version where "you need to announce before a door has been opened whether you plan to switch" (which is such an unusual wording I don't think anyone would claim it's still the MHP). Ultimately the only thing that matters here is references. Change the article to say anything you'd like. But base it on references, not WP:OR. -- Rick Block (talk) 04:08, 17 March 2009 (UTC)

Yes, "contaminates" in the sense of the proposal above for a discussion that is "wholly untouched by conditional gremlins." We all agree that there are sources that say such an analysis in inadequate; but it would not be NPOV to rely only on that analaysis. Try this one: [1]. Do we have to all agree that it's mathematically "exactly correct" in order to report what it says? It does look pretty carefully done, as it lists a bunch of assumptions needed to conclude that the host's opening of a door "gives no additional information about the likhihood of the initially chosen door being correct. etc." Dicklyon (talk) 04:17, 17 March 2009 (UTC)

"Do we all have to agree it's mathematically exactly correct"? No, however given that this is a featured article we should clearly do our best to use sources of the highest quality, particularly if we're talking about a source for what will be presented as the "main" solution (which is what I believe some folks are talking about here). This source says the probability of winning by switching is .67 - which (IMO) makes this source somewhat suspect (and if the point is to use this as a "gold standard" for the solution, I would say we can't interpret this "loosely" as 2/3). It's also published as an appendix to vos Savant's book, and listed on the copyright page of the book as a reprint - which brings up the question of where it was originally published. As best as I can tell this appendix is a revised version (not a reprint) of this paper originally published in Personality and Social Psychology Bulletin (I did a lot of the original sourcing for this article, so I have most of the sources - including vos Savant's book and this paper). Note that this is a psychology journal, not a math journal, which makes this an excellent source for psychological aspects of the Monty Hall problem, but a somewhat less excellent source for mathematical aspects (and, I'll note, Granberg is a professor of sociology at the University of Missouri, not a mathematician). Does this mean we can't use this source? No. In fact, it's already referenced in the article (both the original paper and the "reprint" from vos Savant's book). On the other hand, I would hesitate to use this paper as the source for what we intend to be a mathematically sound unconditional solution. -- Rick Block (talk) 14:09, 17 March 2009 (UTC)

Let me ask you, Rick: does this diagram somehow not map perfectly onto a truly unconditional presentation of the problem? If not, could tweaks be made to it to make it a truly unconditional framing of the problem?--Father Goose (talk) 05:55, 17 March 2009 (UTC)

The diagram has the same basic issue as vos Savant's solution published in the December 1990 Parade column, which is that the problem says to consider the case where the player has picked door 1 and the host has opened door 3 (using these as representative of the situation where the player is deciding to switch after picking one of the doors and the host has opened another one) but the solution considers other cases. In the diagram the player has picked door 1 only in case 1 (so cases 2 and 3 simply don't apply - at least not to the problem as stated) and the host opens door 2 sometimes in case 1 and all the time in case 3 (so even case 1 doesn't always apply and again case 3 never applies). We could presumably restate the problem somehow to make the diagram fit (putting us in WP:OR territory), perhaps:

You'll pick one of these three doors and after you've made your choice I'll open a different door revealing a goat. You can choose now whether your "final" door will be your original pick or the other door that remains unopened after I've opened a door to show a goat. Now, what door would you like and would you like it to be your final door or would you prefer to switch to the other door that's left after I open a door?

Let me ask you something: doesn't the diagram currently in the article clearly show the exact problem that's asked? The player's pick is always door 1 (but the car can be behind any door), and the two cases (host opens door 2 vs. host opens door 3) are clearly grouped. In the problem as asked, the host opens door 3 putting us in the right half of the picture where switching wins twice as often as staying. -- Rick Block (talk) 14:09, 17 March 2009 (UTC)

Accept for a second that the unconditional diagram is not a depiction of the conditionally stated problem. Pretend for a second that you've never heard of the Parade version of the problem (or any other conditionally phrased version), and that you're trying to create a diagram that depicts a purely unconditional form of the problem. Does this diagram successfully do that? If not, what changes would be needed to make it represent an unconditional form of the problem?--Father Goose (talk) 21:10, 18 March 2009 (UTC)

Rick, checking back, I see you're been shepherding this article for many years, since before it made "featured" in 2005. But at that time, it didn't have any of the current problem, of overloading the commonly-published simple solution with all the conditional stuff, and all the strife, confusion, and bloat that has come from that. A solution to the problem was proposed above. Surely you have enough good sources, including the Granberg paper with its list of conditions to make the initial 1/3 probability not change when a goat is revealed, to fix the problem as suggested. If you don't want to use this source on its own, because some idiot editor converted 2/3 to .67, combine it with some others that don't make that mistake. But don't us this stuff about him not being a mathematician; it doesn't take a professional mathematician to analyze a problem in probability, does it? Dicklyon (talk) 15:14, 17 March 2009 (UTC)

Yes, I originally nominated it as a featured article and responded to the criticisms from the FAC review, and the two FARs it's been through as well. My opinion is almost exactly the opposite of Father Goose's - specifically that it has dramatically improved over the last several years (it was originally woefully under-referenced and presented a very shaky unconditional argument as its main "solution"). The inclusion of a more mathematically rigorous solution was largely due to the efforts of an anonymous editor, shortly before the most recent FAR with highly voluminous discussions on the talk page (starting at Talk:Monty Hall problem/Archive 6#Rigorous solution). This editor was the first to mention the Morgan et al. reference - which I believe is the first mathematically rigorous treatment of the MHP published in a peer reviewed math journal. Since this rigorous treatment has already been published, there in all likelihood will never be another (Gillman is sort of an oddity - my assumption is Gillman's paper was already "in press" when the Morgan et al. paper actually appeared). There is certainly no shortage of popular sources about this problem, but the Morgan et al. paper is (more or less) the definitive reference.

You ask whether it takes a professional mathematician to analyze a problem in probability. Of course, in general, no. On the other hand, this problem generates massive amounts of controversy, IMO (well, actually backed up by lots of psychology sources like Granberg) because it deals with conditional probabilities which are not very well understood by most people - but it is a very simple problem on the surface which leads many people to think they understand it when they actually don't. This problem generates so much controversy that "serious mathematicians" at this point pretty much refuse to talk about it since they understand the rat-hole it becomes. The "many people" who think they understand it but actually don't includes lots of people who write about it as well as lots of academics (as evidenced by the large numbers of academics who wrote to vos Savant telling her she was wrong). The point is not that it would take a professional mathematician to produce a sound unconditional solution for this problem, but that it is harder than it seems and just because something happens to be published about this problem does not mean it is mathematically sound.

I definitely understand the request being made here - for an easily understandable (which probably means unconditional), convincing, and (I'll add) mathematically sound solution. Unfortunately, I am not aware of a published solution that meets this criteria. -- Rick Block (talk) 19:40, 17 March 2009 (UTC)

Well, it's great that they got you to come around to the "rigorous" conditional solution. But I think think the Granberg reference is plenty adequate for the "usual" solution, irrespective of whether you think it's "rigorous". And you probably have others that present the usual solution along with some words to the effect that it's applicable when the host's action doesn't provide any extra information about the probability of the originally chosen door having a car. Just go with it. Leaving it the way it is is too higher-math POV. Dicklyon (talk) 22:06, 17 March 2009 (UTC)

Here is another in which it is at least stated that the contestant "learns nothing new" to justify the usual solution; in this type of solution, the different between deciding ahead of time to switch and deciding after a door is opened is moot, based on the assumptions, so the actions you condition on are irrelevant and can be ignored. Oh, and by the way, it's the overwhelmingly most common analysis, and nobody disputes that it gives the right answer (that you should switch) and the right probability of winning (2/3), even if one did decimalize it poorly. Dicklyon (talk) 22:12, 17 March 2009 (UTC)

I added a section break and a few words as step toward the proposed resolution. Feel free to work on it to make it better sourced or more precise. Dicklyon (talk) 22:53, 17 March 2009 (UTC)

It (or some other unconditional solution) is certainly the most common analysis and nobody disputes the "you should switch" answer, but plenty of folks note the actual probability of winning by switching (without the "equal goat constraint") is not 2/3 but something in the interval [0.5, 1]. vos Savant has even written about the "host forgets" variant [2] and says:

Back in 1990, everyone was convinced that it didn’t help to switch, whether the host opened a losing door on purpose or not. Assuming a knowledgeable host who would always open a losing door, that was incorrect. (A knowledgeable host who opened a winning door on purpose wouldn’t have much of a show, would he?!)

Now everyone is convinced that it always helps to switch, regardless of what the host knows. But this is just as incorrect!

Here's some of my own WP:OR - the reason "everyone" now says it always helps to switch is because many sources (like, ahem, vos Savant - whose own book ironically enough is titled "The Power of Logical Thinking") have presented an unconditional solution for the original problem as the (or at least "a") correct solution. Whether it's the standard version or the host forgets version or the host always picks the leftmost door version, it's always a conditional probability problem. Using an unconditional solution without understanding exactly what you're doing is dangerous, and (more of my own WP:OR) most people who present an unconditional solution don't really know what they're talking about.

We've tried to straddle this line before (e.g. this version). I have no objection to this in principle, so long as we're very clear about when and why an unconditional solution is valid. I don't like "Solution" and "Conditional solution" as equal level headers, perhaps Solution with subheaders for "Popular solution" and "Conditional solution". This has been discussed before, with no one on either the "unconditional" or "conditional" side willing to accept a section heading that implied anything other than "this is the solution" (which is why both have been in the same "Solution" section). -- Rick Block (talk) 02:02, 18 March 2009 (UTC)

We should just ignore anyone who comes here to promote the idea that there is only one Right way to argue for the solution, whatever his or her preferred way is. That premise is contrary to the idea of an encyclopedia, and contrary to how things are solved in mathematics in general.

Also, I don't think your headings are good -- "popular solution" appears to imply that there is something wrong with the solution (which the hoi polloi cannot appreciate); "conditional solution" sounds as if the solution itself is only valid on certain conditions.

I suggest we drop the assumption that the headings should be noun phrases that describe the solution. Let them refer to a key idea used in the argument instead. Perhaps "Anticipated choice" and "Conditional probabilities" would do. –Henning Makholm (talk) 12:52, 18 March 2009 (UTC)

I'll give that a try, and see who squacks. Dicklyon (talk) 02:23, 18 March 2009 (UTC)

Slow down, guys!

This talk page is moving too fast for anybody but the most zealous editors to follow the debate. I suspect this in itself makes it difficult for a good consensus to form. How about we

Archive the old stuff already.
Put everybody on a "two talkpage comments per day" restriction.

That would give more people than the two or three protagonists a fighting chance to contribute, at the possible expense of arriving at a conclusion a little slower. But remember, there is no deadline! –Henning Makholm (talk) 13:04, 18 March 2009 (UTC)

I'll slow down; thanks for your willingness to participate. Dicklyon (talk) 22:22, 18 March 2009 (UTC)

General criticisms of the article

At this time I have two criticisms of the article. One is that the diagram in the "popular solution" section depicts the "conditional solution" and thus is in the wrong section. Another is that if the "unconditional solution" is an invalid way to analyze the problem in general, then the simulation approach, as described, is invalid as well.--Father Goose (talk) 22:04, 18 March 2009 (UTC)

Right, why don't you go back to that older version that did a better job on the usual solution, and substitute its image and change words as needed? I was just checking to see if the basic structure got any objections first. And on the simulation approach, it might be good to mention the conditions under which the simulation addresses the problem, hopefully from sources about the simulations. Dicklyon (talk) 22:21, 18 March 2009 (UTC)

I strongly object to reverting to the earlier image since the current image has several advantages. In particular, the current image makes the conditional solution accessible, it matches the problem as described (player picks door 1 and host opens door 3) and its formatting is carefully arranged to convey additional meaning (the column widths reflect the relative probabilities of the items in the column and the "host opens door 3" vs. "host open door 2" cases are adjacent). Showing both images would be possible, but they're so close that this would be redundant. -- Rick Block (talk) 00:42, 19 March 2009 (UTC)

Rick, I thought you had agreed in principle to represent the usual simple solution simply, without reference to complication by the conditional appproach. That figure does that, but it sounds like that's why you object now. I think you also need to reconsider your interpretation of what the problem says. It doesn't really say the host opens door 3, it merely uses that as an illustrative example, where it says "say No. 3", or at least that's the way many of us read it. So why complicate this section by trying to get it to anticipate the more complex interpretation? Dicklyon (talk) 03:55, 20 March 2009 (UTC)

We can present an unconditional solution that doesn't conflict with a conditional solution - choosing to do otherwise seems perverse. Goose's original diagram (which, BTW, the current one descends from) keeps the arrangement of car and goats constant and varies the door the player selects. The current version flips this (consistent with the problem statement) making the placement of the car the dominant variable, rather than the player's pick. Either way works just fine for an unconditional solution and either way is a simplification (there are actually 9 possibilities of car placement and player pick). We want to show a simplification with 3 possibilities to resonate with the 1/3 chance of picking the car. The current diagram doesn't require the goats to be distinguishable and even works with empty doors rather than goats as well.

I agree the "say No. 3" language is meant as an illustrative example and make no claim that the only case to be considered is when the host opens door 3. If you're interpreting anything I've said to mean this you're not understanding what I'm saying. What I am saying is that the question pertains to the probabilities involved after a player has picked a door (any door, not just door 1) and the host has opened some other door (not just door 3). llustratively, we call these "door 1" and "door 3", but we could just as well call them "the door the player initially picked" and "the door the host opened". Whatever we call these doors, "my interpretation" (this is really the interpretation of Morgan et al., and Gillman, and Grinstead and Snell - and pretty much any mathematician who reads the problem) is that we're talking about a conditional probability (not the "average" across all players, but the probabilities for a player initially selecting some specific door when the host has opened some other specific door in response). If we analyze the specific case of "door 1/door 3", we can generalize the answer for "door i/door j". If this answer is the same for all i and j, it must also be the same as the unconditional solution (but not vice versa). -- Rick Block (talk) 04:55, 20 March 2009 (UTC)

No, I get what you mean, but I think the way the example was presented in the problem statement was also strongly suggestive of the situation wherein things are symmetric enough that you don't need to care which door is opened or how they're numbered or whether there might be any door-number-dependent aspect at all intended. Let's see what kind of diagrams are used in publications on the popular solution; maybe that will help guide us. Dicklyon (talk) 05:05, 20 March 2009 (UTC)

If I may ask, what is your response to the two criticisms I made?--Father Goose (talk) 02:18, 19 March 2009 (UTC)

Although I agree the current split into Popular and Conditional sections is kind of a mess (I don't mean this in a derogatory kind of way, it's pretty clearly simply a work in progress) I think the existing diagram can serve both purposes. Specifically, all but the very last line apply to both the conditional and unconditional analysis - and, ignoring the last line, you have switching wins with probability 1/3+1/3 and switching loses with probability 1/6+1/6.

The simulation section explicitly says "a switching strategy really does win two out of three times on the average" (emphasis added). We could certainly make this more clear, and probably should. The "Combining doors" section has a similar issue, and a similar disclaimer. A long, long time ago, the "Combining doors" section was part of the main "Solution" section (it is another commonly presented "popular" solution). How about illustrating the popular solution section with the figure from this section? (this is my second edit to the talk page today, so I guess I'm done until tomorrow - I agree a rate limit here might help control the volume, and I think the /Arguments page is helping as well) -- Rick Block (talk) 03:08, 19 March 2009 (UTC)

I would just like to point out that there are at least 2 separate discussions taking place regarding this article. And I understand there is a 3rd page as well. If our goal is to reach consensus on editing the Article, I think we would benefit from all editors being in 1 discussion. Yes? Glkanter (talk) 15:12, 19 March 2009 (UTC)

Links to them please? If you mean the Arguments page, you'll have to get more specific, as I can't find much there about how to evolve the article. I don't need the math tutorial, as I understand the conditional solution just fine, unlike some people who don't seem to get it. But it's not the solution that most sources present, and it's not neutral to treat it is the only acceptable solution. These math arguments aren't going to help us get to a better article, I think. Dicklyon (talk) 04:04, 20 March 2009 (UTC)

I'm not sure, but I think Glkanter means here, and at /Arguments, and at /Analysis (a page user:Martin Hogbin has created with his own WP:OR analysis). -- Rick Block (talk) 04:55, 20 March 2009 (UTC)

Criticism and a new approach

My general criticism of the article is that it is much too long. A specific criticism of the article is that the Bayesian analysis is cumbersome and restrictive. Why should the quiz-master use equal probabilities for the two doors to open, when he has a choice? I like to see the problem as a game in which the quiz-team (including the quiz-master) has various possible deterministic strategies, and in general will use one of the possible deterministic strategies at random, according to some probability distribution. At the same time, the player has various possible deterministic strategies, and in general will use one of these strategies at random, according to some probability distribution. Here is a complete list:

Quiz-team's 6 possible deterministic (non-random) strategies:

Car behind door 1 and QM will open door 2 when player chooses door 1

Car behind door 1 and QM will open door 3 when player chooses door 1

Car behind door 2 and QM will open door 1 when player chooses door 2

Car behind door 2 and QM will open door 3 when player chooses door 2

Car behind door 3 and QM will open door 1 when player chooses door 3

Car behind door 3 and QM will open door 2 when player chooses door 3

The player's 12 possible deterministic strategies:

Choose door 1 and switch if QM opens 2, switch if QM opens 3

Choose door 1 and switch if QM opens 2, don't switch if QM opens 3

Choose door 1 and don't switch if QM opens 2, switch if QM opens 3

Choose door 1 and don't switch if QM opens 2, don't switch if QM opens 3

Choose door 2 and switch if QM opens 1, switch if QM opens 3

Choose door 2 and switch if QM opens 1, don't switch if QM opens 3

Choose door 2 and don't switch if QM opens 1, switch if QM opens 3

Choose door 2 and don't switch if QM opens 1, don't switch if QM opens 3

Choose door 3 and switch if QM opens 1, switch if QM opens 2

Choose door 3 and switch if QM opens 1, don't switch if QM opens 2

Choose door 3 and don't switch if QM opens 1, switch if QM opens 2

Choose door 3 and don't switch if QM opens 1, don't switch if QM opens 2

This is a zero-sum game and it has a saddle-point, or equilibrium, when we allow randomized strategies, ie each of the two sides chooses from their respective lists at random, according to two lists of probabilities. The quiz-team's minimax strategy is to choose between each of their 6 fixed strategies with equal probabilities. Ie the location of the car is uniform at random (equal probabilities 1/3), and the quizmaster makes his choice of door to open by a fair coin toss, if he has a choice. Then the player cannot win with probability greater than 2/3. The player's minimax strategy is to choose his door initially uniformly at random and then to always switch. Thus he puts equal probabilities 1/3 on the 3 deterministic strategies in which he always switches, and never uses the other 9. This way the player is guaranteed to win the car with probability 2/3, whatever strategy is used by the quiz-team. Whether or not he wins the car with probability 2/3 given his own initial choice, and if you like, also given the choice of the quiz-master, depends on the quiz-team's strategy. For some conditions it can be above 2/3, for others below; the only guarantee we have is that it averages out at exactly 2/3.

I feel that the Bayesian analysis section therefore gives misleading information. The conditional probability, given your initial and given the quiz-master's choice, that the car lies behind "the other" closed door, is only 2/3 when the quiz-master's and indeed the whole quiz-team's behaviour has been unreasonably limited. In order for the player to win 2/3 of the time it is sufficient that the player makes a true random choice of door, and thereafter always switches. Whether or not the player is going to win with conditional probability 2/3 given his initial choice (and also given the quiz-master's choice, if you like), depends on the quiz-team's strategy. Which, we suppose, the player doesn't know.

See http://www.math.leidenuniv.nl/~gill/threedoors.pdf

I shall try to get this paper into a peer-reviewed journal.

Gill110951 (talk) 22:14, 22 March 2009 (UTC)

@Gill: With your analysis as a zero-sum game, you're addressing a different problem than the stated MHP. You are analyzing the problem as if the player leaves home and after closing his front door decides what to do in all possible circumstances. But the MHP puts the player on stage, whith a door chosen (that's why it says: say No. 1) and seeing a goat behind another door (say No. 3). 82.75.140.46 (talk) 17:10, 23 March 2009 (UTC)

You're right, in a sense. But what difference does it make? The player might just as well imagine what he will do if does first choose door 1 and then is shown a goat behind door 3. He can imagine in advance all possibilities. Or: we can imagine that he imagines in advance, all possibilities. What the game-theoretic analysis makes explicit is that there is no way he gets any guarantee by choosing a non-random strategy (ie deciding in advance certainly to choose door 1, etc). There is only a unique conditional solution to the problem, if we restrict the actions of the quizmaster. What we also learn is that the only way the quizmaster can guarantee that the player doesn't win with a probability larger than 2/3 (unconditional) is to put the car behind a uniform random door, and to open a uniform random door when he has a choice. At the same time, the only way the player can guarantee himself to win with probability at least 2/3 is to choose his door uniform at random and thereafter always to switch doors. In the situation that the quizmaster plays the minimax strategy it is true that the conditional probability of the car being behind the other door is 2/3. But how do we know he uses the minimax strategy? I don't see it stated in the problem. So strictly speaking there is no answer to the question "what is the probability the car is behind the other door, given the player chose door 1 [even if he chose it uniformly at random] and the quizmaster opened door 3".

By the way, I am not editing the page itself at this stage, just throwing a new titbit into the arena, for anyone who is interested. Since it is original new research - of the day before yesterday - I am not even allowed to put it on the page. Gill110951 (talk) 10:45, 24 March 2009 (UTC)

Thanks you for your contribution, I think it confirms what I and other have been saying for some time which is that the Morgan paper, although not exactly wrong, answers a question than nobody would ever ask. Martin Hogbin (talk) 22:31, 26 March 2009 (UTC)

In the fully explicit version stated in the article, which seems to match most people's expectations for how the problem should be defined, the host is constrained to open a door randomly if given a choice. This behavior is not specified in the Parade version, leaving open the possibility for other host behaviors. The Morgan et al. paper analyzes the Parade version and expresses this host behavior as a variable q (where q=1/2 means the host is constrained to pick randomly) and shows the player's chance of winning is 1/(1+q). With q=1/2 (which is not stated, but is perhaps the "intended" interpretation) the answer is 2/3, but since q can range from 0-1, the chance of winning by switching without this constraint varies between 1/2 and 1. Rather than confirming they've answered a question nobody would ever ask, I think this thread is confirming the exact opposite. -- Rick Block (talk) 00:12, 27 March 2009 (UTC)

Rick, I think most people agree that Morgan et al.'s interpretation is a bit far fetched, and also it's still the case that averaged over the possible configurations the prob of winning by switching remains 2/3. Since there's nothing in the problem statement to suggest that door 3 was anything but an example, or that the answer could depend on what door the host opened, it takes a bit of imagination to formalate a problem in which it matters and in which the example given is taken to define the question being asked. Sure, it's a perfectly fine precise mathematical interpretation, with the added caveat that the host's choice can be modeled as a stochastic event, and Morgan et al. give a great solution of that problem. But reasonable balance and NPOV would suggest that we put the most weight on the mainstream interpretation of the problem. Right? Dicklyon (talk) 02:00, 27 March 2009 (UTC)

I don't understand your point here. Are you saying the "mainstream interpretation" is that it's an unconditional problem? If the point of the problem is to evaluate the probabilities when the player is in front of two closed doors and one open door (which I think is the standard interpretation), then it's a conditional problem. The natural symmetry of the situation makes the conditional probability of interest the same as the unconditional probability, but without an explicit constraint that the host pick randomly if the player initially picks the car the problem is not necessarily symmetric. This is the main point of the Morgan et al. paper (and Gillman's paper, and numerous other references). It's a quite reasonable assumption that the host has no preference if the player's initial selection is the car and this makes the answer 2/3, but even explicitly stating this constraint doesn't turn it into an unconditional problem. Is there a competing POV here? -- Rick Block (talk) 04:52, 27 March 2009 (UTC)

I'm not saying the mainstream interpretation is that it's an unconditional problem; rather, that in the mainstream interpretation that distinction doesn't come up, and so what you're calling the unconditional solution is generally considered to be the solution. Furthermore, the mainstream interpretation includes the symmetry condition when people go further and try to make it more mathematically rigorous by saying that the host's choices doesn't affect the 1/3 probability of the original choice still being the car after a door is opened. I quite understand the Morgan solution, and that he teaches that all other solutions are inadequate, but that's certainly not the mainstream view. Is it? Dicklyon (talk) 05:07, 27 March 2009 (UTC)

It depends on what you mean by "mainstream interpretation". I believe the mainstream interpretation in published probability theory articles (Morgan et al., Gillman - there are more) and books (Grinstead and Snell for one) is that the problem is a conditional probability problem and that the host's choice when the player initially picks the car therefore matters. Likely because of Morgan et al. and Gillman, the "standard analysis" now includes the condition that the host pick randomly in this case - forcing the answer to unambiguously be 2/3 chance of winning by switching. On the other hand, the popular sources (starting with vos Savant) generally treat the problem as if the question were "what is the chance of winning if the player's predetermined strategy is to switch" (which is an unconditional problem). I don't think this is a POV issue - it's simply a case of the popular sources (led by vos Savant) choosing to answer a simpler question (without saying so - leaving the question open of whether they understand what they're talking about or not). Many of the solutions that claim the host's choice doesn't affect the player's initial 1/3 probability (because the host must open a door and must show a goat - without saying anything about the host's choice if the player initially picks the car) confuse unconditional and conditional probabilities and are arguably simply wrong (Falk is a good reference for this).

Rather than continuing to argue about this, how about following an approach similar to the one used in Grinstead and Snell? They start with the sticker (wins 1/3) vs. switcher (wins 2/3) player strategy, but then go on to explain that this doesn't actually address the conditional probability question that the problem asks and show a conditional solution. -- Rick Block (talk) 00:03, 28 March 2009 (UTC)

Yes, I think that's the strategy I had been aiming for, too. Present the usual simple solution, and then after present the "modern" analysis that says it doesn't really address the problem as they interpret it, and present their new solution. Dicklyon (talk) 03:58, 28 March 2009 (UTC)

That's exactly what I'd like to see as well. We could, in the process, tie it closely to the "simulation" proof, since the usual form of the simulation is multiple trials of the unconditional problem. Using the unconditional problem to prove the solution (via a simulation) apparently had cachet with the originator of the problem (Gardner), so that's got to lend it some weight.--Father Goose (talk) 08:44, 28 March 2009 (UTC)

Ok to me, be it that I would call the "simple solution" a "simple way of understanding" rather than a solution. And as Father Goose already mentioned, the simulation approach is mainly (always) the unconditional one, and the article should mention this shortcoming. Nijdam (talk) 10:07, 28 March 2009 (UTC)

If you don't call it a "solution", you're inflicting a POV about what the problem is, which conflicts with the usual interpretation. And as the simulation, simulating an "always switch" strategy versus and "always stick" strategy doesn't really embody a conditional or unconditional probability model, does it? How is it a shortcoming? Dicklyon (talk) 14:51, 28 March 2009 (UTC)

The usual simulation does not address the conditional probability the problem seemingly asks for. A proper simulation would keep track of switch/stay success per combination of initial player pick and door the host opens (6 combinations). Lumping these all together means the simulation answers the question "what is the chance of winning by switching if deciding to switch before the host opens a door". How about saying something like "Most popular sources assume that the question intends the probability of winning by switching to be the same whether the player decides to switch before or after the host opens a door, and analyze the before case." I believe this is the truth - but finding a reliable source that puts it this way might be challenging. -- Rick Block (talk) 16:20, 28 March 2009 (UTC)

See, for example, /Arguments#Excel simulation of difference between "random goat" and "leftmost goat" variants. Note the effect the host strategy can have on the probability of winning by switching when given knowledge of which door the host opens. -- Rick Block (talk) 16:27, 28 March 2009 (UTC)

Yes, we get that. But in the usual situation where the contestant doesn't have a probabilistic model of the host's door choice behavior, it's really quite an irrelevant distinction to the contestant or to the problem as usually understood. Dicklyon (talk) 16:51, 28 March 2009 (UTC)

(outindent) Dick - you say "we get that". What "we" are you talking about? The question asks about a conditional probability (you agree with this don't you?). Without making it clear what they're doing, most popular sources present a solution based on an analysis of unconditional probabilities. For the conditional and unconditional probabilities to be the same, the host must pick randomly between two goats in the case the player initially picks the car. One might say the popular sources know what they're doing and are simply assuming this to be the case - others might say the popular sources are misunderstanding the problem and are, without realizing it, solving a different problem (one where the player must decide whether to switch before the host opens a door). Morgan et al. and Gillman (and many others) say the latter. You're saying it's POV for Wikipedia to say the popular sources are misunderstanding the problem (right?). Given the number of reliable sources that say exactly this, isn't it also POV to avoid saying it? I think this may perhaps be a case involving a prevalent popular misconception about something where the "experts" say the truth is something different than what is popularly believed to be true. Off hand, I can't think of other examples (surely there must be some). If anyone can think of others it might be interesting to see how they're handled. -- Rick Block (talk) 02:48, 29 March 2009 (UTC)

By "we" I mean myself and any other intelligent discussants here; if someone doesn't get it by now, why bother talking to them? I understand what you're saying, especially that "Without making it clear what they're doing, most popular sources present a solution based on an analysis of unconditional probabilities." I don't think it's necessary to agree with you on "The question asks about a conditional probability (you agree with this don't you?)" since that's not the interpretation of most popular sources, as you note. As to whether the popular sources are "misunderstanding" or not, I don't think we have to know the answer to that. Morgan et al. and Gillman take a position on that, which we can report, but which we don't need to endorse. You can report as much as you like that top statisticians say the popular solution is wrong. But first you need to present the popular solution; then, move on to those who say it's wrong. Don't pollute the presentation of popular solution with the modern revised solution; first the one, then the other with an explanation of why they say the one is inadequate. That's the NPOV way to go about it. Dicklyon (talk) 03:01, 29 March 2009 (UTC)

On second thoughts: why should the popular wrong "solution" be presented first? Beter to give an easy to understand right solution first! Nijdam (talk) 16:24, 29 March 2009 (UTC)

Interesting idea. You could start with a listing of all the elements that make the conditional solution 'required':

It only applies when the contestant chooses door 1, the host reveals door 3, and the car is behind door 1

Without the 'equal goat door' constraint it has no numeric solution

There is some sort of 'host behaviour', but no one's really sure what it might be, or whether the contestant knows about it

Whatever else you guys consider important

Then you could refer the reader to the unconditional solution in the next section, which is the one he wanted all along. Glkanter (talk) 15:11, 30 March 2009 (UTC)

(Counter)example

Maybe the next example is helpful to understand the problems with the problem.

I throw a fair dice. What is the probability of my outcome?

Some people may reason: the outcome is one of the numbers 1 ... 6, so the probability that "the outcome is one of the numbers 1 ... 6" is 1. And they are right in some sense.
The common meaning of the question is of course: for each possible outcome the probability is 1/6.
Imagine you are standing next to me and you see the outcome of my throw. You definitely will say: 1/6.

Nijdam (talk) 09:29, 27 March 2009 (UTC)

It's not clear what this is an example of. Dicklyon (talk) 15:45, 27 March 2009 (UTC)

No? Imagine yourself standing next to the player. What would you base your decision on?Nijdam (talk) 16:25, 29 March 2009 (UTC)

Please explain yourself instead. What is this an example of? How does it relate to the article? –Henning Makholm (talk) 17:00, 29 March 2009 (UTC)

Replace the Popular Solution Section With the 'Combining Doors' Solution

I propose the article be changed in the following way:

Replace the Popular Solution Section with the 'Combining Doors' Solution. Further, I would remove any statements regarding how the host chooses between the two doors. (Only 1 of the four sources in this section mention any 'host behaviour'). I would also add some formal notation of the diagrams, that would convey this:

Once the contestant has selected a door: 1/3 + (1/3 + 1/3) = 1 where the 1/3 on the left of the '+' sign represents the chosen door, and the numbers inside the parenthesis represent the remaining doors.

After a goat is revealed, the formula appears as: 1/3 + (2/3 + 0) = 1 or 1/3 +(0 + 2/3) = 1

As the only two possible outcomes are equivalent, regardless of which door the host reveals, this can be stated as: 1/3 + 2/3 = 1

Therefore switching, once it is offered by the host, will win the car 2x as often as not switching.

Glkanter (talk) 16:50, 27 March 2009 (UTC)

In the light of the ongoing discussion this seemes highly premature, not to say complete without any sense of reality.Nijdam (talk) 17:25, 27 March 2009 (UTC)

Perhaps you could respond in the manner described below:

Please treat this the same way you would an article nominated at WP:FAC. There, each objection must provide a specific rationale that can be addressed. Do you have any specific suggestions for how to improve the proposal above? -- Rick Block (talk) 18:19, 29 March 2009 (UTC)

Thank you. Glkanter (talk) 15:35, 30 March 2009 (UTC)

A concrete proposal

Given the voluminous discussions above, and in the archives of this page and at the #Arguments page, how about the following? Please comment below. -- Rick Block (talk) 17:18, 29 March 2009 (UTC)

Solution

There are two main approaches to solving the Monty Hall problem.

Evaluating switching versus staying

The probability of winning for all players who switch or all players who don't switch can be determined by examining all possible outcomes in either case (Grinstead and Snell 2006:137). For example, the figure below shows the outcomes when switching if the car is randomly placed behind one of the doors and the player initially picks Door 1. Switching will win if the car is behind either Door 2 or Door 3 with probability 2/3 while switching will lose only if the car is behind Door 1 with probability 1/3. Not switching wins if the car is behind Door 1 with probability 1/3, and otherwise loses with probability 2/3. The situation is similar no matter which door the player initially picks, so players who switch win twice as often as players who don't.

Car hidden behind Door 1	Car hidden behind Door 2	Car hidden behind Door 3
Player initially picks Door 1

Host opens either goat door	Host must open Door 3	Host must open Door 2

Switching loses with probability 1/3	Switching wins with probability 2/3

Evaluating the conditional probability

The solution above shows that the probability of winning by switching for all players who switch is 2/3, but this does not necessarily mean the probability of winning by switching is 2/3 given which door the host opens. This probability is a conditional probability (Morgan et al. 1991; Gillman 1992; Grinstead and Snell 2006:137). The difference is whether the analysis, as above, considers all possible scenarios or only the scenarios where the host opens a specific door. Another way to express the difference is whether the player must decide to switch before the host opens a door or is allowed to decide after seeing which door the host opens (Gillman 1992). The conditional probability may differ from the overall probability depending on the exact formulation of the problem.

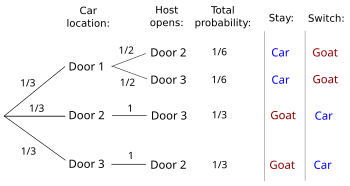
The conditional probability of winning by switching given which door the host opens can be determined referring to the expanded figure below or to an equivalent decision tree as shown to the right (Chun 1991; Grinstead and Snell 2006:137-138). For example, if the host opens Door 3 and the player switches, the player wins with overall probability 1/3 if the car is behind Door 2 and loses with overall probability 1/6 if the car is behind Door 1—the possibilities involving the host opening Door 2 do not apply. To convert these to conditional probabilities they are divided by their sum, so the conditional probability of winning by switching given the player picks Door 1 and the host opens Door 3 is (1/3)/(1/3 + 1/6), which is 2/3. This analysis depends on the constraint in the explicit problem statement that the host choose which door to open randomly if the player has initially selected the car.

Morgan et al. (1991) and Gillman (1992) both show a more general solution where the host is not constrained to pick randomly if the player has initially selected the car, which is how they both interpret the well known statement of the problem in Parade. They consider a scenario where the host chooses which door to open in this case with a preference expressed as a probability q, having a value between 0 and 1. If the host picks randomly q would be 1/2 and switching wins with probability 2/3 regardless of which door the host opens. If the player picks Door 1 and the host's preference for Door 3 is q, then in the case where the host opens Door 3 switching wins with overall probability 1/3 if the car is behind Door 2 and loses with overall probability (1/3)q if the car is behind Door 1. The conditional probability of winning by switching given the host opens Door 3 is therefore (1/3)/(1/3 + (1/3)q) which simplifies to 1/(1+q). Since q can vary between 0 and 1 this conditional probability can vary between 1/2 and 1. This means even without constraining the host to pick randomly if the player initially selects the car, the player is never worse off switching.

Car hidden behind Door 3	Car hidden behind Door 1		Car hidden behind Door 2
Player initially picks Door 1

Host must open Door 2	Host randomly opens either goat door		Host must open Door 3

Probability 1/3	Probability 1/6	Probability 1/6	Probability 1/3
Switching wins	Switching loses	Switching loses	Switching wins
If the host has opened Door 2, switching wins twice as often as staying		If the host has opened Door 3, switching wins twice as often as staying

References

Gillman, Leonard (1992). "The Car and the Goats," American Mathematical Monthly 99: 3–7.
Grinstead, Charles M. and Snell, J. Laurie (2006-07-04). Grinstead and Snell’s Introduction to Probability (PDF). Online version of Introduction to Probability, 2nd edition, published by the American Mathematical Society, Copyright (C) 2003 Charles M. Grinstead and J. Laurie Snell. Retrieved 2008-04-02. {{cite book}}: Check date values in: |date= (help)CS1 maint: multiple names: authors list (link)
Morgan, J. P., Chaganty, N. R., Dahiya, R. C., & Doviak, M. J. (1991). "Let's make a deal: The player's dilemma," American Statistician 45: 284-287.

Comments on the above proposal

I think Nijdam said it best. "In the light of the ongoing discussion this seemes highly premature, not to say complete without any sense of reality." Glkanter (talk) 17:34, 29 March 2009 (UTC)

Please treat this the same way you would an article nominated at WP:FAC. There, each objection must provide a specific rationale that can be addressed. Do you have any specific suggestions for how to improve the proposal above? -- Rick Block (talk) 18:19, 29 March 2009 (UTC)

I say hay okay, or something equivalent. Nijdam (talk) 20:08, 29 March 2009 (UTC)

What the article needs is a simpler, clearer, and more convincing explanation of the more general 'conditional' problem, which is equivalent to the unconditional problem. By this I mean that the Morgan argument applies only to the specific case where the host has opened door 3 as is made clear above. It is far from clear that this what what the original questioner intended to ask. In the more general case, where the host has opened either door 2 or door 3 the probability is 2/3. As I have said before, opening either door 2 or door 3 is essentially a null condition in that it has no effect whatsoever on the probability of winning by switching, thus the conditional and unconditional answers and solutions are both the same. The thing that makes this problem notable is that, even when unambiguously described in a form where the unconditional answer is valid, most people still get it wrong. Martin Hogbin (talk) 21:22, 29 March 2009 (UTC)

Yep, this whole wild goose chase is about how the host decides, in the specific case of the contestant choosing the car, which LOSING door to show. However he decides, there's never been any indication that he shares this algorithm with the contestant. That would kind of violate the 'host vs contestant' roles, wouldn't it? The 'equal goat door' constraint is meaningless and therefore, worthless (as in 'it adds no value'). Glkanter (talk) 00:10, 30 March 2009 (UTC)

In case it's not obvious, I'm suggesting three things here:

Changing the outline a bit by introducing a level 2 "Solution" header and changing the existing level 2 headings "Popular solution" and "Conditional solution" to level 3 headings "Is always switching better?" and "Is switching always better?"
Replacing the content currently in the "Popular solution" section with the content above under "Is always switching better?"
Replacing the content currently in the "Conditional solution" section with the content above under "Is switching always better?"

Martin and Glkanter - are you objecting to the proposed heading structure, or the proposed content under "Is always switching better?" or both? The intent of the "Is always switching better?" section is to convey the 1/3 + 2/3 = 1 style of solution, simply and convincingly. If you think this could be expressed better please suggest improvements. Neither of you apparently have any comprehension whatsoever of the "Is switching always better?" section, so other than suggesting some wording for prerequisites assumed for this section (per Wikipedia:Make technical articles accessible) perhaps it's best if you simply don't comment on this section. -- Rick Block (talk) 15:35, 30 March 2009 (UTC)

Your proposal is promising, Rick -- it does a good job of explaining the differences between the unconditional and conditional solutions, and why a number sources suggest that they are not both valid analyses of "the question as asked".

Somewhat off-topic, what I'd really like to see -- and this may be wishful thinking -- is an analysis of why both the unconditional and conditional scenarios (with the extra stipulation of "host's random pick) produce the same odds: 2/3ds when switching. Is it entirely coincidental?--Father Goose (talk) 22:21, 30 March 2009 (UTC)

Assuming this wasn't just a rhetorical question (and this is probably more appropriate for the #Arguments page), of course it's not coincidental. The random host pick constraint forces the conditional probability to be the same regardless of which door the host opens. The unconditional probability is sort of like the "average" probability - if all conditional probabilities are the same the unconditional probability has to be the same as the conditional probabilities. This would (well, should) be covered in any undergraduate (perhaps even high school) level probability class. -- Rick Block (talk) 00:35, 31 March 2009 (UTC)

Rick, I see several problems with the first part; first, I think it complicates the issue and misrepresents the source when it says "for all players who switch or all players who don't switch;" there's nothing in Grinstead and Snell 2006:137 about "all players." The source says "if a contestant plays the “switch” strategy, then he will win whenever the door he originally picked does not have the car behind it, which happens 2/3 of the time." This applies to any individual player; saying "all players" makes it sound like you're averaging or requiring an emsemble, or somehow not answering the question for a player. It goes on to say "This very simple analysis, though correct, does not quite solve the problem that Craig posed. Craig asked for the conditional probability that you win if you switch, given that you have chosen door 1 and that Monty has chosen door 3." Yet, that is clearly just an interpretation, since Craig's problem statement doesn't say anything about conditional probabilities, and makes it clear that the door numbers are illustrative, not a special problem that needs an answer different from other illustrative numberings. It's fine to present this interpretation, but to present it as a part of the first solution, when it's really a criticism of that solution, is not a neutral approach. Your solution statement continues to focus on door numbers, which is not usual in sources that present this solution as the solution; again, not at all neutral, just a setup for saying why it's wrong. And the figure is similarly peculiar, being focused on the illustrative numbering of which door is originally picked. Look at the simpler figure that I used, which illustrates the simple solution as often presented. Also, you source says "Now suppose that a contestant decides in advance to play the “stay” strategy," but it does not rule out the possibility that the player will choose to use the "stay" or the "switch" strategy after the host reveals a goat. He thens goes on to show how the probabilities can be different from the 1/3 and 2/3 if the host isn't choosing equally between the doors. But that stretch, though valid, is still not the only possible viewpoint on the problem of deciding after the host opens a door. In the common usual reading of the problem, the player has no probabilistic model of the host behavior, so asking for probabilities conditioned on that behavior is just outside the universe of what the player is going to be able to consider in making their strategic choice. So choosing after a door is open is not a different problem from choosing before. This is a common interpretation, not the only one; we need not argue about which interpretation is better; just don't present the solution corresponding to one interpretation in the terms of the other, OK? Dicklyon (talk) 04:38, 5 April 2009 (UTC)

Diagram

I just created this. Does this help make it clearer at all? If so, we could add it to the article.

DavidSJ (talk) 02:20, 31 March 2009 (UTC)

Clear as mud; let's stick to the pictorial ones. Dicklyon (talk) 02:33, 31 March 2009 (UTC)

New "Traditional solution" section

Given the complexity of the discussions, I thought it best to just be bold and rewrite the "traditional" solution as simply as possible, with no hint of the conditional approach, using the old figure where the only three relevant cases are what the contestant had behind their orignally picked door. Then, a separate section to say why some object to the that approach, before the other solution. I picked a ref that I think predated any mention of conditional solutions in the literature, yet which explicity says that the host's action doesn't change the probability of the car being behind the originally chosen door. I'm not claiming that it's rigorous or correct, just that it's traditional, which seems indisputable; we could add more such sources if anyone thinks that will help.

Comments? Dicklyon (talk) 03:09, 1 April 2009 (UTC)

Too bold. Nijdam (talk) 09:54, 1 April 2009 (UTC)

Nijdam, if it's so bold that you feel a need to revert, you should at least say way. I've put it back for now, as it's clearly better than the confusing mess that was there before. Dicklyon (talk) 14:31, 1 April 2009 (UTC)

I'm not too happy about "It is assumed that when the host opens a door to reveal a goat, this action does not give the player any new information about what is behind the door she has chosen, so the "proper Bayesian" still sees the probability of there being a car behind the chosen door as 1/3 ..." The fact that no information is revealed is exactly what those who get fooled have difficulty seeing -- their fallacy is exactly the assumption that revealing a goat will reveal information and somehow "compress" the total probability into the remaining doors. Just asserting the right conclusion it with nothing more than "it is assumed" to back it is not very convincing.

The key step in the elementary argument is "suppose you make your decision to switch or not before Monty opens a door", followed by an argument that deciding later makes no difference (essentially, something like "you already knew you were going to be looking at a goat and an option to switch. Knowing which door that goat is behind is not going to help you decide better"). When this two-step argument is compressed into "it is assumed", the whole thing in my eyes collapses into handwaving. –Henning Makholm (talk) 15:03, 1 April 2009 (UTC)

Does anyone think Dick's version is any better than the version I suggested above? I'm somewhat perplexed why Dick has created a new version rather than discuss the version I suggested. I'm perhaps somewhat biased (as is Dick) but I'll offer the following observations:

Dick's source is an analysis of DNA sequences, which seems like a distinctly odd source for a math problem. The source I reference is a probability and statistics textbook.
Dick's version deletes the image showing the conditional solution - my version includes consistent images for both solutions.

I would suggest that if we're unhappy presenting the previous "confusing mess" it would be better to switch to the version I suggest. -- Rick Block (talk) 16:22, 1 April 2009 (UTC)

Just to recap, point of my attempt at a new version is to present the traditional solution untainted by the more "modern" conditional solution. Rick's proposal recasts the traditional solution as a solution of a different problem, which he calls "probability of winning for all players who switch." Sure, it can be interpreted this way, but that's not the problem that was asked, nor the problem for which the tradtional solution is offered, but rather a recasting in light of the modern conditional way of looking at things. I think we need to avoid that, and just present the solution that was commonly accepted before the conditional one came along. Similarly, I picked the old figure because it better illustrates the traditional solution; I think this is better than picking a figure designed to set up the conditional solution; of course, putting that figure back in the next section would be fine. As for the source, this was just a concise one that I found that had the pretty much exact statement of the problem, as opposed to the sometimes different or over-simplified statements sometimes found with the traditional solution. It's in an article on Bayesian analysis, and it hardly matters that the field is DNA.

As to me being biased, I'm not sure why you say that. I have no preconception or bias in this that I'm aware of. Rick, back on 18 March you said you had no objection in principle to presenting an unconditional solution first, making clear when it is valid, and then introducing a conditional solution afterward, and you invited me that try it. Your proposed version is not in that form at all, and that's why I'm trying again to get one that is. And with the traditional solution "uncontaminated" by the idea that maybe it doesn't solve the stated problem; that can come next. Dicklyon (talk) 04:43, 2 April 2009 (UTC)

If a "popular solution" is presented first, it should be as short as possible, with no suggestive form or formulation giving it a status it doesn't deserve. It may read as: A popular way of understanding the problem is that by sticking to her original choice the player has a chance 1/3 of winning the car, hence switching will increase her chance to 2/3. With the additional remark: For a full understanding one needs to calculate the conditional probabilities, as is done in the next sections. Please improve the formulation and my English. Nijdam (talk) 12:35, 2 April 2009 (UTC)

I think your suggestion is an excellent starting point. Perhaps the 'Combining Doors' diagrams and some narrative could be incorporated? Glkanter (talk) 13:15, 2 April 2009 (UTC)

I think that adding For a full understanding one needs to calculate the conditional probabilities, as is done in the next sections would be over-representing that point of view. It's already represented as a criticism and as a subsequent section; the point of what I thought we had agreed to in principle was to present the typical, original, popular, traditional solution first, untainted by that alternate point of view, and then introduce that other POV next. Sounds like you guys aren't liking that, and are insisting in having the conditional-solution-needed POV intrude on the simple solution section. Do I understand correctly? Dicklyon (talk) 14:29, 2 April 2009 (UTC)

You're (d) right, I don't like the "popular explanation" at all, because it is wrong. And more and more I notice, teachers and pupils in highschools, who like to "discuss" this for them so highly interesting problem, to be satisfied with the popular explanation, missing the point what it is all about. Namely: conditional probabilities! So let us make things clear, and not leaving any ambiguity. Nijdam (talk) 17:30, 2 April 2009 (UTC)

I read Henning Makholm suggestion, and I like it very much. So let me expand my suggested text: A popular way of understanding the problem is that if the player makes the decision not to switch before Monty opens a door she has a chance 1/3 of winning the car. This chance is not affected by Marty opening a door, hence switching will increase her chance to 2/3. For a full understanding one needs to calculate the conditional probabilities, as is done in the next sections Nijdam (talk) 17:42, 2 April 2009 (UTC)

Nijdam, I really do understand your suggestion and your position, and the reasoning for preferring a solution that treats the conditional probabilities. But your POV that "it is wrong" is not the only POV; NPOV suggests that we should present this common/popular/tradtional solution, even though from some points of view it is considered wrong, and then go to say what those alternative points of view are. You can't base the structure of the whole article around your one POV. Dicklyon (talk) 20:08, 2 April 2009 (UTC)

Dick, it's always easy to judge one's opinion as POV, but it's not only my personal opinion, it is also found in the paper of Morgan et al. and others as you may well know. So let us present the right solution first, and then come with the popular explanation. Ok? BTW do you agree that the popular explanation is not complete? Nijdam (talk) 15:05, 3 April 2009 (UTC)

Dick - The bias I alluded to above was that we each would naturally prefer our own writing. You've used the words "traditional" and "modern" a couple of times to refer to the unconditional and conditional approaches. These seem not very accurate to me. Perhaps "non-mathematical", or "intuitive", or something might be a better terminology for the unconditional approach - although I realize this runs into your view that this is a POV matter - but calling the conditional approach "modern" is simply absurd. In Gardner's 1959 version of the Three Prisoners problem he has the guard secretly flip a coin to decide in the case corresponding to the MHP host picking between two goats. The notion that an unconditional solution is sufficient was popularized by vos Savant more than 30 years later. The MHP has been known in mathematics, and known to be a conditional probability problem, since 1975. The two papers that are the best source for this view are the Morgan et al. and Gillman papers, published in late 1991 and early 1992 respectively (roughly a year after vos Savant's original column - pretty much directly in response to her columns). This is not a "traditional" vs. "modern" issue - it's an expert vs. non-expert issue.

Rather than rewrite, can we work on revising the proposal I made above to address your concerns? I don't think this should be terribly difficult. For example, rather than say "There are two main approaches to solving the Monty Hall problem that answer slightly different questions." we could say "There are two main approaches to solving the Monty Hall problem." We have an issue with what to call the two approaches. If you're not willing to use the problems that the expert sources say they address to identify them, or any terms that convey some sort of value judgment between them (like "popular", or "expert", or "common", or "mathematically rigorous") I think we're left with a description of the solution itself. Perhaps "Examining outcomes of switching versus not switching" and "Examining conditional probabilities after the host opens a door". -- Rick Block (talk) 01:57, 3 April 2009 (UTC)

I agree with Rick on the absurdity of calling this a "Traditional" vs "Modern" difference. In addition, this new "Traditional Solution" section is poorly written and confusing. It forces the reader to dance between a frequentist and a Bayesian view, as if he or she had already gone through this talk page and digested pages of conditiona/unconditional mumbo-jumbo even before reading the article. In fact, I am tempted to be bold and revert immediately, but will wait for the argument to settle a bit. Note, I don't have any problem with presenting a frequentist solution (a.k.a. unconditional) along with the bayesian (conditional) one, especially because the more mathematically naive readers will be more attuned to the former than the latter. But please let's not pretend that the frequentist interpretation is "The Correct One(tm)", when it rather obviously isn't (and I won't repeat Rick's excellent argument here for why it isn't).glopk (talk) 16:43, 3 April 2009 (UTC)

I strongly oppose the recent alterations to the article. There is nothing traditional to the so called "traditional" solution, and what is worse, the picture doesn't cover the text, as in the picture the player's choice varies. Nijdam (talk) 19:15, 3 April 2009 (UTC)

I don't have a strong opinion about what to call them, except that the "conditional" idea shouldn't be used to frame the other solution by calling it unconditional. Perhaps "popular" was better in that it expresses the idea of both common and less rigorous and expert. What I object to is just letting the POV of the experts who want to treat it as a problem in conditional probabilities frame the whole structure of the article and the solution, when a perfectly good popular and traditional solution, agreed to by many other smart people, gives the right answer for the problem as usually understood. Each time I think we some agreement in principle, it does out the window with bickering by those who want everything to be framed relative to their "correct" conditional POV. This isn't right. Dicklyon (talk) 23:43, 3 April 2009 (UTC)

The picture illustrates what the text describes: doors have no numbers and the player has chosen either the car, or goat A, or goat B, with equal probabilities. This is really the simple way to look at it that makes it clear that specifying door numbers is irrelevant. Dicklyon (talk) 23:45, 3 April 2009 (UTC)

The "popular" solution is unconditional, why do you object to calling it what it is? Are you happier with this old version? It presents an unconditional solution, says it's correct and then says what the experts say about it as a transition to the conditional solution (and apparently doesn't make anyone happy - Martin Hogbin and Glkanter have been complaining about that version for months from the one side and Nijdam didn't like it either from the other side). I still don't understand what you don't like about my proposal above. Can we work out changes you think would be needed to address your concerns? -- Rick Block (talk) 02:14, 4 April 2009 (UTC)

I object to calling it conditional because that's how it's framed by those who argue that it's incorrect. I think "unconditional" is not a useful characterization of the reasoning except when you're trying to contrast it with the more elaborate "conditional" approach. Neither of the sources present it as "unconditional". Are there sources that call it that, other than those that are presenting the conditional solution as better? Dicklyon (talk) 02:00, 5 April 2009 (UTC)

How complicated is it???

I might have missed something, but surely it's not a difficult problem. Two times out of three, the host indicates to the contestant what door the car is behind by having no choice but to open the other door, therefore it's always worthwhile to swap, because two times out of three he will have chosen the correct door for you. It's more of a psychological trick or an illusion than any great mathematical conundrum. —Preceding unsigned comment added by 88.97.18.12 (talk) 23:57, 2 April 2009 (UTC)

I agree. Glkanter (talk) 00:28, 3 April 2009 (UTC)

I can't understand the fuss. You know that there are two goats and one car. You know that the host has to open one of the two remaining doors. You know that 2/3 times, he will be faced with one car and one goat, and have to open the door with the goat behind it, leaving the car behind the remaining door. Ergo, if you always switch, you win the car 2/3 of the time. What alternative explanations are there? The illusion is that all doors start out with a 1/3 chance, but after the host has opened one door, he has increased the chances of the remaining door having the car behind it to 2/3. Even if you happen to have chosen the car, over the long run you are bound to win. —Preceding unsigned comment added by 81.137.245.144 (talk) 09:10, 3 April 2009 (UTC)

The fuss is that mathematicians say the problem clearly asks about a conditional probability, and that the answer "2/3" is correct only if the host is constrained to pick randomly between two goats (in the case the player picks the car). See the second question in this FAQ. -- Rick Block (talk) 13:47, 3 April 2009 (UTC)

It's a conditional probability problem - you're standing in front of two closed doors and looking at a goat. Of course across all players who switch, 2/3 will win the car - but what about you, the player standing in front of two doors? Maybe the other guys (whoever they are) always win and you not so often. If the host has opened door 3 your chances of winning are the probability that the car is behind door 2 (i.e. 1/3). Your probability of losing by switching is the probability that the host has opened door 3 (let's say this is 1/2) times your probability of having picked the car - i.e. 1/3 * 1/2 = 1/6. Winning 1/3 versus losing 1/6 means winning 2/3. But why is the probability the host opens door 3 when you've picked the car exactly 1/2? Unless we specify the host has to flip a coin or something the host can open whatever door he'd like in this case. This means unless we say how the host decides in this case, the host opens door 3 with some probability between 0 and 1. Let's see what happens using these extremes:

host opens door 3 with probability 0 means you win 1/3 (when the car is behind door 2) and lose (when the car is behind door 1) 0 * 1/3 = 0. Winning 1/3 versus losing 0 means winning with probability 1.
host opens door 3 with probability 1 means you win 1/3 and lose 1 * 1/3 = 1/3. Winning 1/3 versus losing 1/3 means winning with probability 1/2.

This means if the other guys always win you win 1/2 the time. If the host opens a door, no matter how he picks when you've picked the car you win at least 1/2 and maybe you're one of the lucky ones who win with probability 1. Not quite so simple, but yes you should switch every time. And, if you do, you'll not only win 2/3 of the time overall but your chances of winning every time you switch are between 1/2 and 1. -- Rick Block (talk) 02:51, 3 April 2009 (UTC)

And, if the host picks randomly when you've picked the car, you'll win with probability 2/3 every time. But even if you don't know this, you'll win with probably 2/3 overall (and between 1/2 and 1 every time). -- Rick Block (talk) 03:02, 3 April 2009 (UTC)

That's only one of the two prominent points of view on the problem, and we need to represent both, preferably with the traditional one first since it's older and simpler and widely known. By the other POV, if the player doesn't know anything about how the host is going to choose a door, and even if the host is doing something as biased as choosing the left door when he has a choice, the player can not base their estimate of the probability on that, since they don't know it, and so will still be correct -- or as correct as possible -- in using an unconditional model and estimating their probability of winning at 2/3. It's only when they have access to information about the host's behavior that they can use that information to estimate different probabilities, and even then the answer about what they should do, and why, doesn't change. So let's not let this more complex POV about rigorous conditional probabilities derail the discussion of the simple solution. Dicklyon (talk) 05:29, 3 April 2009 (UTC)

Yes, Dick you are quite right. The simple unconditional problem is the one that is notable and interesting and is the one that should have the most prominence in this article. On the arguments page I explain how the paper that is central to the 'academic' approach (that by Morgan et al) conjures up a more complex explanation by imposing arbitrary and unjustified choices. Martin Hogbin (talk) 08:41, 3 April 2009 (UTC)

Let us be precise in our phrasing: there is no such as an "unconditional problem" in the MHP. We're discussing the need for a "conditional solution", and the flaw in the so called "unconditional solution". Nijdam (talk) 19:22, 3 April 2009 (UTC)

That is not what Morgan say. They give a statement of the unconditional problem. Perhaps we could agree on 'unconditional formulation'. Martin Hogbin (talk) 20:45, 3 April 2009 (UTC)

Ok, it is possible to formulate an unconditional problem, that seems to be related with the MHP. It reads: From 3 doors only 1 hides a car, without you knowing which one. Pick a door. What is the probability you pick the car? It may be formulated with much more wording. Hardly an interesting problem. Morgan mentions this, because the "simple solution" is addressing this problem. Nijdam (talk) 11:35, 4 April 2009 (UTC)

Actually, that is exactly the MHP as many people interpret it. As 88.97 wrote above "It's more of a psychological trick or an illusion than any great mathematical conundrum." Because many people insist that the odds become 1/2 & 1/2, not 1/3 & 2/3. That's why it's the world's most famous paradox. Not because people care 'which goat will Monty reveal?' Glkanter (talk) 13:40, 4 April 2009 (UTC)

Too bad for them!! And for you?! Nijdam (talk) 14:50, 4 April 2009 (UTC)

No, it's too bad for the innocent Wikipedia reader who is subjected to all that stuff you self-proclaimed experts have cluttered up the MHP article with. Can't you and Rick find a more appropriate forum to demonstrate your mastery of Probability? Because this article is not the place for it. Glkanter (talk) 15:11, 4 April 2009 (UTC)

Morgan's 'mastery of probability' only exists because they set the problem up to specifically create it. It has now been agreed that it only applies to the case where the producer's possible preference for placing the car is ignored but the host's preference in opening a door is considered important. Martin Hogbin (talk) 20:52, 4 April 2009 (UTC)

Dick - is there some reason you're not addressing my comments above (previous section)? There's no clear consensus for your version, so adding it still seems premature. -- Rick Block (talk) 14:18, 3 April 2009 (UTC)

I believe I've now answered above. Let me know if you can see what's in the way of reaching consensus on this. Dicklyon (talk) 20:55, 4 April 2009 (UTC)

??? As far as I can tell, you haven't responded to comments from Nijdam, Glopk, Henning, or myself. At this point, I'd say there's a better consensus for the version I've proposed than the one you've edited into the article (favorable comments from Father Goose and Nijdam and arguably Glopk vs. no one other than you apparently supporting your version). On the other hand, it's also clear to me there is not a general consensus for either of these versions. I think some slight variation on the version I've proposed is more likely to generate broader support than your version. What has to change in this version to address your concerns? If you'd rather simply edit the version above than discuss changes, please feel free (diffs will be in the history). I've made some changes already attempting to address what I think you've objected to. Better? -- Rick Block (talk) 00:49, 5 April 2009 (UTC)

Rick, please do not draw any conclusions whereby you believe there is any form of consensus for your proposed changes. Simply put, they represent more of the same. Which many of us are trying to explain to you we disagree with very strongly. Glkanter (talk) 01:51, 5 April 2009 (UTC)

Exactly; there seem to be several people who support this general direction; I'd rather seem them work on fixing up the article to be better than more of this incessant bickering that is this talk page. The comments of Nijdam seem to be rather empty; just doesn't like it. And Henning's comments are more about why the other solution is better, rather than commenting on the article change I made; he did suggest we not pretend it is "the correct solution", which I agree with; we need to present all POVs without taking sides. And I already agree we don't need to call it "traditional"; waiting for constructive alternatives. And Rick, which comments have I missed that you want a response to? Dicklyon (talk) 02:03, 5 April 2009 (UTC)

Clearly Glkanter believes that we need a straightforward presentation of the simple popular solution. Martin Hogbin says it "should have the most prominence," but I see no need to go that far; he says the Morgan et al. paper "conjures up a more complex explanation by imposing arbitrary and unjustified choices," which I don't think is an opinion that can be justified from sources. Nevertheless, it does help to clarify the sharp distinction between the different points of view on what the problem is and what the solutions are. As long as we continue to have people who side with these different POVs trying to get the article to go their way, we're not going to get unwedged. What we need is a fair representation of both points of view. That's exactly why the simple solution needs to be presented simply, without value judgement as to whether it correct or adequate or not, and without framing it in the terms of the POV that says it's wrong. After that, the alternative more complex POV should be introduced and discussed. Can I hear from all whether they agree with this general approach or not? Dicklyon (talk) 04:11, 5 April 2009 (UTC)

Dick - Comments not addressed: the generally weak references (not up to par for a featured article - and adding more weak references doesn't help, what is needed is an authoritative reference from a math journal or math textbook), the image issues (doesn't match the problem description and you've deleted the conditional image), the prose issues Glopk pointed out, and my request that you say what you don't like about the alternative I've proposed (which is an attempt to simply present the solution Glkanter and Martin prefer, without any value judgment - have you even read the changed version above?). I'd rather we discuss changes here first, before changing the article but you seem to be completely unwilling to following this approach. Directly answering your question, I'm OK with the general approach, however I think we need to be very careful not to present something that experts would consider to be flat-out wrong. -- Rick Block (talk) 05:00, 5 April 2009 (UTC)

No, I disagree on the refs; the math journals primarily represent the more complicated conditional POV on the problem, because they're mathematicians. The simpler POV isn't something that you could publish a math journal article on, but it's still a viable POV that needs to be represented. Don't take the ScienceApologist route and try trump NPOV with SPOV (scientific point of view). As for the images, the image used illustrates the solution as described; if a conditional image was removed, feel free to put it back in the conditional section. I worked on the prose a bit already, and am happy if Glopk can do more to improve it. And I commented on what's wrong with your version above. Dicklyon (talk) 05:59, 5 April 2009 (UTC)

Combining Doors Solution

I proposed about a week ago that the Combining Doors Solution receive priority in the article, without it being called 'inadequate'. Still waiting for any informed criticisms.

How long is it customary to wait before editing the article? Glkanter (talk) 03:27, 4 April 2009 (UTC)

The Combining Doors Solution, in spite of its interesting and promising name, is essentially the same as the "simple solution". So, let us not give it much attention. Nijdam (talk) 11:38, 4 April 2009 (UTC)

At this point we have 3 alternatives on the table. This one, the one I've suggested, and the one Dick has edited into the article. This one has generated much less commentary than the others, but none have any clear consensus so editing any of them into the article is premature. You should take two other proposals being offered up after this one as objections to this one. -- Rick Block (talk) 12:16, 4 April 2009 (UTC)

I think both of your answers are non-answers.

Please treat this the same way you would an article nominated at WP:FAC. There, each objection must provide a specific rationale that can be addressed. Do you have any specific suggestions for how to improve the proposal above? -- Rick Block (talk) 18:19, 29 March 2009 (UTC)

The solution I refer to is already in the article, and it refers to 4 separate published sources. Morgan doesn't address it. Is there a published source that takes issue with it? Glkanter (talk) 13:33, 4 April 2009 (UTC)

From Morgan et al.:

Solution F5. The probability that a player is shown a goat is 1. So conditioning on this event cannot change the probability of 1/3 that door 1 is a winner before a goat is shown, that is, the probability of winning by not switching is 1/3, and by switching is 2/3.

The combining doors solution is simply a rephrasing of this solution. Rather than present this as the first solution (with the addition of unreferenced "equations"), I think it is more appropriate to present the switch vs. stay comparison (as I suggest above) from a math textbook which is a more authoritative source than any of the references for the combining door solution. -- Rick Block (talk) 15:40, 4 April 2009 (UTC)

Let's keep in mind that the paper continues: Solution F5, like F1 is a true statement that answers a different problem. F5 is incorrect because it does not use the information in the number of the door shown. Nijdam (talk) 20:25, 4 April 2009 (UTC)

Yes, but also keep in mind the Morgan's POV is but one way to frame the problem. Many do not consider it to be the solution to a different problem from the one stated, even if it's different from the other other one that Morgan analyzes, in which the host's choice of door can provide some extra information about the probability of the original door being the car. Dicklyon (talk) 04:02, 5 April 2009 (UTC)

Thank you, Rick. Can you please expand your response so that I can understand where Morgan is finding fault with the Combining Doors solution? I'm sorry, but it's not clear. You're right, my equations are not referenced. They don't go in. Comparing the two proposals? I think the Combining Doors is more concise and the diagrams are more intuitive. And all they show is a goat behind a door, with no comment on where the car is. Which, imho is what the puzzle is really about. Glkanter (talk) 16:05, 4 April 2009 (UTC)

No more comments? I'll make this change later today. Glkanter (talk) 10:27, 10 April 2009 (UTC)

Dick Lyon, except for removing the diagram, you made some nice enhancements. I always liked the way it flowed (sort of) from 1/3, 1/3, 1/3 (although the diagram did not specifically say this, I wish it had) before the goat is revealed, to the 1/3, 2/3, 0 after the goat is revealed.

On a related note, what is with the diagram above it? Are the heads necessary? I could see where someone might find it offensive. Glkanter (talk) 06:23, 11 April 2009 (UTC)

More than three choices

I consider this an encyclopedic question, because it is just as much for my information as it is for my curiosity.

In the game show Deal or No Deal, you may have noticed that, when a contestant is down to two cases, the host offers them a chance to switch cases. Would switching cases increase your chances - even slightly - of getting the higher value case, due to this paradox, and if so, how would you compute that probability. I doubt your chances of getting the higher case would become 25/26. —Preceding unsigned comment added by Wikieditor1988 (talk • contribs) 03:44, 5 April 2009 (UTC)

Each choice in Deal or No Deal is a random choice. With N cases left, the player's chances are 1/N of having the highest prize left. So, no advantage in switching. This is like the "random Monty" variant. -- Rick Block (talk) 03:56, 5 April 2009 (UTC)

Consider comparing the Monty hall problem to Deal or No Deal. If you want to win the top prize (£250,000), then your probability of picking it to start with is 1/22. If you then eliminate the other 20 boxes and are left with the £250,000, then an application of the Monty Halls problem would tell you to switch, because:

Imagine that there are 22 doors. You select one door, and are then shown the other twenty. This means that you have a 21/22 chance of winning the prize if you switch. Whether the host knows where the prises are doesn't matter, because the situation is the same. You have picked a box, and then been shown 20 that you don't want. Therefore switching is best.

Also, even though the box picked is random, at the end you still get left in a situation where you have seen what is behind 20 boxes, and then have the option to swap (see above). Therefore I think the probability of winning big if you swap is bigger than 1/2. If you think there is something wrong with my logic, then please make me aware of it.

(Another statistical quirk is that if you work out the probability of not taking out the quarter of a million by the sum 20/21 x 19/20 x 18/19... x 2/3 then the answer is 2/21. This means that on average 2 games out of 21 should occur where the £250,000 is left as one of the last two boxes.)Cricketmad5Wk (talk) 20:03, 24 April 2009 (UTC)

If we start with 22 boxes, yours and all the rest each have a 1/22 chance of being the grand prize. If you pick one randomly and open it and it is not the grand prize there are 21 left. Each of these 21 (yours and all the rest) now have a 1/21 chance of being the grand prize. Why? Because this is a conditional probability problem. The only possibility you've eliminated is the one where the opened box had the prize. All other possibilities are still in play. The conditional chance for each box is now the unconditional chance (1/22) divided by the total probability that you're in this case (21/22), i.e. (1/22) / (21/22) which is 1/21. If you open another random box and it isn't the grand prize you have another conditional probability problem just like the first one. The remaining (now 20) boxes each have a probability of (1/21) / (20/21) or 1/20. This continues until there are two left and the chances are 1/2 for each box.

This is entirely different from the host randomly opening boxes but avoiding your box and the grand prize (as in the Monty Hall problem). If this is what is going on, then after the first box has been opened you have a 1/21 chance of being in each situation where one specific box is opened. In each of these, you have a (1/22) * (1/21) chance of having the grand prize. There are 21 of these possibilities, so your overall chances of having the grand prize are 21 * (1/22) * (1/21) which is 1/22. The remaining 21/22 chance of the grand prize being somewhere is split evenly among the other 20 boxes, so each of them now has a (21/22) * (1/20) chance of having the grand prize. When the second box is opened, you have a 1/20 chance of being in any of these specific possibilities, so in each you have a (1/22) * (1/20) chance (and there are 20 of these, so you still have a 1/22 chance overall). The remaining 21/22 chance is now split among only 19 so they each have a (21/22) * (1/19) chance. This continues so with N boxes left you have a 1/22 chance and the remaining boxes each have a (21/22) * (1/(N-1)) chance. When we get to two boxes left, your box still (!) has a 1/22 chance and the other box has a 21/22 chance.

Completely random versus random except for the grand prize and your box makes a huge difference. -- Rick Block (talk) 02:11, 25 April 2009 (UTC)

Here is a little something that I came up with myself to prove you right(i.e. the probability is the same)

Let the boxes equal the letters A-V

Let the prize that you want to win be behind box A (It doesn't matter which box the prize you want to win is in, [also assume biggest prize])

Now if you pick box A, then the probability of getting through to the end of the game with the prize you want still in the game is 1(certain).

This occurs is (1/22) cases. (1)*(1/22)=(1/22)

If you don't pick box A then the probability of you getting to the end of the game without taking out the prize you want is:

(20/21)*(19/20)*(18/19)... *(1/2).

This can be re-written as:

((20!)*(1!))/(21!).

The sum works out with a value of (1/22) = 0.04545454545454545454545 This occurs is 21/22 cases so:

(21/22)*(1/22)= (1/22)

Therefore, you are correct, and the probabilities remain the same

N.B. I came up with this by accident when trying to prove mathematically that the opposite was true. Perhaps it should be posted as a proof for the probabilities remaining the same?? .Cricketmad5Wk (talk) 16:02, 25 April 2009 (UTC)

Original proofs should not be posted in articles - see WP:OR. It's fine to discuss them on talk pages, but unless something is published in a reliable source it can't be used in an article. -- Rick Block (talk) 17:37, 25 April 2009 (UTC)

The stages in the decision

I'll try to find out where each of you stand. (Again I use X=choice, C=car, A=open and the rules)

1. Before the player made her choice

The probability she picks the car is: P(X=C)=1/3. This means that on the average 1 out of 3 players will choose the door with the car. One may reason from here that switching after the choice of door and the showing of a goat will on the average result in 2/3 winning the car.

2. After the player has chosen a door and before the quizmaster opens one

If the player chooses door x, we now have to condition on the event {X=x}. For every x the (conditional) probability she picks the car is: P(C=x|X=x)=P(C=x)=1/3 (because X an C are independent and the car is placed randomly). One may reason from here that for every choice x switching after the showing of a goat will on the average for players with this choice result in 2/3 winning the car.

3. After the player has chosen a door and the quizmaster has opened one

If the player chooses door x and the quizmaster has opened door a (≠x), we now have to condition on the event {X=x, A=a}. For every x and a (≠x) the (conditional) probability she picks the car is: P(C=x|X=x,A=a)=1/3. Why is this?

We can directly calculate it:

P(C=x|X=x,A=a) = P(A=a|X=x,C=x)P(C=x|X=x)/P(A=a|X=x) = {1/2.1/3}/{1/2.1/3+1.1/3+0/1/3}=1/3

Or use the symmetry. For x,a,b all different:

P(C=x|X=x,A=a)=P(C=x|X=x,A=b)=(let's say)t

Now is:

=t{P(A=a|X=x)+P(A=b|X=x)} = t

hence:

t=P(C=x)=1/3

For every choice x and opened door a switching will on the average for players with this choice and opened door, result in 2/3 winning the car.

Really unconditional is the reasoning in case 1. Although formally conditional on the choice, due to the independance of the choice and the place of the car, case 2 may also be considered as unconditional. Case 3. is definitely conditional, and only in this case has the player made her choice and the quizmaster opened a door. I cannot imagine someone to disagree with these considerations. The controversy just originates from the idea that case 1 or case 2 forms a solution to the stated MHP. Does anyone agree with this? Nijdam (talk) 12:52, 5 April 2009 (UTC)

I don't see the point of this discussion. It doesn't really matter how we analyze the problem or solution. What matters is what analyses we find in reliable sources. Dicklyon (talk) 15:00, 5 April 2009 (UTC)

Yet I'm interested where you stand? Nijdam (talk) 22:22, 5 April 2009 (UTC)

Where I stand is simply that the different points of view on the solutions need to be fairly represented; your way of framing the problem is alinged with one of those POVs, I think, and going into it in more depth is sort of off-topic here, I think. Or if I misunderstood, what were trying to find out with this posting? Your statement "If the player chooses door x and the quizmaster has opened door a (≠x), we now have to condition on the event {X=x, A=a}" represents one POV clearly; we get that; let's just no lose sight of the other POV in which you don't have to do that. Dicklyon (talk) 22:29, 5 April 2009 (UTC)

Yet you haven't said what you think about the problem, let us say: your POV. You often use the term POV. Is it because other opinions than yours are just POV's? All I have explained can be found in scientific articles (although I do not need them for such a simple problem). My concern is, what is demonstrated in the following reaction of Candy.Nijdam (talk) 14:36, 6 April 2009 (UTC)

In the literature on this problem, there are two main points of view: one simple analysis, that you call unconditional; and one conditional analysis by people who say that the other is insufficient or solves the wrong problem. Editors here have polarized over these two points of view, and conduct lengthy arguments over the merits of them, rather than discussing how to fairly represent both in the article. My position is that both points of view are reasonable, and that the article has been firmly in the grip of the people who support the conditional point of view; that's why I'm pushing in the other direction, to get back to some balance and objectivity and proper weight. As far as I can tell, I'm the only one willing to not take sides, but maybe I'm missing someone. Reactions by people like Candy, who haven't yet come to understand the issue, are natural, need to be politely addressed and dismissed, but shouldn't confuse the main discussion. Dicklyon (talk) 15:05, 6 April 2009 (UTC)

I don't understand why you have gone to these lengths to explain what is extremely simple. Regardless of what happens, if the contestant sticks with their original choice the probability of getting the car is 1/3. You seem to have made a bit of a mountain out of a molehill? --Candy (talk) 04:35, 6 April 2009 (UTC)

This is the idea I'm afraid a lot of people have. And I think the "simple solution" propagates this. That's my concern.Nijdam (talk) 14:36, 6 April 2009 (UTC)

Are you agreeing or disagreeing with me Nijdam? --Candy (talk) 23:18, 6 April 2009 (UTC)

Please see the "Conditional solution" section of the article, or the FAQ at the top of this page. If you'd like to discuss this, please use the /Arguments page. -- Rick Block (talk) 03:17, 7 April 2009 (UTC)

Outline changes

Can we have a focused discussion about the recent outline changes (and, after we agree on an outline we can discuss the individual sections)? It's not clear to me where folks stand overall on these. The article now has

Problem
Popular solution
Criticisms of the traditional solution
Conditional solution

all at the same heading level. I'd prefer

Problem
Solution
- Popular solution
- Conditional solution

with the bit in the "criticisms" section about experimental validation moved to the "Popular solution" section. I'd simply delete the other parts of this section (starting with "Nevertheless ...") since they are addressed in the "Conditional solution" section.

Related to this, I object to including the unconditional solution in the lead and think the paragraph starting with "Simple probability ..." should be deleted.

Other opinions? -- Rick Block (talk) 17:06, 5 April 2009 (UTC)

The alternative is to leave the simple (popular/traditional) solution in the lead, and keep the criticisms from the conditional guys outside the presentation of the simple solution. I think the current structure works better, with the "criticism" section, what you want to call it, making the transition to the more complex analysis. I'm OK moving the "experimental validation" part into the popular solution section, as long as it's not phrased according to the conditional POV. The outline you proposed would also get complicated, as the "Problem" section would have to already go into the two different points of view about how to interpret the problem statement; it's probably best to leave those POVs, especially the more complicated one, to the corresponding solution section. Dicklyon (talk) 17:30, 5 April 2009 (UTC)

As in the example above, the "Solution" section can simply say there are multiple approaches (surely you're not suggesting that saying there are multiple approaches is POV?). The point about deleting the unconditional solution from the lead is that including it but not the conditional solution is favoring this POV. -- Rick Block (talk) 18:04, 5 April 2009 (UTC)

Yes, it would be fine to say there are multiple solutions, in the lead; but we should probably also say the result, that it is always advantageous to switch because the probably of winning will be double that way, under the usual interpretation of the problem (we don't need to say exactly how we arrive at it, or under what conditions it is the exactly answer, until the sections that follow); but to leave that out of the lead is probably not a good idea. Dicklyon (talk) 19:08, 5 April 2009 (UTC)

We seem to be talking about two different leads here. In the article lead, not the section lead, I'm suggesting deleting the paragraph that says "Simple probability indicates ..." - this would leave the sentence from the previous paragraph that says "In fact, in the usual interpretation of the problem the player should switch — doing so doubles the probability of winning the car from 1/3 to 2/3.". Then, in the solution section, I'm suggesting under "Solution" we say "There are two main approaches to solving the Monty Hall problem." (as in the linked section above) and present both as subsections. -- Rick Block (talk) 19:40, 5 April 2009 (UTC)

Yes, removing that paragraph would be fine. I said why I don't like that approach to the solutions, though. Dicklyon (talk) 20:01, 5 April 2009 (UTC)

I would prefer something along these lines:

Problem
Popular solution
- Sources of confusion
- Aids to understanding
- Criticisms of the popular solution
Conditional solution
- Sources of confusion
- Aids to understanding
- Criticisms (or limitations) if the conditional solution

Where each solution has its own 'Sources of confusion' and 'Aids to understanding', and 'Criticism' section. At the moment these two sections merely add more confusion and less understanding to the popular solution. I accept that we may have a problem with reliable sources for the 'Criticisms (or limitations) if the conditional solution'. Martin Hogbin (talk) 18:55, 5 April 2009 (UTC)

Is it just me or does this appear to be illogical?

The paragraph, "Simple probability indicates that the player has a 2/3 chance of initially choosing a goat. Players who unconditionally stick to that choice therefore have only a 1/3 chance of winning the car. Players who unconditionally switch get the opposite of their original choice, so they have a 2/3 chance of winning a car.", appears to me to be illogical. If they unconditionally switch they still have a 1/3 chance. --Candy (talk) 04:41, 6 April 2009 (UTC)

We're likely deleting this paragraph anyway (see just above), but why do say it's illogical? Unconditionally switching means deciding to switch before the host opens a door knowing that the host will open a door. If players who stay win 1/3 of the time, since there are only two closed doors at the end players who switch must win 2/3 of the time (right?). -- Rick Block (talk) 13:21, 6 April 2009 (UTC)

Quite so Candy. When I added this bit it said "Players initially have a 1/3 chance of choosing the car and a 2/3 chance of choosing the goat. Players who stick to their original choice therefore have only a 1/3 chance of winning the car (and a 2/3 chance of getting a goat). Players who switch always get the opposite of their original choice so they have a 2/3 chance of getting a car (and 1/3 chance of getting a goat)", but this is Wikipedia. Martin Hogbin (talk) 21:31, 6 April 2009 (UTC)

Let me go to the source perhaps of the problem with this 1/3 and 2/3. In the reference, [3], there is a statement:

"Using the “stay” strategy, a contestant will win the car with probability 1/3, since 1/3 of the time the door he picks will have the car behind it. On the other hand, if a contestant plays the “switch” strategy, then he will win whenever the door he originally picked does not have the car behind it, which happens 2/3 of the time."

The 2/3 is not the probability of winning after a goat is revealed. It is also not the probability of winning if the person simply decides to change their mind before any more information is revealed It is referring to the "switch strategy" defined in the article. Therefore, I continue to contend that the statement I quoted earlier in this Wikipedia article is incorrect and illogical. It appears, to me at least. to be taken out of context. --Candy (talk) 23:57, 6 April 2009 (UTC)

I want to stipulate that in the referred textbook, the authors also put it straight, that the "unconditional" solution is, allthough logically a sound reasoning, not addressing the posed question. The question, they argue, needs conditional probabilities to be answered. BTW: the problem posed is our MHP!.Nijdam (talk) 16:26, 7 April 2009 (UTC)

Of course you're right, but the statement is also right. The "unconditional" part means players who make the decision to switch or not BEFORE a goat is revealed and do not "simply decide to change their mind". If the decision to switch or not is made AFTER knowing which door was opened showing a goat then the problem must be solved conditionally. --67.193.128.233 (talk) 14:30, 7 April 2009 (UTC)

Why are you saying Candy is right? Her statement "If they unconditionally switch they still have a 1/3 chance" clearly indicates that her misunderstanding is not as subtle as you're making it out to be. In the context of the statement, there's only one door that can be switched to – nobody is talking about "changing your mind" before the host opens a door; if that's not clear enough, it could be fixed, but it's not "illogical". Dicklyon (talk) 14:35, 7 April 2009 (UTC)

It makes no difference why the players switch, when they decide to switch, what the host does (within the standard rules), or what new information they may or may not have received after they have chosen their door. Players who switch have a 2/3 chance of winning. That is all there is to it.

This is not the same as the MH problem as it is formulated by Morgan. To make the distinction clear, consider the extreme case where, after the player has chosen their door, the host says where the car is situated. Regardless of this fact, players who switch still have a 2/3 chance of winning. This statement refers to players in general not one particular player.Martin Hogbin (talk) 22:08, 7 April 2009 (UTC)

Can we not squabble about pointless things? We agreed to delete the paragraph from the intro. I just deleted it. This issue is now moot. -- Rick Block (talk) 00:53, 8 April 2009 (UTC)

We have all refrained from edit waring over the content, preferring to try to reach a consensus by discussion. I certainly did not agree to the deletion of that paragraph. Martin Hogbin (talk) 08:05, 9 April 2009 (UTC)

Martin - The suggestion to delete this paragraph had not been opposed by anyone. For reference, the paragraph said this:

Simple probability indicates that the player has a 2/3 chance of initially choosing a goat. Players who unconditionally stick to that choice therefore have only a 1/3 chance of winning the car. Players who unconditionally switch get the opposite of their original choice, so they have a 2/3 chance of winning a car.

My reason for suggesting we delete it is because the lead already says the player should switch and that doing so doubles the probability of winning the car. Adding this solution but not the expert discussion about this solution violates NPOV. Rather than get into this in the lead, I think it's far better just to state what the result is rather than any particular solution method. We can certainly discuss this further if you'd like, however the point of the lead is to summarize the entire article so it clearly must not favor one POV over another. -- Rick Block (talk) 14:18, 9 April 2009 (UTC)

Weasels ahoy!

"Nevertheless, some statisticians cricitize the solution as being incorrect for the problem as stated..." needs both a citation for specification. --Candy (talk) 23:21, 6 April 2009 (UTC)

No, they are answering a different question. Martin Hogbin (talk) 22:10, 7 April 2009 (UTC)

It still needs to say who, if we say it, and cite them; I think the proper citations are Morgan et al. and some of those; but someone should verify that this correctly reflects their position. Dicklyon (talk) 22:52, 7 April 2009 (UTC)

Morgan certainly do criticize the simple solution and they are cited throughout the article. Martin Hogbin (talk) 09:32, 8 April 2009 (UTC)

My suggestion above (see #Outline changes) is to delete this section and incorporate the experimental validation parts in the previous section. I believe the remainder is sufficiently covered in the current "Conditional solution" section. Dick said above the current structure works better, with the "criticism" section, what you want to call it, making the transition to the more complex analysis. Are there any other opinions on deleting the criticism section? -- Rick Block (talk) 00:47, 8 April 2009 (UTC)

I do not mind the criticism leading to the academic solution but I object to the simple solution being presented as in any way inferior to or less rigorous than the academic solution, it simply answers a different question. I also believe that there should be something to indicate the limited circumstances (still being discussed on the arguments page) under which the academic solution applies. Even considering only the Parade statement, there are many ways the problem can be formulated. To some of these the academic solution applies, to others the simple solution, and to others yet more complex solutions.

It is the simple formulation and solution that makes this problem notable and of interest to the general public, and that formulation should have a substantial, clear, and convincing section of its own. Martin Hogbin (talk) 09:32, 8 April 2009 (UTC)

This is my point as well, sort of. The simple solution needs to be presented as a solution; if there are sources that comment on how "rigorous" it is, that can follow, but we can't say it's somehow less without saying who says so. Even saying it solves a different problem is the opinion of its critics, not of its adherents, typically, so needs to be presented as such. Dicklyon (talk) 15:24, 8 April 2009 (UTC)

There we go again! Nijdam (talk) 16:03, 8 April 2009 (UTC)

What is the meaning of ...Imagine that you chose Door 1 and the host opens Door 3, which has a goat. He then asks you "Do you want to switch to Door Number 2?' Any idea?Nijdam (talk) 16:14, 8 April 2009 (UTC)

Firstly Nijdam let me point out that, like Morgan, you have misquoted the original question which, with my emphasis, actually says ,'You pick a door, say No. 1, and the host, who knows what's behind the doors, opens another door, say No.3'. The door numbers are just given as examples, the questioner surely does not want an answer that applies only to the specific case that the host has opened door 3.

Secondly let me quote from the commentary by Prof R G Seymann at the end of the Morgan paper. He says, 'Without a clear understanding of the precise intent of the questioner, there can be no single correct solution to any problem' (my emphasis again). Note that it is the intent of the questioner that we should consider. The original question was a comment from a reader of a general interest magazine not a question in a statistics exam. My guess is that what the questioner really wanted to know was whether it is generally better to swap doors on the show, in other words the unconditional problem That quotation from Seymann's commentry should be written in bold at the top of this article. Martin Hogbin (talk) 21:17, 8 April 2009 (UTC)

[Outindented]The above qouted wording come fom the (more exact) formulation of the problem in the article itself. It is on this formulation I gave - it seems ages ago to me - my reaction. And right I was, as it turns out. There is the original formulation of the question by Whitaker. It is also in the article. The reformulation was merely meant to get rid of the unknown strategy of the host, but it also made the doors (more) explicit. It is an interesting question why some people, who understand (in the mean time) the nature of probability and conditional probability, want to maintain the "simple solution". Maybe it is psychological. Once thought one understood the 2/3 chance instead of the 50/50, one is reluctant to admit it was on false grounds. And hence the problem has to be adapted, as to fit the simple solution. Let us face the practical situation: the player is on stage, she points to door and another is opened. The player knows her situation, and in this situation she has to decide. If we decide for her, we formulate for each possible situation, the decision. Only in our thoughts can we imagine a door is opened, without us knowing which one. In the practical situation it would mean, the player points to a door, Monty opens a door, but the player is blindfolded, so doesn't see which one. Why opening a door if she is not allowed to see it? Is she allowed to use the information, given by opening a door? If yes, she will give a conditional answer like: if door x is opened then ... If she is not allowed to use the information, then don't bother about opening a door. Even don't bring it up, it hasn't happened as far as the player is concerned. Let me finally repeat, that when this whole - in my opinion (POV) too lengthy - discussion started, it was about the more exact formulation in which the door numbers were given. And in my opinion (POV) the problem is only of interest when the player sees the opened door. Nijdam (talk) 06:38, 9 April 2009 (UTC)

The question that you are interested in is the academic version as formulated by Krauss and Wang. That is fine, I have no objection to that but there is another much more notable question, that posed by Whitaker, which is what this article should about. Kraus and Wang have clearly formulated the question to make it unambiguously conditional, the academic question, but that is not the the question that will be of interest to most of our readers. This is what I have been saying all along, there are two distinct classes of ways to formulate the question, neither is any more correct or rigorous than the other, they each interpret the question differently. If the question is formulated as K an W do then it is necessarily conditional, if on the other hand, we take it that the questioner meant to ask an unconditional formulation of the question, or that he did not intend to identify the doors, or that he assumed that the host would act randomly, then the unconditional solution is correct. In the article we should give both solutions with an explanation of which class of formulation each is applicable to. You my be more interested in the academic solution, I am more interested in the popular solution. Let us work together to give excellent examples of each. Martin Hogbin (talk) 08:00, 9 April 2009 (UTC)

Martin - From what I can tell, what you're suggesting is contrary to Dick's intent which is that the popular solution be presented without qualification. He's not arguing that the question is interpreted differently or that the popular solution is "correct", but that the popular solution is the popular solution. This perspective completely avoids any need to talk about exactly what problem those who publish the popular solution think it is solving. We frankly don't care. We don't need to justify its correctness. It is published in reliable sources, so we include it here clearly identified in some way (and attributed to one or more sources that have published it). The job of the article is not to convince anyone that this solution is correct, and whether any of us as individuals think it is or not is completely irrelevant (to the editing process). Discussions about the correctness of what reliable sources say or what the "truth" is generally have no particular place in Wikipedia - they continually come up with regard to this problem which is why there's now a Talk:Monty Hall problem/Arguments page.

Nijdam - ditto the "expert" POV. I've suggested this before, but from an academic viewpoint you should view this article more like a summary of the literature than anything else. We can certainly say "popular sources say this" and "experts say the popular sources are not quite right, and say instead ...", but unlike a literature summary we can't really take a stance. If the article faithfully (neutrally) says what both the popular sources say and what the experts say (including what the experts say about the popular sources) anyone reading the article will be able to draw their own conclusions.

Dick - does this roughly capture what you're trying to do here?

-- Rick Block (talk) 14:04, 9 April 2009 (UTC)

I do not think that Dick is against stating the assumptions under which the popular/unconditional solution is valid and there is no need not to do so. The concept of 'first decide the question' is made clear by the Seymann quote and a clearly unconditional formulation of the problem is given by Morgan (as we have now). I want the unconditional problem not to be seen as the poor relation of the conditional one, it is a problem in its own right and probably the question that Whitaker intended to ask. I think it is important for the unconditional problem to have its own 'aids to understanding' as bringing up the issue of conditionality only complicates the problem further for most people. Martin Hogbin (talk) 17:06, 9 April 2009 (UTC)

If I understand Rick and Martin, I think I agree with both. Dicklyon (talk) 17:37, 9 April 2009 (UTC)

I interpret what Martin is saying is that he wants to "clarify" the problem in such a way as to justify that the popularly presented solutions are correct. In my opinion this would be WP:OR. If we're not going to simply present the expert POV (which I think would be perfectly justifiable - it is a math problem after all, so saying anything other than what the most reliable math sources have to say about it seems kind of silly to me but I understand Dick's NPOV argument), then the popular solution needs to stand by what the popular sources say. They never (as far as I have seen) "clarify" the problem. They generally use a statement like the Parade statement and proceed to say "and the answer is ...". Any clarifications or justifications would need to be sourced. If we're going to go this "it's a POV issue" route, then we need to keep our opinions out of it and say what the sources say. This means no more in the case of the popular solution (specifically, no justifications or clarifications unless these can be sourced), and no less in the case of what the math sources say (specifically, no omitting what they say about the popular sources). -- Rick Block (talk) 18:43, 9 April 2009 (UTC)

Let's Eliminate the "Criticisms of the traditional solution" section

I'm once again returning from the arguments page.

On those pages, particularly the last couple of sections, you will see that the two main opponents to meaningful changes to the article are out of objections. They have no game. It would be almost comical, but I've invested dozens (hundreds?) of hours in this. But, read it and decide for yourself.

I propose we remove the "Criticisms of the traditional solution" section. I'm not going to repeat that section here. It says that in some cases the host revealing a door might provide the player more information...

Of course, that's ridiculous. The Contestant, and anyone else not associated with the program, do not know the original location of the car. Nor does anyone outside of the program know of any host behaviours. Of which none are described in the problem.

Then it says, "Under more general conditions, however, a more general solution is needed (Morgan et al. 1991).". Which, while published, may not have enough merit to discredit the previously provided solution.

I would also like to see the Combining Doors moved from 'Aids to Understanding' to the 'Solutions' section. I think this is far and away the best representation of why it's 1/3 vs 2/3 and not 1/2 vs 1/2. It's already in the article, and has passed muster for 2 or 3 FA reviews.

There may be other statements that negate the validity of the Solution now in place. I'd like to remove all of these as well. Glkanter (talk) 20:21, 8 April 2009 (UTC)

And I would take out the 'equal goat door constraint' from the existing Combining Doors solution. Only 1 of 4 sources mention it, and there is no other mention of host behaviour in the MHP. Glkanter (talk) 20:28, 8 April 2009 (UTC)

Most of my students understood the complete problem and (conditional) solution in no more than 5 minutes. Of course some didn't: they failed the exam.Nijdam (talk) 06:44, 9 April 2009 (UTC)

Maybe a way forwards

Although the details are not yet agreed it is clear that there are two classes of formulation of the problem, to avoid further argument here let me call them the conditional and the unconditional formulations. It is perfectly reasonable to take Whitaker's original question to be asking the unconditional question. On the other most academic sources formulate it conditionally.

Why do we not have two sections, conditional and unconditional, where those interested in that particular formulation take the responsibility for deciding that section's format and structure? Each section should indicate precisely the assumptions on which their solution applies. The normal standards of WP quality should apply to both sections. Martin Hogbin (talk) 08:18, 9 April 2009 (UTC)

We could do that. My suggestion was to segue from the unconditional to the conditional via a short statement that some mathematicians have found the former lacking; I did this with a criticisms section, but it could just as well be an introductory sentence in the conditional solution section. Furthermore, while I'm happy to refer here to the "unconditional" solution, I wouldn't call it that in the article, as the sources that present it as the solution don't generally call it that, and as the term frames it with respect to the other solution; it's really not at all clear what "unconditional" means until you specify what particular events you're not conditioning on, and those particular events don't come up until the conditional section; it's silly. Dicklyon (talk) 14:38, 9 April 2009 (UTC)

I pretty much agree with the above. Provided that there are no statements discrediting the unconditional solution. In that spirit, Dick, what do you think of deleting the Criticisms section immediately? Glkanter (talk) 15:01, 9 April 2009 (UTC)

That's fine by me; consider putting the gist of it into the next section? Dicklyon (talk) 16:12, 9 April 2009 (UTC)

More like 'disappear' it. Take a look at it. Based on all the things I wrote on the Arguments page, I would categorize it as 'horrible'. Glkanter (talk) 16:28, 9 April 2009 (UTC)

As you might expect, I agree with Glkanter that the unconditional section should not be seen as the poor relation of the conditional section. The unconditional formulation is probably what Whitaker actually meant to ask and it certainly is the notable problem. Martin Hogbin (talk) 16:52, 9 April 2009 (UTC)

As long as the formulation of the problem permits the simple solution as a solution it's fine to me. Otherwise it should be discredited as being incomplete or even wrong. Nijdam (talk) 19:04, 9 April 2009 (UTC)

Yes, of course. Martin Hogbin (talk) 21:11, 9 April 2009 (UTC)

The key to peaceful coexistence seems to be to find a way to segue into conditional probabilities without implying that the simple solution lacks something that it ought to have. Here are two suggestions for the transition:

Remark that analysis via conditional probabilities allow generalization to related but different problems, such as if we have knowledge of Monty's bias towards different doors. Then show how the c.p. analysis gives the same result as the simple analysis, and afterwards briefly discuss other possibilities.
Note that the first solution is simple and clever, but requires a creative insight (namely, that under appropriate assumptions it is irrelevant whether you decide before or after being given the choice). What follows is a less creative argument for the same answer that does things by the book without clever shortcuts: (bla bla bla)

The second one is the one I like best, but might not be acceptable to the conditional-probabilities camp. –Henning Makholm (talk) 22:56, 9 April 2009 (UTC)

Sorry, I don't care for either. Maybe there is no segue. Rather, an intro that says here are two different techniques, both published. Then present them. Glkanter (talk) 23:12, 9 April 2009 (UTC)

Your contribution would be more helpful if you explained what you think is wrong with the proposal rather than just rejecting them. –Henning Makholm (talk) 23:26, 9 April 2009 (UTC)

For #1, I don't believe there is such a thing as knowledge of Monty's bias. Not outside the show's production team, anyways. (see the Arguments page). #2, I disagree with your characterization. Because the problem is simple, the solution is simple. And there's no shortcuts. There's just no extraneous bs. Glkanter (talk) 23:53, 9 April 2009 (UTC)

G, while I sympathize with the POV that the simple solution is all that's needed, it's not all that's out there. We need to report the other interpretation and why people use the conditional approach; and do so in their terms, not ours. I agree that a contestant isn't likely to have a model of Monty's bias, and that it's peculiar to interpret the problem as if she has, nevertheless, it's what they do, and they publish in top journals, so it should get good coverage. It just shouldn't trump the simple POV. Dicklyon (talk) 00:30, 10 April 2009 (UTC)

I agree that the Morgan pov is published and deserves some mention. To help determine how much, let's talk about the Contestant's knowledge of a host bias. First, there's no such item mentioned until Morgan. Second, the only reasonable presumption is that once the bias is created, the Contestant becomes aware of it. So, it becomes a new premise, making it a new puzzle. Check out Rick Block's 'forgetful host' bias on the Arguments page. It's really "Deal or No Deal". And, three out of the 5 premises change. How is that relevant to the MHP? Lastly, how did the Contestant become aware of any such bias? Does the production team tell him? For what purpose? Heck, in the US that would be against the law. So, yes, it's published. Does it make much sense? Not to me. Not at all. So, mention it if we must, but severely limit it's prominence. Glkanter (talk) 00:47, 10 April 2009 (UTC)

Neither sounded great to me, either, but I'd have to see them done out. What matters most to me is that the statements be sourced. If Morgan or someone uses a statement like one or the other of these, and we can attribute it him, or someone else, it will be OK. But since they both sounded like something you made up, it's hard to say yet. Can we follow a source? Dicklyon (talk) 23:45, 9 April 2009 (UTC)

I like the concept involved in Henning's option 2 in that it extends the applicability of the unconditional solution to special conditional cases. As the unconditional solution is the really notable one I think the more we make of it the better.

To avoid confusion we should have two sub-sections in the 'unconditional' section. The first treats the problem strictly unconditionally and the second considers intuitive extensions of the treatment to cover the appropriate conditional cases. This then naturally leads to further discussion of the more general conditional case.

On thing I would also like to clear up, probably on the arguments page, is the matter of problem style. I am not an expert on statistics but it seems to me that there are various styles in which a question can be posed. In the formal style we are given a problem, to be answered only on the information given in the question; what any of the characters in the problem may or may not know is irrelevant. The more traditional style is to pose the question from a particular point of view or state of knowledge of an individual involved in the problem itself. Common statements of the 'three prisoners' problem are good examples of this approach. Would somebody who is an expert on the subject like to comment on my understanding, either here or on the arguments page. I think much of the argument about this topic results from failing to adequately make the above distinction. Martin Hogbin (talk) 10:28, 10 April 2009 (UTC)

Is there anyone arguing we should keep the "Criticisms of the popular solution" section? Like I suggested above, if we move the first three sentences (up to but not including "Nevertheless ...") to the "Popular solution" section I think the rest is covered adequately in the current "Conditional solution" section. -- Rick Block (talk) 02:46, 10 April 2009 (UTC)

I'm OK getting rid of the section and moving the content; however, the first sentence is really Morgan et al.'s criticism and narrowing of the solution, and doesn't belong as part of the solution; but it can be modified to work, I think. You want me to work on it? Dicklyon (talk) 04:11, 10 April 2009 (UTC)

I'm mostly just checking to make sure no one is going to complain if the section is deleted. We'll need to talk about the specific content at some point (completing deleting the first sentence for now would be OK with me, but it's the lead-in to the second sentence so ...), but first things first. -- Rick Block (talk) 04:43, 10 April 2009 (UTC)

I think it is best to leave the lead until last. As you have said, it should be a summary of the article as a whole. In that case it might as well be left as it is with the intention of rewriting it when the article changes have been completed, but I do not care much either way. Martin Hogbin (talk) 10:04, 10 April 2009 (UTC)

I have changed the intro to the popular solution section to show it as a correct solution to a different formulation. Is everyone OK with this? Martin Hogbin (talk) 16:10, 10 April 2009 (UTC)

Absolutely not. In my opinion, the problem statement you've included is not anyone's usual understanding of the Monty Hall problem. If you want to claim that it is, I respectfully request that you provide a reference to some published source that actually says it is common to interpret the problem this way. Without a source that says something like "this is how the problem is usually interpreted" (which, BTW, is the exact opposite of what Morgan et al. say - so using them to justify this problem statement is perverse) this claim is simply your own WP:OR. -- Rick Block (talk) 18:40, 10 April 2009 (UTC)

Morgan refer to this statement as 'the unconditional problem' . They also say in their conclusions that 'The unconditional problem is of interest too...'. The statement that I quoted obviously is a possible interpretation of the problem and Morgan say that it is of interest, what more do I need? Martin Hogbin (talk) 19:25, 10 April 2009 (UTC)

I agree with Rick here; Morgan's "unconditional" framing is not relevant to the popular solution. Let's just leave it out until we talk about conditional. Dicklyon (talk) 05:12, 11 April 2009 (UTC)

My suggestion, which seems to have some support, is for the unconditional solution to stand in its own right as a solution to the unconditional problem (and special conditional cases). In order to do this we need a statement of the unconditional problem and it would be best to use one from a reliable source. Morgan have just such a statement and I do not see why we cannot use it, purely as a published statement of the unconditional problem. We do not have to suggest that Morgan themselves interpret the problem unconditionally. Alternatively, does anyone know of another unconditional problem statement that we could use? Martin Hogbin (talk) 09:24, 11 April 2009 (UTC)

How about we delete the 4th paragraph of the Sources of Confusion section?

Here's what it says:

"Another source of confusion is that the usual wording of the problem statement asks about the conditional probability of winning given which door is opened by the host, as opposed to the overall or unconditional probability. These are mathematically different questions and can have different answers depending on how the host chooses which door to open if the player's initial choice is the car (Morgan et al., 1991; Gillman 1992). For example, if the host opens Door 3 whenever possible then the probability of winning by switching for players initially choosing Door 1 is 2/3 overall, but only 1/2 if the host opens Door 3. In its usual form the problem statement does not specify this detail of the host's behavior, making the answer that switching wins the car with probability 2/3 mathematically unjustified. Many commonly presented solutions address the unconditional probability, ignoring which door the host opens; Morgan et al. call these "false solutions" (1991)."

This is just another Morgan pov. And it adds a non-existent premise, so it's not even addressing the MHP. Glkanter (talk) 10:16, 10 April 2009 (UTC)

I have started to address the point you (we) make. I have added a quotation from the Seymann comment at the end of the Morgan paper. No one seems to have objected to this, which does not surprise me as it is a quote from a reliable source making a point that cannot really be contested. Martin Hogbin (talk) 10:45, 10 April 2009 (UTC)

You might like to look at my above remark about question style. I believe that there are two styles of asking probability problems which must be approached in different ways. I am waiting for someone who knows about such things to confirm this as I believe this fact may be responsible for much of the argument here. Martin Hogbin (talk) 10:48, 10 April 2009 (UTC)

Oh sure, I read it. This issue came up earlier this week. It immediately became muddled, so I started using the phrase "anyone not associated with the production of the show". That covers the Contestant and all of us 'observers'. I think it only arises with 'host behaviour', which is a euphemism for 'new premise' (and, therefore, a different problem). And 'host behaviour' doesn't exist in the MHP, beyond revealing a goat and always offering the switch. Please, don't get me started. BTW, do you agree with my suggested deletion? Glkanter (talk) 11:06, 10 April 2009 (UTC)

I think the whole article needs to be restructured to give at least equal prominence to the 'unconditional' formulation. This would include splitting the 'Sources of confusion' section in two, one to deal exclusively with the 'unconditional' problem. AS it is now it only adds more confusion. How to start this process, if everyone agrees, I do not know.

I think you should discuss the question style issue but probably on the arguments page. I believe that many of the arguments her come from mixing the two styles. Martin Hogbin (talk) 11:23, 10 April 2009 (UTC)

I'm not much interested in beginning that discussion. I think it's just one of the various obfuscation techniques.

Quite the reverse. I am trying to clarify things. Once you understand how formal probability problems are treated you can see how the trick is done. Martin Hogbin (talk) 14:55, 10 April 2009 (UTC)

I just read your new section. Very interesting, very well written. I hope you're right, that this will help to properly define the problem, in order that it can be properly solved. You have much more patience than I do. I've been at this almost 6 months (although I took a two month break), and I dread the thought of this new discussion with the same cast of editors. I'll probably sit it out. But, if it codifies that new premises make new problems, that alleged 'host behaviours' are new premises, and that for this Wikipedia article we're only discussing the MHP, well, then maybe it's worth it. But it's still a shame that it's necessary. What about deleting the 4th paragraph of the Sources of Confusion section? Glkanter (talk) 15:58, 10 April 2009 (UTC)

Thank you for your support, there have been no serious challenges to what I have said so far. Regarding sources of confusion, I believe that it is essential to have a separate section for the unconditional/traditional/simple solution before we can address this point and there seems to be something of a consensus to do this. There is plenty of confusion about the unconditional problem, that is why the problem is notable, and it does nothing to reduce this confusion to discuss the conditional problem. There is plenty to say about the conditional problem also but that should be said in a separate section. Martin Hogbin (talk) 09:17, 11 April 2009 (UTC)

In light of the fact that there have already been 37 edits to the article in April, I think the process has already started. You've mentioned numerous times that neither of the competing approaches can claim sole bragging rights. In that vein, I'd like to remove all the statements that 'weaken' the unconditional solution. Glkanter (talk) 11:48, 10 April 2009 (UTC)

I disagree with the suggestion to delete this paragraph. It's impeccably sourced and is one of the most significant points in the entire article. Would anyone prefer the "The distinction between the conditional and unconditional situations here seems to confound many" to the "false solutions" quote? You two have both amply demonstrated how profoundly confounded you are, so let's all just assume you violently disagree with this and let others weigh in with their opinions. -- Rick Block (talk) 13:31, 10 April 2009 (UTC)

The accusation that I am profoundly confused is rude and unjustified. I have demonstrated on the arguments page that I fully understand the issue of conditional probability. Perhaps you should continue with discussion of the subject matter rather that try to make your point by attacking editors who disagree with you. Martin Hogbin (talk) 22:05, 11 April 2009 (UTC)

I agree with Rick on this, too. However, it might make sense to make it very clear that this is Morgan et al's interpretation; if we state "the usual wording of the problem statement asks about the conditional probability" as a fact verifiable by Morgan, as opposed to an opinion thereof, that should be fixed, or made more clear. Dicklyon (talk) 05:15, 11 April 2009 (UTC)

Now that I look at, though, it's really not clear what to report, or whether it belongs in the "sources of confusion" section. Rick, can you give us a quote or two? Does Morgan reall refer to this as a source of confusion? Or is it just his statement of the problem he's solving? Same with Gilman. Do they present it the same way? Can we rephrase it properly attributed as their interpretations and/or opinions, instead of as a statement that's verifiable in their papers? Dicklyon (talk) 05:25, 11 April 2009 (UTC)

If Morgan is right, that the unconditional solution is a 'false solution', how does he explain the experts that have used it subsequent to his criticism? Like Devlin, for example. http://www.maa.org/devlin/devlin_07_03.html Devlin seems pretty aware of the MHP's history. I wonder why he didn't mention Morgan's 'equal goat door constraint'? It should be his duty, yes? My guess? To the professional community, Morgan is like the crazy old uncle hidden in your attic. Glkanter (talk) 22:54, 11 April 2009 (UTC)

Who knows? Maybe Devlin feels that Morgan's approach is overly pedantic and not worth mentioning. Or maybe he's unaware of it. Either way, so what? Dicklyon (talk) 01:33, 12 April 2009 (UTC)

In this particular instance, I guess my only point is that as a 'Source of Confusion' the article includes Morgan's opinion that the unconditional solution is a 'false solution'. That would seem to be contradicted by Devlin's (and others') use of it as their sole method, subsequent to Morgan's paper.

In the larger scheme of things, it's been stated repeatedly by the Morganians that absent any direct published criticism of Morgan, his is the final word. I'm just showing that there have been unconditional solutions published since Morgan, which, while not a full on smack-down, show disagreement with his conclusions. I presume it would be unseemly for these professionals to get into a public argument, so as you suggest, they ignore him. I wish we could do the same with the article. Glkanter (talk) 02:03, 12 April 2009 (UTC)

It's no problem reporting different points of view, even if they're mutual contradictory, without deeming one final. We can report the "source of confusion" if Morgan called it that. What worries me here though is that Morgan didn't call it that, and that it's really an editor's interpretation to call it a source of confusion. Seems to me I had a copy of Morgan, but now I can't find it. Does anyone have one they can send me? Dicklyon (talk) 02:58, 12 April 2009 (UTC)

I'm traveling and my participation will be somewhat limited over the next week or so. Between the archives of this page and the /Arguments page, I think most of the relevant passages from the Morgan et al. paper have already been quoted. The main points are:

1. The usual statement of the problem (such as the one from Parade) asks about the conditional probability of winning by switching given that the host has opened a particular door.

2. The popular solutions do not address this question but address a different question (e.g. the success of a pre-selected "switch strategy")

3. The answers to these questions can be different unless the host is constrained to open a randomly selected door in the case the player's initially selected door hides the car.

A quote from the paper concerning what they label as "F1" ("false solution" #1) is at Talk:Monty_Hall_problem/Archive_9#Wrong explanation. Note in particular the phrase "The distinction between the conditional and unconditional situations here seems to confound many". So, yes, they're directly saying this is a source of confusion. Gillman's comments are similarly quoted in the same thread in the archive. The Grinstead and Snell book says something similar as well (it's freely available online). -- Rick Block (talk) 05:17, 12 April 2009 (UTC)

Thanks, Rick, that's very helpful. I've added that brief quote from that quote into the relevant paragraph in the article, and rephrased it to more clearly attribute the interpretation to these authors. When you get a chance, let me know if that seems OK. I believe it is much better than the previous statement that a "source of confusion is that the usual wording of the problem statement asks about the conditional probability," which is not really verifiable as a fact. Dicklyon (talk) 06:08, 12 April 2009 (UTC)

I think I agree and understand their main points that you summarize above, except that the "other question" doesn't really require the player to decide on a strategy before a door is revealed; it really only requires that the player's decision not be influenced by which door is opened, right? For example, a player new to the game may not know that she's going to be offered a chance to switch until after a door is opened and the offer is made; then all the rules and such having been explained, she can choose to switch or not; having no statistical model of which door the host might open when given a choice, she might sensibly ignore that bit of information and still solve the unconditional problem, yes? So why are still guys (or Gillman at least) so insistent on the bold before a door has been opened? Dicklyon (talk) 06:08, 12 April 2009 (UTC)

From my standpoint, this hasn't gotten any better.

"That is, they, and some others, interpret the usual wording of the problem statement as asking about the conditional probability of winning given which door is opened by the host, as opposed to the overall or unconditional probability. These are mathematically different questions and can have different answers depending on how the host chooses which door to open when the player's initial choice is the car (Morgan et al., 1991; Gillman 1992). For example, if the host opens Door 3 whenever possible then the probability of winning by switching for players initially choosing Door 1 is 2/3 overall, but only 1/2 if the host opens Door 3."

Just once, I'd like to see Morgan's POV made without giving a host behaviour. Because I believe the act of giving a host behaviour makes it a different problem, and no longer the MHP. Without a host behaviour, it 'averages 2/3'. Unconditionally, it is 2/3.

And this is clearly Morgan's POV: "In its usual form the problem statement does not specify this detail of the host's behavior, making the answer that switching wins the car with probability 2/3 mathematically unjustified. Many commonly presented solutions address the unconditional probability, ignoring which door the host opens; Morgan et al. call these "false solutions" (1991)." For example, Devlin says it's unconditionally 2/3. Is Devlin guilty of providing a 'false solution'?

This would, at best, belong in a section explaining why there are two solution techniques provided, as per Martin's suggestion. Glkanter (talk) 07:00, 12 April 2009 (UTC)

I don't understand; how can Morgan's POV be explained without reference to a model of the host behavior? The host behavior is what he's talking about conditioning on and exploiting a probabilistic model of. Are you just saying it's in the wrong place in the article? That the "confounding" concept is relevant only to one problem interpretation? I think that's clear now, but maybe not. Dicklyon (talk) 15:11, 12 April 2009 (UTC)

Imho, Morgan is OK to say "because no host behaviour has been described as a premise, using the conditional solution can only provide a result that in any one instance is between 1/2 and 1, and averages 2/3". But the moment he says, 'take, for instance, the following behaviour...', he is adding a premise regarding the hosts behaviour. And still undecided is whether the Contestant, or whoever's 'state of knowledge' we're talking about, knows of this. To me, that is a new problem. Did you see in the arguments page where 'the forgetful host' behaviour actually turned out to be "Deal or No Deal?" 3 out of 5 'agreed upon' premises changed. That makes it a different problem. This is what they taught in my college. I assumed that was a universal principal.

This is not a 'Source of Confusion' for anyone who is using the unconditional interpretation and solution. It is the reason for having the two different interpretations and solutions, as proposed by Martin. I'm suggesting that a section explaining why there are two solutions is the only appropriate place for this criticism. Glkanter (talk) 19:00, 12 April 2009 (UTC)

I agree that it would make more sense in a section about the conditional problem; it's not a relevant confusion about the usual understanding of the problem. Dicklyon (talk) 02:57, 13 April 2009 (UTC)

Based on your comment above, I didn't expect your reversion. Would you prefer I create the section called 'Why Two Solution Techniques Are Provided' and put it there? Glkanter (talk) 18:45, 13 April 2009 (UTC)

It needs to go someplace. I'm not sure a new section is the right idea. Dicklyon (talk) 19:25, 13 April 2009 (UTC)

Well, maybe it needs to go someplace else. Because it doesn't belong in the Sources of Confusion. Glkanter (talk) 22:06, 13 April 2009 (UTC)

This gets back to what problem are we solving? In nearly all phrasings of the problem the player chooses a door, then the host opens a door, and then the player is asked whether she wants to switch and we're asked to think about the probability of winning by switching. This much makes it a conditional probability problem (and calling this a "POV" seems like kind of a stretch - it's more like a simple fact that most people are unaware of). The "unconditional" solutions ignore which door the host opens, which produces the correct answer for this question if and only if the host opens one of the remaining doors with equal probability in the case the player has initially selected the car. If we're asking about the probability of winning by switching after the host has opened a door, as a math problem the host's preference in this case (where the player has initially picked the car) always affects the answer. In the Parade version of the problem the host's preference was not specified - which makes it unknown, rather than 1/2. If it is, say q, then the chances of winning by switching are 1/(1+q), i.e. somewhere between 1/2 and 1 (not simply "2/3", as it is when q is 1/2). Morgan (and Gillman, and others) are so insistent about this because "solving" this problem unconditionally introduces an assumption that was not in the Parade version of the problem statement (which is the version they both discuss). Their POV is that anyone approaching the problem unconditionally is confused about the nature of the problem and (for the Parade version) is introducing an assumption that is not stated. -- Rick Block (talk) 14:05, 14 April 2009 (UTC)

Dicklyon, is it OK to delete the 4th paragraph now? Glkanter (talk) 11:06, 18 April 2009 (UTC)

It is not OK with me. -- Rick Block (talk) 16:57, 18 April 2009 (UTC)

I agree it's not OK to delete it. It represents, as Rick says, the POV that "that anyone approaching the problem unconditionally is confused". However, that's not a confusion about the solution, but about what the problem is, and it's probably a minority POV, so probably belongs more in the conditional section, or a transition to that section, rather than in a place were it appears to be trying to say something about why people are confused in their analysis of the more common interpretation of the problem. Dicklyon (talk) 17:36, 18 April 2009 (UTC)

Congrats

Congrats on the FA; I read it and this article is a very interesting read! However, I have one problem with it. Shouldn't the article use inline citations? ResMar 22:39, 12 April 2009 (UTC)

It uses the alternative WP:HARVARD style. Dicklyon (talk) 23:41, 12 April 2009 (UTC)

Why does the Conditional Solution begin with the non-existent 'equal goat door constraint?

As I've been claiming, this, or any host behaviour is a new premise which makes it not the MHP. It seems to me that the Conditional Solution should demonstrate that the answer is between 1/2 and 1, and averages 2/3. Glkanter (talk) 23:36, 12 April 2009 (UTC)

In fact, the entire 2nd paragraph, the tree, and the concluding diagram all rely on the 'equal goat door constraint'. All this does is provide a contrivance where the result is forced to 2/3. Why choose this particular host behaviour? (Not that there should be ANY host behaviour.) Why not show the 'left most door constraint', or the 'host forgets constraint' (aka Deal or No Deal). And maybe this section should include an explanation of how any of these behaviours give the Contestant any knowledge of where the car is? Since they don't, despite what the opening paragraph says. Glkanter (talk) 23:47, 12 April 2009 (UTC)

If the player has a probabilistic model of the host's behavior, the door opened can give the player some info about where the car might be, different from in the unconditional case if the probabilities are biased. I think they do the equal probs first to show that it gets the same solution to the what's effectively the same problem. Dicklyon (talk) 00:02, 13 April 2009 (UTC)

But where did the Contestant get it from? It simply doesn't exist without adding a host behaviour premise of some type. (Plus a mechanism for transferring this model into the Contestant's brain. Is this collusion? ESP, perhaps?) Doesn't a new premise make it a different problem than the MHP? One that coincidentally (or not?) has the same result? Isn't this the Morganians entire point on why the unconditional is a 'false solution'? Can't the Morganians make their point without adding unsupported premises? Is it common to give a solution to one problem using a different problem? I'm not familiar with this technique. Glkanter (talk) 00:58, 13 April 2009 (UTC)

Well, I agree with you that's it's far fetched as an interpretation of the intended problem, and not likely practical in reality, but that's not their point, is it? The Morganians point is that if you can model the probabilistic behavior of the host you can determine the probabilities in a given situation (a given door having been opened), and that if you can't model it then you can't really compute your probability of winning. As I read it, the original problem only asked if you should switch, so you can certainly interpret this as working a different problem; so is providing the 2/3 answer from the unconditional method; the only answer really called for is that you should always switch, and even that one needs more assumptions than are sometimes stated in the problem. Dicklyon (talk) 01:32, 13 April 2009 (UTC)

Do you agree that adding premises makes it a new problem? Would that make it unfit as a solution? Not a Wikipedia "it's published" solution, but as a valid mathematical solution. Glkanter (talk) 01:50, 13 April 2009 (UTC)

It's not up to me. We should report what's in the literature. Dicklyon (talk) 01:58, 13 April 2009 (UTC)

You can't say whether, in your opinion, you consider it a valid mathematical solution? Why not? Glkanter (talk) 02:16, 13 April 2009 (UTC)

It is mathematically valid; I don't think that requires any opinion. As to whether it addresses the stated problem, I have no opinion worth discussing. Dicklyon (talk) 02:18, 13 April 2009 (UTC)

Ouch! There were two parts to that question. Does adding a premise make it a different problem? Glkanter (talk) 02:53, 13 April 2009 (UTC)

Is it just me, or is this as tedious and irrelevent a nitpick as saying, "Since the problem does not explicitly specify which way up the host is, we must also consider the possibility that he is standing on his head for the entire game" ? Honestly, what kind of person, apart from a mathematician who has already studied the problem and knows to think about it, would hear the problem as (originally) stated and not assume the host will choose one of the two remaining doors with equal probability? For that matter, what kind of foolish assertion is "any similar problem in which the (inexplicably necessary, for some people) host behaviour is specified is a totally different problem distinct from the entire idea of the Monty Hall Problem" ? Come on. This is just silliness, right? Maelin (Talk | Contribs) 05:39, 19 April 2009 (UTC)

What's silly is that we don't just report what's out there, including those points of view that we disagree with. Then we could get the article done up well without squabbling over such nits. Dicklyon (talk) 05:46, 19 April 2009 (UTC)

These discussions are very stimulating to a mathematician! Inspired by them, I have now written two short notes on the Monty Hall problem, plan to merge and rewrite and submit to a reputable journal. I hope that especially the second note might clarify some points. Links: Gill's first attempt, including novel game theoretic approach; Gill's second attempt, comparison of conditional and unconditional approaches. I try to see what can be derived with what assumptions, ideally a mathematician comes up with "necessary and sufficient conditions": "If you do assume X, then Y is true; if you don't assume X, then Y is not true". Nearly there, I think. Gill110951 (talk) 13:16, 21 April 2009 (UTC)

@Gill: I read your second attempt. Lookes fine to me, and it describes in a plain mathematical way the problem and the analysis. What remains is the connection of the problem in words and the math. Will you try? BTW: your remark above about "necessary and sufficient conditions" puzzles me. Nijdam (talk) 16:16, 21 April 2009 (UTC)

@Nijdam: I am glad you liked the second note. I was hoping that someone who understands my maths and likes the solution, would themselves build the word-bridge from the original problem formulation(s) to the mathematical analysis. I may do it myself one day, when I work those pdf's into a real paper and offer it for publication somewhere, but it is not going to be very soon.

Concerning necessary and sufficient conditions, I mean the following. Let's agree that conditional and unconditional Monty-Hall problem can be formulated in terms of a sequence of three probability distributions: the quiz-team's choice of door, the player's choice of door, the quizmaster's choice of door given the preceding two. Let's agree that the quizmaster must open a door revealing a goat. Let's agree that the quizteam's and the player's choice are (statistically) independent of one another. Then everything depends on 1) three probabilities, adding to 1, of the original location of the car; 2) three probabilities, adding to 1, of the player's choice; and 3) for each of three possible locations of the car, two conditional probabilities, adding to 1, that the quizmaster will open each of the other two doors with goats behind them given that player and quiz-team have both chosen the same, particular, door. Taking account of the constraints that probabilities add to one, it follows that by specifying exactly (3-1)+(3-1)+(2-1)+(2-1)+(2-1)=7 input probabilities, everything else is determined, including the output probabilities of interest. Certain combinations of values of those 7 input probabilities imply that Prob(win|switch)=2/3. Other combinations imply that Prob(car is behind 3|player chose 1 and QM opens 2) is greater than or equal to 1/2. Yet other combinations imply that Prob(car is behind door x|player chose y, QM chose z) is at least 1/2 for all of the six possible values of the triple (x,y,z). So far, everyone talks about sufficient conditions: that is, (preferably nice/attractive/intuitively meaningful) conditions on the input probabilities, which imply a desired property of an output probability. Looking for necessary conditions is going the other way: suppose I tell you that it is never disadvantageous to switch, ie all six conditional probabilities of the other door being the good door are at least 0.5, what can we deduce about the 7 input probabilities? Can we say something nice/meaningful about them? I don't think anyone has every looked at the problem in this way before, just as it seems no-one ever asked themselves what game theory has to say about this game. Gill110951 (talk) 16:17, 26 April 2009 (UTC)

Ok, if that's what you mean, I understand. One remark: to be complete, you should introduce conditional probabilities, not only depending on the position of the car, but also on the choice of the door by the player. BTW: I'm not an expert on game theory, so I have difficulty in understanding that part of your paper, although I would like to see the connection with the probabilistic approach. Yet I'm familiar with the basic concepts of game theory, that's why I think you might change the wording as to make it understandable for the lesser expert. Nijdam (talk) 20:02, 26 April 2009 (UTC)

Aids to Understanding

The "Aids to Understanding" section should be revised. The fourth sub-section includes no references. The first sub-section includes one reference, but the rest of what is written is not an illustration or explication of the referenced point. The second and third sub-sections are relevant, referenced descriptions of explanations that have been presented as aids in understanding why the best answer to the posed question is not "No." (The posed question is "Is it to your advantage to switch your choice?") The first and fourth sub-sections should be eliminated, and the section title or an introduction to the section should imply that the subject matter of each remaining sub-section is a suggested aid to understanding. This isn't a "how-to" magazine article. —Preceding unsigned comment added by 74.248.220.132 (talk) 03:30, 19 April 2009 (UTC)

The first thing that needs to happen to this section is that it needs to be split into two parts, the first dealing with the simple, notable, 'real', Monty Hall problem, which is one of the most unintuitive and notorious probability problems known, and the academic complications to the problem which are less notable but many may find interesting. Martin Hogbin (talk) 09:50, 19 April 2009 (UTC)

Perhaps many things about this puzzle should be split into two parts. Why not let's start by splitting the unreferenced parts that should be eliminated from the referenced parts that might (or might not) be worth keeping? And seriously, the "real" Monty Hall problem might be all kinds of things. GameSetPoint (talk) 02:57, 21 April 2009 (UTC)

Proposed new section - Problem formulations and approaches

I propose that we add a section on the above subject immediately after the 'Problem' section but before the various solutions. This should make clear some of the way the problem can be interpreted and formulated and the correct approach to a solution in each case.

Krauss and Wang (I strongly suggest that anyone interested in this article reads their paper) make the following points:

Most people interpret the problem as their mathematically explicit version (this is already in the section above).

'No other statistical puzzle comes so close to fooling all the people all the time'. [Referring to vos Savant's formulation and solution]

The conditional solution is only relevant in the case that the door opened by the host is identified in the problem formulation. They say:

'As demonstrated different assumptions about Monty Hall's strategy lead to different Bayesian solutions [referring to their version of Morgan's calculation].... The advantage of the no-door and the one-door scenarios, in which Monty hall's behaviour is not specified, is that participants do not need to consider the possible strategies that Monty Hall might use '. [The one-door scenario refers to the case where the door opened by Monty is not specified.]

This last point is one that I have been making all along. I have to admit that I deleted a section that may have been trying to make this point but it made it so badly that I did not understand the point bing made. We need to make clear that this distinction is based on a reasonable interpretation of the question not mathematical sloppiness. Martin Hogbin (talk) 10:41, 19 April 2009 (UTC)

That sounds like a good idea. Contrasting the interpretations of the problem will make the presentation of solutions much more clear and simple, I think. But it has to be presented with a good neutral balanced presentation of the alternative views on what the problem is. Can you draft that? Dicklyon (talk) 16:09, 19 April 2009 (UTC)

TeaDrinker deleted my change following the "opening more doors" ilustration, as follows and asked for comment: "Marilyn’s illustration based on a million doors (where all doors are eliminated but two – Door 1 (which had one in a million chance of having a car) plus one of the other 999,999 doors (which either has the car, 999,999 out of a million times, or one time in a million has a goat) is powerful but misleading. This assumes that the original problem calls for Monty to reveal ALL remaining goats except (possibly) one. The problem could just as easily be looked at as Monty revealing just ONE goat from behind Doors 2 through 1,000,000. If only one of the 999,999 doors were revealed to have a goat, and you had to choose between keeping Door 1 or choosing any one of the 999,998 other remaining doors, your odds were still be better if you switched to one of the 999,998 doors, but only very slightly better." TeaDrinker admitted that my point was valid but suggested that this does not explain why my odds are still better even if I have to choose from 999,998 remaining doors. But the point was not that I disagree with Marilyn, rather that for her dramatic illustration of the correct answer she may have smuggled in an unwarranted assumption about Monty's task.83.5.134.48 (talk) 09:09, 20 April 2009 (UTC)

I agree with Hogbin & others (I think!) and believe that the current page could be reorganized to be more helpful. Specifically, the classic problem should be stated in isolated, simple terms, although spelling out some of the main assumptions that were not spelled out originally. Mainly these are (1) Monty knows where the car is, (2) Monty always offers the choice (he's not playing a head game, deciding to offer a choice based on private, perhaps spiteful reasons, e.g. mostly when someone has in fact chosen the car and he wants to 'cheat' them out of it - even if this would be more fun on a real show! (and necessary if people were familiar with the problem beforehand and therefore would always switch when given a choice)), and (3) if both remaining doors conceal goats, Monty's choice of which goat to show is random. Then the solution(s) to the classic problem should be stated. Only later should variations in the problem itself be presented. In my view the so-called "conditional solution" as presented here is not really a solution to the classic problem but rather a discussion of certain variations on the classic problem and thus muddies the waters. It should be moved to the variations section. (For example, if both doors have goats, does Monty have a rule that he must open a certain door and not the other one? That would give away the solution every time, etc. etc.) When the classic problem is properly presented, it is easier to concede that (telescoping the illusory motions), the player is effectively allowed from the outset to choose either just Door 1, or the better of Doors 2 and 3. That said, could there be feedback on this before (or a lack thereof) so one could safely make a change without some good soul like TeaDrinker (greetings) just deleting it? (There must be a game theory to that, too.)83.5.134.48 (talk) 13:12, 20 April 2009 (UTC)

The question we keep running up against is what is the probability question that is being asked. I think there are two main interpretations:

1) What is the probability of winning by switching, averaged across all players?

2) What is the probability of winning by switching for a player deciding to switch after the host opens a door, this player looking at two closed doors and one open door showing a goat?

The math sources ALL say the question is #2 and that this is a conditional probability question. Perhaps the popular sources understand that these are different questions, or perhaps not. Solving a conditional problem with an unconditional approach works, but only under certain assumptions. Rather than stand on our heads trying to force an unconditional solution to "work" I think what we should really do is present a conditional solution and then follow this up with a discussion of the "popular" (unconditional) solutions, specifically mentioning that they are valid only for a very constrained version of the problem or a version that asks about the probability averaged across all players.

The issue with the unconditional approaches is that it leads the reader to think that these approaches are generally correct ("the host opening a door doesn't affect the player's initial 1/3 chance", or "the combined probabilities of the unchosen doors must always be 2/3", or even "dividing the cases by where the car is initially placed results in a 1/3:1/3:1/3 distribution that is unaffected by the host opening a door"). In a fairly recent column, vos Savant addresses the "host forgets" variant (exactly the same as the classic MHP, but the host forgot which door the car is behind and opens a door revealing a goat accidentally). In her analysis of this version she laments [4] "Back in 1990, everyone was convinced that it didn’t help to switch, whether the host opened a losing door on purpose or not. ... Now everyone is convinced that it always helps to switch, regardless of what the host knows. But this is just as incorrect!" This is absolutely true. And, IMO, it's precisely because the popular sources do NOT address the "classic" MHP as a conditional probability problem. As a featured article on Wikipedia, IMO this article must not make the same mistake. -- Rick Block (talk) 13:53, 20 April 2009 (UTC)

Rick, where is the evidence that any of the "popular" solutions are addressing your problem number 1? I don't see them interpreting the problem that way (though the simulations do address it that way, effectively, in some cases). Dicklyon (talk) 14:36, 20 April 2009 (UTC)

I'm not saying the popular solutions address #1 (and since they don't say we can't really tell). On the other hand, we do know (and I don't think this is a POV issue) that the mathematically precise way to solve a conditional probability problem is to treat it as a conditional probability problem and that the popular solutions do not do this. What I'm suggesting is the main solution in the article be a conditional solution, presented as comprehensibly as we can. And then, perhaps in the "aids to understanding" section, present one or more of the popular solutions with some discussion about why they work only in the case where the host is constrained to pick randomly if the player has initially selected the car. I crafted a conditional solution in the same general style as vos Savant's wording for the "host forgets" variant in a thread on the Talk:Monty Hall problem/Arguments page. Here's a slightly different version:

Here’s one way to look at it. Some of the time, the host will open door 2. In our puzzle, that didn’t occur. So we’re considering only the times when either: 1) You have chosen the door with the prize and the host has opened door 3 - and if the host opens door 2 and door 3 each half the time when you've initially chosen the car, your original 1/3 chance is divided in half and is now 1/6; or 2) The prize is behind the unopened door, which it is with probability 1/3 (in which case the host is forced to open door 3). The car is behind the unopened door one-third of the time but behind your door only 1/6 of the time, so your chances of getting the car double by switching.

The "host forgets" variant is one example of very minor variants where the unconditional approaches result in the wrong answer for reasons which aren't exactly obvious - e.g. how does the host forgetting where the car is change the original 1/3 probability of having selected the car?. The answer is it doesn't, but that's not the same as the conditional probability after the host has opened a door. In explaining this version, vos Savant treats it as a conditional probability problem. -- Rick Block (talk) 19:08, 20 April 2009 (UTC)

The above paragraph grossly mis-states vos Savants's discussion. The 'host forgets' is actually 'the host chooses among the 2 remaining doors randomly'. Which means, sometimes he reveals the car, ending the game. And three of the five 'agreed' premises change. It's not a 'minor' change. Here's vos Savant's link, see for yourself: http://www.parade.com/articles/web_exclusives/2007/02-04-2007/Marilyn-Readers-Respond . Since it's a random choice, it could be the contestant revealing (the) subsequent door(s). Just like Deal or No Deal! Here's where this fiction was most recently brought up. http://wiki.riteme.site/wiki/Talk:Monty_Hall_problem/Arguments#Since_The_Contestant_Can.27t_Know_Of_Any_Host_Behaviour...

Glkanter (talk) 19:37, 20 April 2009 (UTC)

G - you're missing the point. In the "host forgets" variant it's given that the host opened door 3 and did not reveal the car. It's a conditional probability question - just like the standard MHP where it's given that the host has opened door 3. The only difference is whether the host did this on purpose or whether it was a fortuitous accident. -- Rick Block (talk) 00:57, 21 April 2009 (UTC)

Rick, sounds like you're missing the point, too. It's a totally different question when it's given that the host always opens a door with a goat than when the host opens a door at random. The probabilities depend on the game definition; when the usual definition is used, which door the host opens, and what's behind it, provides no new information, so the result of the conditional problem can be worked unconditionally before the game, or on the fly, without conditioning on which door was opened. Dicklyon (talk) 16:36, 21 April 2009 (UTC)

Dicklyon, sounds like you're also missing the point. You still doesn't seem to understand that it needs proof to work the conditional prob's - which are essentially needed - unconditional (at any stage). Calculate the question marks beneath. Nijdam (talk) 16:42, 21 April 2009 (UTC)

Right - the question is how do you know that in the standard version no new information is provided by the host opening a door? I mean, in the host forgets version, the host is actually acting completely randomly - how can there be more information provided in this variant? Yes, the "normal" rules ensure the unconditional and conditional probabilities are the same, but asserting the host's actions do not change the player's initial chance of having selected the car is effectively assuming the solution. What Nijdam is saying is that this assertion should have some reasoning behind it. The generally published popular solutions omit this. Mathematically, it's like a geometry proof that uses as a "fact" that an angle in the accompanying diagram is a right angle without saying why this is so (in a case where the angle is indeed a right angle). The published mathematical criticisms of the popular solutions are that these solutions either 1) don't answer the conditional question that is apparently asked (they answer question #1 from above, not #2), or 2) (if they're actually attempting to answer question #1) assume something without explaining why it's true - making them (as Morgan et al. put it) "false solutions" . -- Rick Block (talk) 18:41, 21 April 2009 (UTC)

Rick, you seem to the one be making assertions. For example what is your justification for saying, 'asserting the host's actions do not change the player's initial chance of having selected the car is effectively assuming the solution'? If the only information given is random information then it is no information, this is well accepted.

You have also ignored the question of what Whitaker actually wanted to know. Do you think he actually wanted the answer to the specific question Morgan took him to be asking or is it more realistic to take it that what he actually wanted to know was simply, 'Is it generally better to swap?'. I know which my money is on. Taking his rather vague question as being intended to be a formal conditional probability problem is simply a way of adding pointless academic complexity. A simple problem that most people get wrong is interesting and notable, a complicated problem that most people do not understand is not. Martin Hogbin (talk) 22:14, 21 April 2009 (UTC)

I have been arguing these points and others on the arguments page where you have not produced any evidence or arguments to the contrary. You now state your opinion as though it were fact. Martin Hogbin (talk) 22:14, 21 April 2009 (UTC)

I do - of course - fully agree with Rick. And for the people who still do not understand the issue: look at it this way: the door chosen is No. 1; "the" probability of hiding the car is:

door                    1    2    3 
before action host     1/3  1/3  1/3
after opening door 3    ?    ?    0

At least anyone agrees about the zero chance of door 3 (I hope). Therefore the second line is different from the former, and hence a different probability. The first line we call the unconditional probabilities (although the choice may also be considerd as a condition). The second line shows the conditional probabilities. On forehand we do not know much about the question marks, only they sum up to 1. Nijdam (talk) 09:27, 21 April 2009 (UTC)

Where are the brave ones to tell me what the question marks are? And why? Nijdam (talk) 22:49, 22 April 2009 (UTC)

What reliable sources actually say is that given that usual rules and if the question is interpreted such that the player has picked a specific identified door and the host has opened a specific identified door, the problem is conditional, which I agree. If the question is interpreted in a way that the host opens one (unspecified) of the two unchosen doors the condition becomes non-existent.

In the specific conditional case where the producer (or whoever) places the car randomly but the host does not open a door randomly the host's door opening strategy is important. In the case where the host chooses randomly the conditional case is equivalent to the unconditional one.

On the other hand, reliable sources, referring to the simple unconditional solution, say that no other statistical puzzle comes so close to fooling all of the people all of the time. They also say that incomplete information is not the cause of the difficulty most people have in solving the problem and that even when the problem is made quite clear, with the host choosing randomly, most people still get it wrong. It is clear that a substantial and separate part of this article should be devoted to clearly and convincingly explaining the basic (unconditional, fully defined or whatever) problem and solution. Martin Hogbin (talk) 18:07, 21 April 2009 (UTC)

Martin - Do you have a reference for your statement just above that the Massimo Piattelli-Palmarini quote ("no other statistical puzzle ...") refers to the unconditional solution? You keep saying the unconditional problem is the most notable one and the one that people get wrong. Do you have a reference for this claim as well? As far as I know, the problem is always stated in a conditional form, and (according to at least Falk, who is a psychologist who has studied it) it is the conditional nature of the problem that trips people up (Boy or Girl paradox being another classic example). Perhaps we can agree that people have trouble solving conditional probability problems using any method, but I think approaching MHP as a conditional problem is actually both more convincing and more useful than approaching it unconditionally. As I've said repeatedly, the problem I have with unconditional approaches is that the conditions under which they're valid are not at all obvious - the easiest example perhaps being the "host forgets" scenario for which the unconditional approach fails. -- Rick Block (talk) 14:08, 22 April 2009 (UTC)

requested ref. Dicklyon (talk) 15:55, 22 April 2009 (UTC)

This reference is where the quote comes from, but it doesn't say anything like "this is referring to the unconditional solution". It's clearly referring to the MHP - but Martin is claiming it's referring to his unconditional interpretation. -- Rick Block (talk) 16:21, 22 April 2009 (UTC)

I doubt that there is a reliable source saying that the Massimo Piattelli-Palmarini quote specifically refers to the unconditional problem or for that matter that it refers to the conditional problem, however, we do have some evidence on the subject from reliable sources. Krauss and Wang quote Piattelli-Palmarini on the second page of their paper where the subject of conditionality has not yet been discussed and shortly after they have said, 'These discussions have verified vos Savant's conclusion that it is is mathematically correct to switch...'. I note that this only says 'correct to switch' but also that it refers specifically to vos Savant's conclusion.

It is hard to see what other plausible meaning the quote could have. Are you suggesting that it only refers to conditional formulations of the problem and that otherwise people find it easy. Krauss and Wang discount this possibility.

My position on the problem has hardened a little. I now see the conditional issue as little more than an academic complication and K&W seem to support this view. I have tried several times to discuss this with you on the arguments page but you have not always engaged. I would be happy to continue this discussion there.

Your view is that reliable sources all say that the problem must be treated conditionally and that any solution that does not do so is false. That may be the view of Morgan et al for a specific formulation of the problem but such a rigid interpretation does not seem to be universal. So what I am essentially saying is that Morgan overstate the case for conditional treatment and you overstate the case that reliable sources only support this treatment of the problem. There is another side to the problemMartin Hogbin (talk) 17:22, 22 April 2009 (UTC)

One door to be selected - two doors will remain unselected (each regarded solely and regarded as a group of two doors)

For each single one of the two remaining unselected doors, each one of these two doors regarded solely for itself alone, the chance of winning amounts to 1/3 according to the rule (together thus 2/3). Risk to contain a goat for each of these two doors regarded solely for itself alone amounts to 2/3 (risk together thus 4/3).

[Nijdam]Let C be the number of the door with the car: P(C=c)=1/3 for c=1,2,3. That's all there is to say. Risks of 2/3 and adding up to 4/3 are unknown to me.Nijdam (talk) 22:19, 22 April 2009 (UTC)

@Nijdam: 4/3 unknown to you? What about training to count beyond 1, if possible to 2 at least. So: 3 doors, two goats. Risk for every single door=2/3. In case the player has chosen the winning door, there will remain two doors with two goats. Makes two goats for the two remaining two doors altogether. Repeat: 2 goats (or 6/3, if you like). But two goats are not granted every time. Only ONE goat is for sure, and for the second door a further risk of 1/3. So: 3/3 + 1/3 = 4/3 goats for two doors. Or in other words: one and 1/3 goat for two doors altogether. Kind regards, Gerhardvalentin (talk) 00:55, 23 April 2009 (UTC)

But since a group of two doors must inevitably contain at least one goat however according to the rule (there is only one car), one of these two remaining doors obviously has the risk=1 to contain a goat and a chance=0 to contain the car, imperatively from the start. This is a fact in reference to the group of TWO unselected doors (The player does not know yet to which one of the two doors this applies). As accentuated, this applies anyway only to ONE of the two doors that are going to remain unselected. Thus the other one of these two doors inevitabely will have a chance of winning of 2/3 and a risk to contain a goat of 1/3, imperatively from the start. (The player does not know yet to which one of the two doors this applies).

[Nijdam]Let X be the number of the door chosen by the player. Perhaps the meaning of this part is: P(C ≠ X) = 2/3. Nothing yet has been said about the probabilities after opening of one of the two remaining doors.Nijdam (talk) 22:19, 22 April 2009 (UTC)

The opening of one door by the host, showing a goat, changes neither the chance of winning of the door originally selected (1/3) nor the chance of winning of the two doors not selected (of together 2/3).

[Nijdam]This is no more than just a statement, that needs to be proven. Right is that the sum of the two (conditional) probabilities is 1. So if one in not changed, so is not the other. Without this proof, no more can be said. And besides: after the opening of the door, the probabilities at stake are conditional probabilities. Nijdam (talk) 22:19, 22 April 2009 (UTC)

However: The opening of the door with one goat shows the aforementioned distribution of chance and risk within the group of the two doors not selected. The door opened will have a risk to contain a goat=1 and will have a chance to contain the car=0. The other one that will remain still closed thus is the one with a chance of winning=2/3 and it has the remaining risk to contain a goat=1/3. All of that as a compulsive implication of the rules, imperatively from the beginning.

Opening the door by the host, showing a goat, did in no way bring any additional information regarding the position of the car, however. -- Gerhardvalentin (talk) 21:27, 21 April 2009 (UTC)

Comments? Say: What the article needs is a clear presentation of the given inevitable consequences that are valid right from the beginning, set by the original conditions of task. Of the clearly laid out intrinsic consequences that inevitably result right from the problem definition. A clearly represented definition of the initial position and its immanent consequences. -- Gerhardvalentin (talk) 10:47, 22 April 2009 (UTC)

@Nijdam: Thank you for your recurring thoughts and would like to suggest some experimental training / proof. Regards, -- Gerhardvalentin (talk) 23:09, 22 April 2009 (UTC)

The flaw in the assertion that "switching wins 2 out of 3 times" is this that there is only ONE guesser who only can only make ONE guess. Therefore switching has no bearing on the probability of the result. —Preceding unsigned comment added by 194.202.122.223 (talk) 08:29, 22 April 2009 (UTC)

If you are arguing that it is not better to switch then you are wrong. It is accepted by all that, in the simple case, you have a 2/3 chance of winning by switching. This page is for discussing ways to improve the article not the validity of the simple solution. If you want to discuss this probability further , I suggest that you do it on the arguments page. Martin Hogbin (talk) 09:33, 22 April 2009 (UTC)

The crux of the issue that dominates this page is the statement above:

The opening of one door by the host, showing a goat, changes neither the chance of winning of the door originally selected (1/3) nor the chance of winning of the two doors not selected (of together 2/3).

This is a true, but confusing, statement. I think a better, less confusing, way to phrase the same thing would be:

The opening of one door by the host, showing a goat, changes neither the overall chance of winning of the door originally selected (1/3) nor the overall chance of winning of the two doors not selected (each 1/3, so together 2/3).

The point is that the chances described in this statement are the overall chances, which are the chances in effect before the host opens a door. Nijdam puts it quite succinctly above

door                      1    2    3 
before host opens a door 1/3  1/3  1/3
after opening door 3      ?    ?    0

The chances this statement refers to are the 1/3:1/3:1/3 chances from the first line. The host does not, and can not, change these. When the host opens a door (say door 3) the chances have clearly changed. If we want to talk about chances that never change, then we need to talk about what happens when the host opens either door, perhaps like this:

                   1/3           +          1/3         +         1/3    = 1
                   /\                       /\                    /\
                  /  \                     /  \                  /  \
                 /    \                   /    \                /    \
                /      \                 /      \              /      \
host opens:  door 2   door 3          door 2   door 3       door 2   door 3
              /          \             /          \          /          \
             /            \           /            \        /            \
            ?       +      ?     +   0       +     1/3     1/3     +      0 = 1

What the statement above is actually saying is that for the player's chosen door ?+?=1/3 (not that each ? in this diagram is 1/3) and that the 1/3 for each of the unchosen doors don't change either (so, still, 1/3+1/3=2/3). The problem statement asks about the case where the host opens door 3, so (rearranging slightly) we have

door                      1     2     3                        1    2    3
before host opens a door 1/3 + 1/3 + 1/3 = 1
after opening door 3      ?  + 1/3 +  0  + (host opens door 2) ? +  0 + 1/3 = 1

In the case the host opens door 3, we have ? + 1/3 + 0. The sum is not 1, but the overall probability the host opens door 3. To make these terms conditional probabilities that sum to 1 we have to divide by their sum, but since one of the terms is ? we don't exactly know how to do this. If we assume the two ? terms are the same, they're both 1/6 and then we have 1/6 + 1/3 + 0, so conditionally we have 1/3 + 2/3 + 0 = 1 - but note that the 1/3 here is not the "chance of winning of the door originally selected" unchanged, but half of this. -- Rick Block (talk) 15:32, 22 April 2009 (UTC)

Rick Block: Did you consider that 1/3 + 1/3 + 1/3 only applies BEFORE the player made his choice?
Only before he made his choice. For:
As soon as he has chosen one of the three doors the situation has changed completely:

One door chosen: chance=1/3 and risk=2/3.

As to the two doors he denied (with an overall chance=2/3, overall risk=4/3):

One door NOT chosen chance=0 and risk to contain a goat=1 (There's only 1 car! But the player still does not know yet to which one of those two unselected doors this applies)

The other door NOT chosen: chance=2/3 and risk=only 1/3 (the player still doesn't know yet to which one of the two doors that have not been selected this will apply).

But after the host has opened one of the two doors not selected, showing a goat:

One door not chosen showing a goat chance=0 and risk=1 (Now the player knows to which one of those two unselected doors this applies, i.e. to the open door showing a goat).

The other door not chosen (still closed): chance=2/3 and risk is reduced to 1/3 (now the player knows to which of the two unselected doors this applies, i.e. to the still closed door)
Regards, -- Gerhardvalentin (talk) 20:16, 22 April 2009 (UTC)

One of several flaws in Rick's explanation is this. For some unexplained reason it is assumed that the producer acts randomly when he has the car placed behind one of the doors. If this assumption is not made then the chance of initially picking the car is not 1/3 but indeterminate. When it comes to the host's choice of door the host is presumed to act non-randomly thus we cannot assume that the host may pick door 2 or 3 with equal probability.

The above explanation therefore only applies in the somewhat contrived case that the producer (or his agent) is taken to act randomly but the host is not. If the both act non-randomly the question is indeterminate and if they both act randomly the chances of having picked the car, given that the host has picked a particular door are always 1/3. I would be interested to hear Rick's response to this. Martin Hogbin (talk) 07:00, 23 April 2009 (UTC)

The explanation above relates to the problem statement from Krauss & Wang where both the car placement and host's choice of door in the case the player initially picked the car are explicitly random. It can be easily extended to the interpretation of the Parade version analyzed by Morgan et al. and Gillman where the host's preference is treated as an unknown variable, but in the above the host's preference is assumed to be 1/2 (i.e. random).

It does indeed turn out that the chances of having picked the car remains numerically 1/3 if the producer acts randomly and the host acts as specified in the K&R statement of the problem, but this fact is the result of the analysis not the reason the analysis ends up the way it does. If we assume the doors (and goats) are indistinguishable, i.e. treat the problem as an urn problem (which forces the random choices Martin mentions), the marginally simpler approach of enumerating all possibilities assuming the player switches is valid and leads to the same result without using conditional probability (these conditions force all conditional outcomes to have the same probability as the unconditional outcome).

On the other hand, if we're answering the conditional question that the problem statement apparently asks (flipping from win by switching to win by staying, the question is what is the probability of the player's door being the one with the car given the player has picked door 1 and the host has opened door 3), the "1/3" which is the probability of the player's initial chance of picking the car is not the same as the "1/3" corresponding to this conditional probability. For example, if we're talking about 3000 players who have picked door 1, we'd expect about 1000 to have selected the car and if all 3000 stay with their initial choice only these 1000 will win the car (and, if all 3000 switch, the other 2000 will win the car). The initial "1/3" is these 1000 players. After the host has opened door 3 we're no longer talking about all 3000 of these players but only a subset. If the host picks randomly between doors 2 and 3 if the player has initially selected the car, we're talking about roughly 1500 of the 3000. Of these, about 500 will have initially selected the car. These 500 is what the conditional probability of "1/3" is talking about. These two "1/3" are of course numerically the same, but they correspond to distinctly different sets of players. -- Rick Block (talk) 13:56, 23 April 2009 (UTC)

Of course the conditional sample is a subset of the unconditional one but, in the case that the host acts randomly, it is a representative sample since it is taken randomly. So yes, the problem is conditional in the sense that we have applied a condition but it is a condition that makes no difference. If the host chooses randomly we can fill in the question marks in your diagram above with the value 1/6 (as is done in the article itself). As others have pointed out this is not a numerical coincidence but the result of an obvious symmetry. Now tell me why the case that the car is not initially non-randomly placed is never considered. Martin Hogbin (talk) 21:27, 23 April 2009 (UTC)

The non-random initial placement certainly can be considered, and there are actually sources that examine a variant where the player knows the initial non-random placement. About such a variant, Morgan et al. say "Other variations appear to be of less interest. One possibility is to incorporate prior information on the part of the player as to the location of the car, or, related to this, to allow nonuniform probabilities of assignment of the car to the three doors, but these are unlikely to correspond to a real playing of this particular game show situation." My opinion (which I freely admit is WP:OR) is that the variant Morgan et al. (and Gillman, who you keep ignoring) analyze pretty much exactly matches an actual game show situation (of course, the actual rules of Let's Make a Deal were not the same as any version of the MHP, so this entire discussion is one of conjecture). In an actual game show the car would be hidden, and as Glkanter has observed, providing the player information about the location of the car would violate US laws, which means the initial location should be assumed to be random. The constraint that the host pick randomly if the player initially selects the car is NOT in most statements of the problem (and doesn't seem to me like something a game show would clarify), so when analyzing the conditional probabilities it seems entirely reasonable to consider the case where the host has a preference (and, to Glkanter - it doesn't matter whether the player knows this preference or not, it influences the probability in either case - and, to you Martin, this preference might be entirely momentary and might change player by player or day by day). Assigning this preference to a variable, and analyzing the extremes says the player is never worse off switching.

If we are going to refer to the actual show then I see no greater reason to assume the car was initially placed randomly than to assume the host chooses randomly. In reality they were probably both roughly random. They probably put the car behind a door without thinking too much about it and the host probably made up his mind at the time, having no special preference. Martin Hogbin (talk) 17:03, 24 April 2009 (UTC)

If where you're really going is that we should assume the host picks randomly in the case the player initially selects the car, please tell me where in the simple solution we say anything like "in the case that the host acts randomly, it is a representative sample since it is taken randomly" or the conditional cases are clearly the same because "of an obvious symmetry". As far as I recollect, I've never seen an unconditional solution that actually provides any kind of justification for ignoring the possibility that the host might have an unknown preference. To some extent, as Dicklyon would say, this entire discussion is moot since we should say what the sources say - not what we might wish they say. -- Rick Block (talk) 01:31, 24 April 2009 (UTC)

Vos Savant later justified her solution by saying that she took the host to be acting purely as the agent of chance. All the sources make clear that the chances of winning by switching are in fact 2/3 if the host chooses randomly. So what we have is a solution that ignores something that makes no difference and gets the right answer, it probably shares these features with most mathematical solutions. Martin Hogbin (talk) 17:20, 24 April 2009 (UTC)

Explaining the solution

Before the player makes her choice, there are, in conformity with the rule: 1 car, 2 goats, and three closed doors, each one
with a chance of winning of 1/3 and a risk to contain a goat of 2/3. Chances= 1/3 + 1/3 + 1/3, risks= 2/3 + 2/3 + 2/3.

After the player has made her choice however to one of the three doors (with a chance=1/3 and a risk=2/3), the situation has changed dramatically:
The remaining residue of the two unselected doors, with an overall chance of winning amounting together to 2/3, and their overall risk of together 4/3, must – as to the rule – inevitably contain at least 1 goat with a chance=0 and a risk=1 (for, as to the rule, there’s only one car).

Solely from the terms of the rule, the compelling implications are:

The selected door has a chance=1/3 and a risk=2/3 (no more info about this door).

One of the two doors that have not been selected has inevitably a chance=0 and a risk=1 (Only 1 car. The player still doesn’t know to which one of those two doors this will apply).

The other one of those two unselected doors – according to the rule – thus has inevitably a chance of 2/3 and a risk of only 1/3 (The player still does not know to which one of the two unselected doors this will apply).

But when the host opens one door from the rest of the two unselected doors, showing a goat, he shows to the player the position of the inevitable rivet, and by this the player also has knowledge of the position of the (still closed) door with the chance of 2/3 and the risk of 1/3.

Its chance of winning is twice as high (2/3) as the chance of winning of the door originally selected by the player (1/3), but in one third of all cases (i.e. whenever the player should coincidentally have selected the winning door with the car), it will contain a goat also.

So, the opening of the door containing a goat has definitely shown the distribution of chances within the group of the two unselected doors, but beyond that it has not given any further information regarding the chances of the door originally selected (1/3) nor regarding the chances of the group of the two unselected doors (overall by 2/3), nor any reference to the actual position of the car, whatsoever.

All these facts stated above do result exclusively from the regulations already specified in the rule, and from its inevitable consequences. And any mathematical calculations, if provided correctly, will lead to the same results:

In 1/3 of all cases, in which the player should coincidentally have selected the winning door, she would lose by a change and win by persisting. In the other 2/3 of all cases, in which she should have selected one of the two doors each containing a goat, she wins by changeing and loses when persisting.

Thus, by changing, the player doubles her chance from 1/3 on 2/3.

The persistent "WHY ?" should be considered, and should be responded. Who will put this info on top of the article? Kind regards, -- Gerhardvalentin (talk) 22:04, 22 April 2009 (UTC)

@Gerhardvalentin. Your problem is partly your unfamiliarity with probability theory. You try to make computations, from which I understand the purpose, but the focus is on the wrong issue. You should focus on why the probability of the door initially chosen to hide the car is 1/3 before the opening of one of the other doors, and also 1/3 after. That is the whole and only issue. Nijdam (talk) 22:28, 22 April 2009 (UTC)

@Nijdam: Probability theory is concerned with analysis of random phenomena. It's not fair to blame reality for resulting misjudgements of any calculations whatsoever, i.e. stochastics not to be neglected. Thank you for your efforts, hope you're going to find a correct theorem that corresponds to reality. Regards, -- Gerhardvalentin (talk) 23:34, 22 April 2009 (UTC)

@Gerhardvalentin. The problem is that Rick and Nijdam cannot decide which model of probability to apply. If you apply the 'probability is a state of knowledge' principle and take it that any information not given should be treated as random, then you are right. The player has no knowledge of the initial car placement or the host's door choice policy, we therefore should take both of these to be random, and the chances of winning by switching are consequently 2/3.

If, one the other hand, we take the more formal view that anything not specified in the problem statement must be taken as indeterminate it is possible to contrive a case where the probability of winning by switching is not 2/3. If you are interested I can show you how. Martin Hogbin (talk) 07:15, 23 April 2009 (UTC)

I'm really amused about such statements. Rick and I do perfectly know what to do and what to apply. And whatever model we or you apply, the needed solution is with conditional probabilities, whether you like it or not. Nijdam (talk) 14:51, 23 April 2009 (UTC)

Nijdam - the difference here is that Martin wants to treat the problem as an urn problem which might be stated as follows:

There are one white ball and two (indistinguishable) black balls in an urn. The player withdraws one without looking at it. The host now looks in the urn and withdraws a black ball and shows it to the player. The host offers the player the opportunity to switch for the remaining ball in the urn. If the player wins a car by ending up with the white ball should she switch?

This is the problem the "unconditional" solutions address. Martin's claim is that this is the "true", "notable" MHP. Unfortunately, in the MHP the doors have numbers and physical locations on a stage so the doors are clearly distinguishable which you and I are saying means it's NOT an urn problem, but a conditional probability problem. -- Rick Block (talk) 15:18, 23 April 2009 (UTC)

Let me answer the question of what I am trying to do. It is something quite separate from the conditional issue and from the distinguishability of the doors.

Consider this quotation from the Three Prisoners Problem (where the prisoners are all clearly distinguishable), 'Prisoner A, prior to hearing from the warden, estimates his chances of being pardoned as 1/3, the same as both B and C'. Note that A estimates his chances of being pardoned as 1/3. This is not strictly valid as nothing in the problem statement says that the choice of prisoner to be pardoned is random, however, it is taken that, as the prisoner has no information as to who will be pardoned, he will estimate his chances as 1/3. This is common, but less formal, way of looking at things. If some information is unknown it is taken to be random. In Morgan's interpretation of the MHP this approach is not taken. Although the player has no knowledge of the host's door opening policy we do not take this to be random. Martin Hogbin (talk) 22:48, 23 April 2009 (UTC)

Ok forget about the math, let's tackle the english!

Why does it matter if the host knows or does not know which door the car is behind? If the host does not know and opens up a door the result is either a goat or a car. If it's the car well, he can't offer you a choice of switching, that's just dumb. So once the game has reached the stage of "Do you want to switch?" the math shows it's probably in your best interest to switch. 190.93.76.58 (talk) 05:31, 25 April 2009 (UTC)

Evidently you haven't read or understood much of the stuff that's already been said about this. It does matter very much how you got to that game state. Having one door open and two doors to choose between can have all sorts of different probabilities on the two doors depending on the rules of the game, how you got there, and what your model of the host behavior is. Dicklyon (talk) 05:36, 25 April 2009 (UTC)

And yet you [Dicklyon] have missed my point and completely overlooked the heading of my discussion. Math is math. You either get the right answer or the wrong answer. What is intriguing about the Monty Hall problem is common human misconception in deciding weather to switch or not switch and the tendency to choose the outcome not in your best interest. Consider a change up of the old question.

Suppose you're on a game show, and you're given the choice of three doors: Behind one door is a car; behind the others, goats. You pick a door, say No. 1, and then the host, says would you like a chance to see what is behind one of the doors you didn’t choose. You say yes. He then asks you to choose another door. So you choose a door No. 3 and it’s a goat. [1]. He then says to you, "Do you want to switch to door No. 2?" Is it to your advantage to switch your choice?

[1] And in case your wondering if you choose door No. 3 and it’s the car, no you don’t win it. He just says “Too bad, maybe you should have choose door No. 3 first.” You go home knowing you picked the car only at the wrong time.

As I said math is math. Once your at "Do you want to switch to door No. 2?" the math is the same regardless of knowing/not knowing what is really behind door No. 3. and the rational solution is to always switch. Just remember the original problem states the host knows what's behind door No. 3, it never said he told you he knows.

190.93.76.58 (talk) 08:22, 25 April 2009 (UTC)

Not so. You need to draw yourself a diagram to prove this but if the host opens any unchosen door randomly there are three possibilities each having equal probability. These are: you have chosen a goat and the host reveals another goat (you win by switching), you have chosen a goat and the host reveals a car (game is void), you have chosen the car and the host reveals a goat (you lose by switching). Considering only the non-void games, you have the same probability of winning by switching as you have of losing by switching. The fact that it matters whether the host always opens a door to reveal a goat (in which case he must know where the goat is) or opens either of the two doors not chosen by the player randomly, and we then disregard (or replay) the cases where he happens to reveal a car, is surprising and it is what the Monty Hall problem is all about. Martin Hogbin (talk) 14:04, 25 April 2009 (UTC)

I think we should stop discussing novel interpretations bases on content-free nonsense like "math is math", and get back to discussing how best to improve the article based on sources. Interpretations and developments not based on sources are irrelevant to our deliberations. Dicklyon (talk) 14:40, 25 April 2009 (UTC)

Anyone mind if we simply move this thread to the /Arguments page? -- Rick Block (talk) 17:33, 25 April 2009 (UTC)

I am sorry if anyone feels disagreeable to the discussion this topic is on. Just to reiterate this discussion is not about math. If you can’t handle this basic math take a refresher class or two.

My contribution is about the human aspect of the Monty Hall problem whereby enough data is presented to the contestant and he is asked to make a decision and inevitably chooses an answer not in his best interest.

Furthermore the Monty Hall problem is not a mid term math problem where you have 30 minutes to solve it, show all working. You don’t have 30 minutes, you are not required to use only mathematical algorithms, you are on TV and the host is expecting a response in 30 seconds. It is an entertaining puzzle that allows one to look into human psychology.

A “Monty Hall problem” has the following simple characteristics: - there are three doors - there is a prize behind each door - two of the prizes are undesirable one is desirable - you are asked initially to choose one door - subsequently one door which was not chosen is opened to reveled one of the undesirable prizes. - you are then asked “Do you want to switch to door …?” My solution is simply recognize the problem is of type Monty Hall and always accept the switch which is in your best interest.

As for you math purest. The acceptable answers to the Monty Hall problem are “Yes, I will like to switch.” or “No, I do not want to switch.” There is no “If I choose Yes to switch the probability of that is …” response required.

The actual structure of a Monty Hall problem (elegant or not) can change the working of the mathematical solution but it does not affect that in the general sense the option to always accept the switch is never mathematically not in your best interest.

The root of this discussion deals with whether the host knows or does not know what is behind each door and its effect on reveling a door with a goat. The information stated that the host knows what is behind the doors is for an observer to the problem. Remember you are the contestant and you have to arrive at your solution based on the information presented to you. The Wikipedia article does not discuss this in length. 190.93.76.58 (talk) 02:38, 26 April 2009 (UTC)

What the article says is what reliable sources say about the problem. Are there reliable sources you can point to that are not represented by what the article says? Conversely, if what you're saying is not published in reliable sources it should not (must not) be in the article. -- Rick Block (talk) 03:36, 26 April 2009 (UTC)

I thought you [Rick Block] might say that. My rebuttal is simply if someone made a comment on the Monty Hall problem based on mathematics which could be shown to be in error you would not include it as part of the article even if it were published. However, I disagree with the logic of some of your "reliable sources" in solving the Monty Hall problem using the assumption that the host knows what is behind the doors just because he was able to open one door and show a goat. Yet you have many citations to "reliable sources" that purport this idea. The Month Hall problem is a simple problem and I am not going to look for a published article that supports my comments. My contribution to the article is from the perspective of the reader, your article structure should not imply any one discussion on the logic aspect of solving the Monty Hall problem is right (or wrong) just by citing sources. Also, your article goes on and on about the different mathematical methods and the varying possible probability solutions, but does not clearly answer the fundamental question asked. Do I switch doors or not?

190.93.76.58 (talk) 04:19, 26 April 2009 (UTC)

The article says (in the lead) "In fact, the player should switch—doing so doubles the probability of winning the car from 1/3 to 2/3.". Is this not clear enough? Regarding the "assumption" that the host knows what is behind the doors, the problem statement (the one from Parade) says "You pick a door, say No. 1, and the host, who knows what's behind the doors, opens another door" (emphasis added). This is not an inference based on the fact that the host was able to open a door showing a goat (which indeed some sources argue), but is explicitly given by the problem statement. It would seem you're thinking of some other statement of the problem. If you're not willing to offer published sources supporting your comments, you're on the wrong website. -- Rick Block (talk) 17:00, 26 April 2009 (UTC)

I can see you have fallen into the Monty Hall trap. Not the trap that argues whether you have a 2/3 or 1/2 chance by accepting the switch but the trap that the Monty Hall problem is a math problem. Seeing just how many educated people are confused just by the implications of the different mathematical permutations to the Monty Hall problem analysis, I can see you "adamantly defending your stand in print."

Just to be clear. "In fact, the player should switch—doing so doubles the probability of winning the car from 1/3 to 2/3." is not an answer, especially to a question with a riddle component; the answer is "Yes, I accept the switch." notice the full-stop after switch. If you want to support the answer using mathematics go ahead but that is not part of the answer.

Regarding the "assumption" that the host knows what is behind the doors, the problem statement (the one from Parade) says "You pick a door, say No. 1, and the host, who knows what's behind the doors, opens another door" (emphasis added). Who exactly is this information for? If Monty himself was calculating the best odds he was giving his contestant he would arrive at 2/3 chance. How is the contestant to know that Monty knows what's behind the doors. If Monty had clearly said, "Now, before I open the door No. 1 you have chosen let me first open one of the doors with a goat" and then he offered the switch it is clear to the contestant (who is the one playing the game and the one the question is directed to) that Monty knows what is behind the doors.

As there are rules w.r.t citing sources to support arguments which you keep reminding me this will me my last post since I am not as invested in your Monty Hall article as you are. Just note your article has to glue the citations together for readability, unfortunately it seems you have force fit some of the puzzle pieces together.

190.93.76.58 (talk) 18:33, 26 April 2009 (UTC)

Once more, How about we delete the 4th paragraph of the Sources of Confusion section?

Here's what it says:

"Another source of confusion is that the usual wording of the problem statement asks about the conditional probability of winning given which door is opened by the host, as opposed to the overall or unconditional probability. These are mathematically different questions and can have different answers depending on how the host chooses which door to open if the player's initial choice is the car (Morgan et al., 1991; Gillman 1992). For example, if the host opens Door 3 whenever possible then the probability of winning by switching for players initially choosing Door 1 is 2/3 overall, but only 1/2 if the host opens Door 3. In its usual form the problem statement does not specify this detail of the host's behavior, making the answer that switching wins the car with probability 2/3 mathematically unjustified. Many commonly presented solutions address the unconditional probability, ignoring which door the host opens; Morgan et al. call these "false solutions" (1991)."

This is just another Morgan pov. And it adds a non-existent premise, so it's not even addressing the MHP. Please refer to the arguments page http://wiki.riteme.site/wiki/Talk:Monty_Hall_problem/Arguments#This_is_Morgan.27s_Entire_Argument_Against_the_.22Combining_Doors.22_Solution where the Morganians agree that this is not a source of mathematical confusion, but merely Morgan's opinion of what Whitaker is NOT asking. Glkanter (talk) 09:58, 28 April 2009 (UTC)

I am not sure that I would want to delete this but it certainly should be in an 'academic solutions' section. It is of no relevance at all to the notable MHP. Martin Hogbin (talk) 17:43, 28 April 2009 (UTC)

Has anything changed since the last time we discussed this 2 weeks ago? -- Rick Block (talk) 00:02, 29 April 2009 (UTC)

Talk:Monty Hall problem/Archive 10

New archive

Slow down, guys!

General criticisms of the article

Criticism and a new approach

(Counter)example

Replace the Popular Solution Section With the 'Combining Doors' Solution

A concrete proposal

Comments on the above proposal

Diagram

New "Traditional solution" section

How complicated is it???

Combining Doors Solution

More than three choices

The stages in the decision

1. Before the player made her choice

2. After the player has chosen a door and before the quizmaster opens one

3. After the player has chosen a door and the quizmaster has opened one

Outline changes

Suggested wording for Popular solution section

Is it just me or does this appear to be illogical?

Weasels ahoy!

Let's Eliminate the "Criticisms of the traditional solution" section

Maybe a way forwards

How about we delete the 4th paragraph of the Sources of Confusion section?

Popular solution diagram

Congrats

Popular solution door numbers.

Why does the Conditional Solution begin with the non-existent 'equal goat door constraint?

Aids to Understanding

Proposed new section - Problem formulations and approaches

One door to be selected - two doors will remain unselected (each regarded solely and regarded as a group of two doors)

Explaining the solution

Ok forget about the math, let's tackle the english!

Once more, How about we delete the 4th paragraph of the Sources of Confusion section?