Talk:Cube root

Infinitely nested cube roots

What is the point of this section ? This is an encyclopedia, not a bunch of random stuff from high school math problems. I think this part should be deleted.

Related image

Plot of y = ∛x by Mathematica.

User:Shen has replaced the old picture with a newer one (that is probably better). Ah well... I don't like images (especially ones I made) to disappear into oblivion. :-) — Ambush Commander^(Talk) 17:03, 29 January 2006 (UTC)

Aha, yeah... Sorry about the confusion. I'll get round to telling people if I do it again (really) Shen 21:29, 29 January 2006 (UTC)

Uh... no need to be sorry. :-D If it's a better picture, it's a better picture. — Ambush Commander^(Talk) 21:34, 29 January 2006 (UTC)

Plot of y = ∛x,the new image.

Both the new and the old image suffer from the same problem, the numbers on the axes are too small. Would be nice if the picture is redrawn with biggerfont sizes. Oleg Alexandrov (talk) 03:29, 30 January 2006 (UTC)

Well, part of the problem is the resizing of the plots. — Ambush Commander^(Talk) 03:36, 30 January 2006 (UTC)

Good point. The first picture does not scale the axes, and is more natural. Unless the new picture fixes that and the font sizes, I would suggest using the old one. Oleg Alexandrov (talk) 21:07, 30 January 2006 (UTC)

dxvs\fg ]\]u90-[u90] uj[u90- —Preceding unsigned comment added by 86.154.161.191 (talk) 19:38, 11 September 2008 (UTC)

I gave it some bigger fonts (increased the size of everything by 2). It should look a lot nicer! Shen 21:11, 30 January 2006 (UTC)

Thanks, looks good! Oleg Alexandrov (talk) 02:23, 31 January 2006 (UTC)

The Cube Root and Root-Solving History

I found a cube root method in a book on Vedic Mathematics. There is an algebraic digit schedule. The method is simple enough to be done by mental math. If one has already memorized the cubes in the first twelve digits then you can obtain the first and last digit on sight of the perfect cube. If you have the digit schedule then you can calculate the middle digits. Example: four Cube Root digits: HJKL ==> Start with the units digit in the root, L, as the units digit in the cube root of L³ in the number.

A Hindu woman traveled to San Jose, California about 20 years ago and beat a computer at the 23rd root of a 50?-digit number in a demonstration of mental math and Vedic algorithms. Apparently she had the general formula. More later. Larry R. Holmgren 09:40, 1 March 2007 (UTC)

This method is only useful on TV shows. Most numbers have no integer cube root so the algorithm cannot be applied. Thus it is only a curiosity. It cannot be extended to work for any N-th root (N odd) as claimed above: for N=11 there are 8 possible endings for eleventh roots of ...7056: namely 0006, 1256, 2506, 3756, 5006, 6256, 7506, 8756. --Alpertron 12:59, 30 March 2007 (UTC)

It is for sure that the issue on the extremely simple and general high-order arithmetical root-solving methods shown in the aforementioned webpage: http://mipagina.cantv.net/arithmetic/rmdef.htm are of so much relevance to the Cube-Root's article and its history, indeed. The history of root-solving is plenty of Tricks and Patches (as for example: those Vedic tricks you have brought to light), however the true is that no natural arithmetical methods habe been developed --since Babylonian times up to now-- by far similar to these new general high-order methods shown in the aforementioned webpage, and frankly this is so worrying. Author: D. Gomez.

CUBE ROOTS

Cube Roots of exact cubes, mainly by inspection and argumentation, page 316-317. Place-value analysis of a three-digit cube root, cba, page 318. Analytic sorting of the expansion into place-values, the three-digit cube root (cba) schedule of subtractions for cube roots of a given number, page 319, 321. First divide out any factors of 8, or 64, or 512, from the number to remove ambiguity in digit selection, page 323. Knowing the cubes of the first 10 counting numbers enables one to match the units digit of the given number (the perfect cube) to the cube root. A similar analysis is done to infer the next digit, b, by seeing the right-most digit in the cube. If the remainders grow too large, go back and increase the quotient digit. The following step's deductions will be larger.

What book is this coming from?Nickalh50 (talk) 12:23, 11 February 2011 (UTC)

Digit schedule of subtractions for a three-digit cube root, cba

The units place is determined by a3. 
(Subtract it to eliminate the units digit.) 
The tens place is determined by 3a2b. 
The hundreds place is formed by 3ab2 + 3a2b. 
The thousands place is formed by b3 + 3b2c. 
The ten-thousands place is given by 3ac2 + 3b2c. 
The hundred-thousands place is contributed by 3bc2. 
The millions place is constituted by c3.

Example one:

What is the cube root of the perfect cube —The preceding unsigned comment was added by Larry R. Holmgren (talk • contribs) 00:56, 6 March 2007 (UTC).

Four-digit schedule of subtractions for cube roots HJKL, pages 321, 323, 325.

Given the cube of a number, we work from right to left. 
To determine the units place, L, examine the units digit in the cube. 
Deduct L3.  
The tens digit is K.  Evaluate and analyse 3L2K. 
Deduct 3L2K. 
For J, the hundreds digit, evaluate and analyse 3L2J + 3LK2. 
Deduct 3L2J + 3LK2. 
For H, the thousands digit, evaluate and analyse 
3L2H + 6LKJ + K3. 
And 3L2H + 6LKJ + K3 is the portion to deduct.

Four-digit cube root, HJKL Method

1. Subtract (L³) to eliminate the units digit. We have L by the final digit pattern in the cube.

2. Subtract (3L²K) to eliminate the penultimate digit to find the cube root digit K.

3. Subtract (3L²J+3LK²) to eliminate the pre-penultimate digit to find the cube root digit J.

4. Subtract (3L²H+6LKJ+K³) to eliminate the prior digit to find the cube root digit H.

Example: Find an incompletely given perfect cube. 
Find the cube root of a 12-digit perfect cube 
whose last four digits are 6741.  
Thus, on sight, we known that 
the number of cube root digits = 12/3 = 4; Root digits H = ? and L = 1.  
Framework:  ___,___,___6,741. = (HJKL)3  
                                                                                                            
For L : L=1, Therefore L3 = 1.  Subtract it.           ………6741                                            
For K:  Deduct 3L2K = 3K (ends in 4).                  ……-……1                               
The 3rd multiple of 8 ends in 4. K=8. Deduction:3K=24. ……-…24  
For J:  Deduct 3L2J+3LK2 = 3J+192 (ends in 5).        ………65x  
So, 3J ends in 3, J=1. Deduction = 3+192=195.       ……-…195  
For H: Deduct 3L2H+6LKJ+K3 = 3H+48+512 = 3H+560.      ………7x  
(ends in 7). So, 3H ends in 7.                     …-……587 
The 9th multiple of 3 ends in 7. So H=9.              ………x
(No need to deduct 27+560=587.                                  
Therefore, (HJKL)3 = (9181)3 = ___,___,__6,741.

General cube roots

General cube roots can be calculated using a long division framework and rightward, column-wise division. The sequence of digits for a three digit schedule, for cube roots, abc, given the number (100a+10b+1c)³ + R, page 327:

The millions place is determined by a3.  
The hundred-thousands place is determined by 3a2b.  
The ten-thousands place is formed by 3ab2 + 3a2b.  
The one-thousands place is formed by b3 + 3b2c.  
The hundreds place is given by 3ac2 + 3b2c.  
The tens place is contributed by 3bc2.  
The units place is constituted by c3.  

The Dividends, Quotients, and Remainders: The divisor in cube roots is 3c³. The units digit, c, is obtained by inspection of the first group of digits in the cube after the digits of the given number are grouped in threes. Since we know (or have a reference list of) the first ten cubes we may see the nearest cube below the first group at sight.

The first D, Q, and R are available on sight.  
Use the following adjustments to the gross dividend at each step.  
From the second dividend no deduction is made. 
From the third dividend subtract 3ab2. Note the change.  
From the fourth dividend subtract 6abc + b3. Note the change.  
From the fifth dividend subtract 3ac2 + 3b2c. 
From the sixth dividend subtract 3bc2. 
From the seventh dividend subtract c3. 

The divisor should not be small, try two groups (4, 5, or 6 digits) as the first group, page 330. The four-digit schedule for cube roots, abcd, given the cube (1000a+100b+10c+1d)³, or an incomplete cube with a remainder. pages 337-338.

The analytic digit schedule:

First digit, billions = a3 
Second digit, hundred-millions = 3a2b. 
Third digit, ten-millions place = 3ab2 + 3a2c 
Fourth digit, one-millions place = 6abc + b3 + 3a2d 
Fifth digit, hundred-thousands place = 6abd + 3ac2 + 3b2c 
Sixth digit, ten-thousands place = 6acd + 3bc2 + 3b2d 
Seventh digit, one-thousands place = 6bcd + 3ad2 + c3 
Eighth digit, hundreds place = 3bd2 + 3c2d 
Ninth digit, tens place = 3cd2 
Tenth digit, ones place = d3 

The divisor in cube roots is 3a³. The deduction schedule for the long division, rightward, column-wise method for cube roots.

For the first dividend, Q1 and R1 are obtained by inspection, a3. 
From the second dividend Q2 and R2 by simple division, no other subtraction! 
From the third gross dividend subtract 3a2b.  
From the fourth gross dividend subtract 6abc + b3  
From the fifth gross dividend subtract 6abd + 3ac2 + 3b2c  
From the sixth gross dividend subtract 6acd + 3bc2 + 3b2d 
From the seventh gross dividend subtract 6bcd + 3ad2 + c3 
From the eighth gross dividend subtract 3bd2 + 3c2d 
From the ninth gross dividend subtract 3cd2 
From the tenth gross dividend subtract d3 

An alternative method is to cut the four-digit schedule to three-digits by equating d=0, page 338, and substituting (c+d) for c, page 340.

No Vedic sutra mentioned for cube roots by the author of Vedic Mathematics: Sixteen Simple Mathematical Formulae from the Vedas, by Swami Sankaracarya (1880-1960), Motilal Banarsidass Indological Publishers and Booksellers, Varnasi, India, 1965; reprinted in Delhi, India, 1975, 1978. 367 pages.

Examples and several cube root digit schedules are given. 5th root, 7th root, and nth root general formula only hinted at by the binomial expansion of that power. Page 340, Vedic Mathematics, 1965, 1978. Larry R. Holmgren 20:03, 3 March 2007 (UTC)

How much space is appropriate for examples? Larry R. Holmgren 19:02, 4 March 2007 (UTC)

"The cube root" vs. "A cube root"

The introductory paragraph begins: "In mathematics, the cube root of a number, denoted ${\sqrt[{3}]{x}}$ or x^1/3, is the number a such that a³ = x. All real numbers have exactly one real cube root and 2 complex roots, and all nonzero complex numbers have 3 distinct complex cube roots."

As there are generally three cube roots, shouldn't we replace the with a? The new text would read: "In mathematics, a cube root of a number, denoted ${\sqrt[{3}]{x}}$ or x^1/3, is a number a such that a³ = x. All real numbers have exactly one real cube root and 2 complex roots, and all nonzero complex numbers have 3 distinct complex cube roots." DRE 18:01, 20 February 2007 (UTC)

Sounds good. I've went ahead and made the change. — Edward Z. Yang^(Talk) 00:18, 4 March 2007 (UTC)

Technically, the complex numbers are the set of numbers in the form a + bi where a and b are real numbers. So, all real numbers are also complex numbers, if b = 0. Thus, perhaps it would be to say "exactly one real cube root and 2 nonreal roots..." StatisticsMan 20:03, 21 April 2007 (UTC)

Except when a is also zero. In this case all three roots are real. --Alpertron 13:18, 30 April 2007 (UTC)

Well, distinct cube roots, anyway. — Edward Z. Yang^(Talk) 19:13, 30 April 2007 (UTC)

[Query by Joe user] 18:30, 22 October 2007 (UTC)

I have another question about the introductory paragraph. How many cube-roots does zero have?

Also, some explanation of the 3D plot would be appreciated. The vertical axis is clearly the imaginary part of the complex cube-root. Which of the two horizontal axes is the real number whose cube-root is being evaluated? Obviously the remaining axis is the one that is real (part of the complex) cube-root.

[End query by Joe user] 18:30, 22 October 2007 (UTC)

Algorithm to computing cube roots using square roots

I had to revert the algorithm to the original one I posted on January 2006. Unfortunately in this edit someone changed the order of the operation, resuting in a loss of precision. Notice that trying to fix an non-existent bug he introduced one. Best regards, Alpertron (talk) 01:05, 8 November 2008 (UTC)

2 July 2009

The text:

This process continues until the number does not change after pressing the multiplication button because the repeated square root gives 1 (this means that the solution has been figured to as many significant digits as the calculator can handle). Then, press the square root button one last time. At this point an approximation of the cube root of the original number will be shown in the display.

makes no sense vis a vis the calculator available on a Windows XP platform. The algorithm works when you execute it as indicated in the text preceding this paragraph, at which point the display shows the correct cube root. Taking one last square root destroys this result.

It is unclear what numbers are actually being multiplied when the * key is pressed on the Windows calculator, and from my own personal experience, different commodity calculators do different things in this context of key sequences. This section of the article therefore needs some clarification, and the assumed behaviour of the calculator keys should be explicated in the "Why the algoithm works" section. —Preceding unsigned comment added by 199.199.129.35 (talk) 17:26, 2 July 2009 (UTC)

The error occurs because the article was vandalized on June 20th. Please try again the algorithm pressing only once the square root button at the beginning instead of twice (this was added on June 20th and I had to revert it). Best regards, Alpertron (talk) 19:51, 3 July 2009 (UTC)

After working out the algebra explicitly in order to see how the algorithm works, I see that there are really two methods that work:

• The initial factor (the square of one half) in the infinite product is applied initially via two square roots, at which point the value at the end of each cycle shows an increasingly close approximation of the cube root of x.

• Only half of the initial factor is applied via a single square root, followed at the end by one additional square root, which applies the other half of the initial factor. In effect, this method computes x raised to the 2/3, using the identity ((x ^ 2/3) ^ 1/2) = x ^ 1/3. In this method, the value at the end of each cycle shows an increasingly close approximation of unity, showing that the continuing factor of the infinite product that exponentiation transforms the original infinite product into is giving smaller and smaller corrections.

It is unclear that either method is better or easier to remember. Certainly neither is more correct. Therefore the change made on 20 June 2009 is not really vandalism, just an incomplete good faith edit by someone with a different perspective. —Preceding unsigned comment added by 199.199.129.35 (talk) 23:08, 7 July 2009 (UTC)

The change was wrong (if that change also includes erasing the last square root), because the final result would be less approximate to the true answer. You need to work out the error analysis to see this. Best regards, Alpertron (talk) 11:53, 8 July 2009 (UTC)

Proposed removal of "Continued fraction" material to main article generalized continued fraction

The section Cube root#Continued fraction is out of place here. It should be moved to a different article. The generalized continued fraction article already has a section for continued fractions of roots, and so this material is much more germane there. We should be observant here both of WP:WEIGHT and summary style. Having long and ugly sections with many continued fraction expansions does not improve this article. I don't see so much of a problem mentioning that such and so expansions exist. Le Docteur (talk) 18:09, 10 November 2009 (UTC)

Very well. It has been so moved. I'll not press the point any further. ---Glenn L (talk) 23:32, 10 November 2009 (UTC)

Update: since making the move it has been determined that, as far as generalized continued fractions are concerned, the cube root is simply a case of the nth root with n = 3 so was never needed here in the first place. Only the square root is really a special case. Thanks for helping me "see outside the box". Glenn L (talk) 02:04, 12 November 2010 (UTC)

EXTERNAL LINKS

I wonder what makes a person so "priviliged" that his/her external-link is allowed to appear at wikipedia. I mean, if Wikipedia does not like EXTERNAL LINKS then WIKIPEDIA SHOULD NOT mantain the external-links section. I think it is an obvious public-outrage for many people to realize that only two or three guys are "priviliged" by wikipedia, i do not see any justice on that. Wikipedia should either mantain the EXTERNAL LINKS in another related page or definitely eliminate all of them. What makes some few guys so "privileged"? What makes some guy to be the judge for selecting only two or three external links? Mirificium (talk) 22:39, 20 March 2010 (UTC)

Cube root on standard calculator

Hi, about this identity:

${\frac {1}{3}}={\frac {1}{2^{2}}}\left(1+{\frac {1}{2^{2}}}\right)\left(1+{\frac {1}{2^{4}}}\right)\left(1+{\frac {1}{2^{8}}}\right)\left(1+{\frac {1}{2^{16}}}\right)\dots .$

I think it's necessary to mention how to obtain it, or at least link to which kind of identity this is. --UAwiki (talk) 12:40, 21 October 2011 (UTC)

The formula can be proven using mathematical induction.

Let $A_{1}={\frac {1}{2^{2}}}$, $A_{2}={\frac {1}{2^{2}}}\left(1+{\frac {1}{2^{2}}}\right)$,$A_{3}={\frac {1}{2^{2}}}\left(1+{\frac {1}{2^{2}}}\right)\left(1+{\frac {1}{2^{4}}}\right)$, and so on.

This means that the formula above is :$A=\lim _{n\to \infty }A_{n}$

We will show that $A_{n}={\frac {1}{3}}\times {\frac {2^{2^{n}}-1}{2^{2^{n}}}}$

It is clear that for n=1 the formula holds because $A_{1}={\frac {1}{3}}\times {\frac {3}{4}}={\frac {1}{4}}$

Now suppose that it holds for n=1, 2, 3, ..., k. Now we will need to show that it holds for k+1:

$A_{k+1}=A_{k}\left(1+1/2^{2^{k}}\right)$

$A_{k+1}={\frac {1}{3}}\times {\frac {2^{2^{k}}-1}{2^{2^{k}}}}\times {\frac {2^{2^{k}}+1}{2^{2^{k}}}}$

$A_{k+1}={\frac {1}{3}}\times {\frac {2^{2^{k+1}}-1}{2^{2^{k+1}}}}$

So all values of $A_{n}$ are correct. It is clear that the limit is A = 1/3. Best regards, Alpertron (talk) 21:54, 23 October 2011 (UTC)

Thanks a lot, this proves the sentence, but I expected to find an indication of where was it deducted from, not all the deduction but a little idea, for example List of mathematical series or any of the Infinite product examples... not just to prove it's correct. Greets --UAwiki (talk) 00:15, 29 October 2011 (UTC)

The infinite product does not come from the identities you cited above. An informal deduction of the formula can be done if you use binary notation:

$A_{1}=0.01$,

$A_{2}=0.01\times 1.01=0.0101$,

$A_{3}=0.0101\times 1.0001=0.01010101$,

$A_{4}=0.01010101\times 1.00000001=0.0101010101010101$,

and so on duplicating the number of "01" on every step.

It is clear that 1/3 = 0.01010101010101...

Best regards, Alpertron (talk) 01:24, 2 November 2011 (UTC)

Unexplained images

The section "Complex numbers" contains the following images:

Plot of the complex cube root together with its two additional leaves. The first picture shows the main branch which is described in the text

Riemann surface of the cube root. One can see how all three leaves fit together

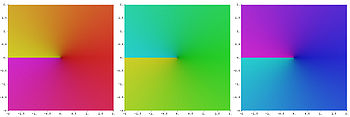
Not already knowing the material presented by the graphs, I have no idea what they are saying. What is measured on the vertical and horizontal axes? What do the shades of colors represent? What are "leaves"? The first picture "shows the main branch" of what? None of this is explained in the article. And "the main branch which is described in the text" must refer to the text of some other article that this image appears in.

Can we just delete these images? Duoduoduo (talk) 15:25, 26 November 2011 (UTC)

Complex principal values

The section which begins "For complex numbers, the principal cube root is usually defined as the cube root that has the greatest real part" is completely unsourced. I have never come across this concept of complex principal cube root anywhere else, so I wonder is it really "usually" defined that way, or only occasionally? Can anybody provide any sources, or justify the statement? The editor who uses the pseudonym "JamesBWatson" (talk) 11:01, 10 July 2018 (UTC)

I found two snippets directly supporting this: [1] and [2]. - DVdm (talk) 11:40, 10 July 2018 (UTC)

And we could use this one as a source:

References

- DVdm (talk) 11:47, 10 July 2018 (UTC)

All cube roots of real numbers and imginary numbers

I am wondering if we can put in a section on the methods of calculating all cube roots of real numbers (including the two complex conjugates) and imaginary numbers (including the two complex conjugates). I was a math minor in college, and I drafted something that can be added as a section to this post:

requested addition

The key to computing all three cube roots of a real number or an imaginary number is to set up a formula of the cube of a complex number, using the coefficients “a” for the “real” component and “b” for the “imaginary”. Then, in the case of a real number, get the formula for each coefficient with the “real” component set to the cube and the “imaginary” component set to 0. In the case of an imaginary number, get the formula for each coefficient with the “real” component set to 0 and the “imaginary” component set to the cube. • The formula for the cube of a complex number: (a + bi) 3 = a3 + 3a2bi + 3ab2i2 + b3i3 • The third term contains “i2” equal to -1, and the fourth term contains “i3” equal to -i: = a3 + 3a2bi - 3ab2 - b3i • Combining “like” terms based on “i”: = a3 - 3ab2 + 3a2bi - b3i • Factor out “i” and group “real” and “imaginary” components: = (a3 - 3ab2) + (3a2b - b3) i • Factor out “a” in the “real” component and “b” in the “imaginary” component: = a(a2 - 3b2) + b(3a2 – b2) i   For a real number k where “imaginary” component = 0: b(3a2 – b2) = 0 (“imaginary” component) Get the values of the coefficients “a” and “b” based on each term above set to 0. A. b = 0 a(a2 – 3b2) = k (“real” component) a(a2 – 0) = k a3 = k a = 3√k B. 3a2 – b2 = 0 v) b2 = 3a2 a(a2 – 3b2) = k (“real” component) a(a2 – 3[3a2]) = k a(a2 – 9a2) = k a(–8a2) = k –8a3 = k a = – 3√k / 2 b2 = 3 (– 3√k / 2 )2 (equation v) above) b2 = 3( (3√k)2 / 4 ) b = ±√3 ( 3√k / 2 ) ⇒ ±3√k / 2 ⋅ √3 The roots are: 3√k, – (3√k / 2) + (3√k / 2 ⋅ √3)i, – (3√k / 2) – (3√k /2 ⋅ √3) i For examples, the following are cube roots for noted values: 3√1 ⇒ 1, – 1/2 + 1/2√3i, – 1/2 – 1/2√3i 3√–1 ⇒ –1, 1/2 + 1/2√3i, 1/2 – 1/2√3i 3√8 ⇒ 2, – 1 + √3i [ – 2/2 + (2/2 ⋅ √3)i ], – 1 – √3i [ – 2/2 – (2/2 ⋅ √3)i ] 3√–8 ⇒ –2, 1 + √3i [– (–2/2) + (2/2 ⋅ √3)i ], 1 – √3i [– (–2/2) – (2/2 ⋅ √3)i ] 3√27 ⇒ 3, – 3/2 + 3/2√3i, – 3/2 – 3/2√3i 3√–27 ⇒ –3, 3/2 + 3/2√3i, 3/2 – 3/2√3i For an imaginary number ki where “real” component = 0: a(a2 - 3b2) = 0 (“real” component) Get the values of the coefficients “a” and “b” based on each term above set to 0. A. a = 0 b(3a2 – b2) = k (“imaginary” component) b(0 – b2) = k – b3 = k b = 3√(–k) = – 3√k B. a2 - 3b2 = 0 w) a2 = 3b2 b(3a2 – b2) = k (“imaginary” component) b(3[3b2] – b2) = k b(9b2 – b2) = k b(8b2) = k 8b3 = k b = 3√k / 2 a2 = 3( 3√k / 2 )2 (equation w) above) a2 = 3( (3√k)2 / 4 ) a = ±√3( 3√k / 2 ) ⇒ ±3√k / 2 ⋅ √3 The roots are: – 3√k i, + (3√k / 2 ⋅ √3) + (3√k / 2)i, – (3√k / 2 ⋅ √3) + (3√k / 2)i For examples, the following are cube roots for noted values: 3√i ⇒ – i [ – (+1)i ], 1/2√3 + 1/2i, – 1/2√3 + 1/2 i 3√–i ⇒ i [ – (–1)i ], 1/2√3 – 1/2i, – 1/2√3 – 1/2 i 3√8i ⇒ –2i [ – (+2)i ], √3 + i [ 2/2√3 + 2/2i ], –√3 + i [ – 2/2√3 + 2/2i ] 3√–8i ⇒ 2i [ – (–2)i ], √3 – i [ 2/2√3 – 2/2i ], –√3 – i [ – 2/2√3 – 2/2i ] 3√27i ⇒ – 3i [ – (+3)i ], 3/2√3 + 3/2i, – 3/2√3 + 3/2i 3√–27i ⇒ 3i [ – (–3)i ], 3/2√3 – 3/2i, – 3/2√3 – 3/2i

Dkf12 (talk) 21:20, 29 March 2020 (UTC)

Wikipedia does not accept our wp:original research. - DVdm (talk) 18:17, 29 March 2020 (UTC)

Without providing anything like above, one will need to leave Wikipedia and search for the solution that will be difficult for most out there. I did that via BING.com and stumbled on an posting on the cube root of "I" that started with solving cubes with the coefficients, and I took it from there. Dkf12 (talk) 18:56, 29 March 2020 (UTC)

I reviewed the section on wp:original research, but what I put is not anything like political opinions or the like. What I stated are mathematical facts. Please note I did not update the post and instead put it all in a talk page, to make sure I clear any hurdles or whatever. Please advise on how I can get a hold of wp:reliable sources, because I believe if this posts delves more on the solutions to get ALL cubes of both real an imaginary numbers, that will be good for people like me who can be called a math nerd! Dkf12 (talk) 19:39, 29 March 2020 (UTC)

Could we use "Template:Original research"? Dkf12 (talk) 19:45, 29 March 2020 (UTC)

See wp:CALC to learn about routine calculations as exceptions to wp:NOR. The above clearly does not qualify. - DVdm (talk) 20:16, 29 March 2020 (UTC)

I read "wp:CALC" and again "wp:NOR", but I disagree. My formulas use nothing but routine calculations. I appreciate how you want to keep a strict set of standards for updates to posts and Wikipedia does have a much better reputation then from even ten years ago or so. But, how can we make the article better? Listen, when someone like me, whom my wife calls a bit of a "math nerd", reads the post, that someone should get a clear explanation of how one calculates all three cube roots of real numbers. The section on "Complex Numbers" uses formulas like "3√r * exp(I* Θ/3 + 2i*e/3)" that was vague to me since I never took complex analysis, and I wonder exaxtly how that leads to "-1 + √3" for one of the cube roots of 8. Dkf12 (talk) 20:48, 29 March 2020 (UTC)

You are now disrupting this talk page—see wp:Talk page guidelines. Second level warning on your talk page. - DVdm (talk) 21:38, 29 March 2020 (UTC)

@DVdm, I apologize. I am trying to get the hang of editing the talk page. Dkf12 (talk) 22:00, 29 March 2020 (UTC)

Please advise if we can make the post more clear, or put a link to a new post with the detail. I am a math major but never took complex analysis, yet I do like to see details. I solved it on my own with the formulas in this talk page and I wanted to share it with others out there. I understand we have an issue with "wp:original research" and I cannot update the post although my formulas use nothing but routine calculations. I just wish we could make the post more clear regardless. Dkf12 (talk) 19:40, 31 March 2020 (UTC)

The only advise we can give you, is to stop trying to share your own work. Wikipedia does not want our own work. - DVdm (talk) 07:20, 1 April 2020 (UTC)

My question now if we can make the article more clear. I had to go to BING to get some insights into how one calculates all cube roots of real numbers without knowing complex analysis. I found a clue and took it from there. Most people out there won't have the mathematical skills I have and won't be able solve the problem. I understand I can't share my work and I never attempted it outside of this talk page. I was just hoping someone can make the article more clear for others out there. Dkf12 (talk) 14:57, 1 April 2020 (UTC)