Jump to content

User:J Doug McLean/sandbox

From Wikipedia, the free encyclopedia

Overview

[edit]
Lift is defined as the component of the aerodynamic force that is perpendicular to the flow direction, and drag is the component that is parallel to the flow direction.

A fluid flowing around the surface of a solid object applies a force on it. It doesn't matter whether the object is moving through a stationary fluid (e.g. an aircraft flying through the air) or whether the object is stationary and the fluid is moving (e.g. a wing in a wind tunnel) or whether both are moving (e.g. a sailboat using the wind to move forward). Lift is the component of this force that is perpendicular to the oncoming flow direction.[1] Lift is always accompanied by a drag force, which is the component of the surface force parallel to the flow direction.

Lift is mostly associated with the wings of fixed-wing aircraft, although it is more widely generated by many other streamlined bodies such as propellers, kites, helicopter rotors, racing car wings, maritime sails, wind turbines, and by sailboat keels, ship's rudders, and hydrofoils in water. Lift is also used by flying and gliding animals, especially by birds, bats, and insects, and even in the plant world by the seeds of certain trees.[2] While the common meaning of the word "lift" assumes that lift opposes weight, lift can be in any direction with respect to gravity, since it is defined with respect to the direction of flow rather than to the direction of gravity. When an aircraft is cruising in straight and level flight, most of the lift opposes gravity.[3] However, when an aircraft is climbing, descending, or banking in a turn the lift is tilted with respect to the vertical.[4] Lift may also act as downforce in some aerobatic manoeuvres, or on the wing on a racing car. Lift may also be largely horizontal, for instance on a sailing ship.

The lift discussed in this article is mainly in relation to airfoils, although marine hydrofoils and propellers share the same physical principles and work in the same way, despite differences between air and water such as density, compressibility, and viscosity.

The flow around a lifting airfoil is a fluid mechanics phenomenon that can be understood on essentially two levels:

1) The level of the rigorous science represented by the mathematical theories, which are based on established laws of physics and represent the flow accurately, but which require solving partial differential equations[5], and

2) The level of qualitative physical explanations, which attempt to interpret the physics without solving equations. Correctly explaining lift in these qualitative terms is difficult because the cause-and-effect relationships involved are subtle[6]. A comprehensive explanation that captures all of the essential aspects is necessarily complex. There are also many simplified explanations. But simplifying the explanation of lift is inherently problematic, and all of the known simplified explanations leave significant parts of the phenomenon unexplained and have other significant flaws[7]. These issues are discussed further in connection with each of the simplified explanations presented below.

Simplified physical explanations of lift on an airfoil

[edit]
A cross-section of a wing defines an airfoil shape.

An airfoil is a streamlined shape that is capable of generating significantly more lift than drag.[8] A flat plate can generate lift, but not as much as a streamlined airfoil, and with somewhat higher drag.

Many different simplified explanations have been proposed.[9][10][11][12][13] Most follow one of two basic approaches, based either on Newton's laws of motion or on Bernoulli's principle.[14][15][16][17]

Explanation based on flow deflection and Newton's laws

[edit]
When an airfoil generates lift, it deflects air downwards, and to do this it must exert a downward force on the air. Newton's third law requires that the air must exert an equal upward force on the airfoil.

An airfoil generates lift by exerting a downward force on the air as it flows past. According to Newton's third law, the air must exert an equal and opposite (upward) force on the airfoil, which is lift.[18][19][20][21]

The airflow changes direction as it passes the airfoil and follows a path that is curved downward. According to Newton's second law, this change in flow direction requires a downward force applied to the air by the airfoil. Then Newton's third law requires the air to exert an upward force on the airfoil; thus a reaction force, lift, is generated opposite to the directional change. In the case of an airplane wing, the wing exerts a downward force on the air and the air exerts an upward force on the wing.[22][23][24][25][26][27]

The downward turning of the flow is not produced solely by the lower surface of the airfoil, and the air flow above the airfoil accounts for much of the downward-turning action (reference?).

This explanation is correct as far as it goes but is incomplete. It doesn't explain how the airfoil can impart downward turning to a much deeper swath of the flow than it actually touches. Furthermore, it doesn't mention that the lift force is exerted by pressure differences, and doesn't explain how those pressure differences are sustained.[28]

Controversy regarding the Coandă effect

[edit]
Main article: Coandă effect

Some versions of the flow-deflection explanation of lift cite the Coandă effect as the reason the flow is able to follow the convex upper surface of the airfoil. The conventional definition in the aerodynamics field is that the Coandă effect refers to the tendency of a fluid jet to stay attached to an adjacent surface that curves away from the flow, and the resultant entrainment of ambient air into the flow.[29][30][31]

More broadly, some consider the effect to include the tendency of any fluid boundary layer to adhere to a curved surface, not just the boundary layer accompanying a fluid jet. It is in this broader sense that the Coandă effect is used by some popular references to explain why airflow remains attached to the top side of an airfoil.[32][33] This is a controversial use of the term "Coandă effect"; the flow following the upper surface simply reflects an absence of boundary-layer separation, thus it is not an example of the Coandă effect.[34][35][36][37]

Explanations based on an increase in flow speed and Bernoulli's principle

[edit]

There are two common versions of this explanation, one based on "equal transit time", and one based on "streamtube pinching".

Equal transit time

[edit]
An illustration of the incorrect equal transit-time explanation of airfoil lift.[9]

The "equal transit time" explanation starts by arguing that the flow over the upper surface is faster than the flow over the lower surface because the path length over the upper surface is longer and must be traversed in equal transit time[38][39][40]. Bernoulli's principle states that under certain conditions increased flow speed is associated with reduced pressure. It is concluded that the reduced pressure over the upper surface results in upward lift[41].

A serious flaw in the equal transit time explanation is that it doesn't correctly explain what causes the flow to speed up[42]. The longer-path-length explanation is simply wrong. No difference in path length is needed, and even when there is a difference, it's typically much too small to explain the observed speed difference [43]. This is because the assumption of equal transit time is wrong. There is no physical principle that requires equal transit time and experimental results show that this assumption is false.[44][45][46][47][48][49] In fact, the air moving over the top of an airfoil generating lift moves much faster than the equal transit theory predicts.[50]. The much higher flow speed over the upper surface can be clearly seen in the animated flow visualization adjacent to The wider flow around the airfoil.

Obstruction of the flow

[edit]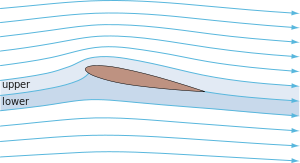
Streamlines and streamtubes around an airfoil generating lift. Note the narrower streamtubes above and the wider streamtubes below.

Like the equal transit time explanation, the "streamtube pinching" explanation argues that the flow over the upper surface is faster than the flow over the lower surface, but gives a different reason for the difference in speed. It argues that the curved upper surface acts as more of an obstacle to the flow, forcing the streamlines to pinch closer together, making the streamtubes narrower. When streamtubes become narrower, conservation of mass requires that flow speed must increase[51]. Reduced upper-surface pressure and upward lift follow from the higher speed by Bernoulli's principle, just as in the equal transit time explanation.

One serious flaw in the streamtube pinching explanation is that it doesn't really explain how streamtube pinching comes about at all, let alone why it's greater over the upper surface than the lower surface. Another flaw is that conservation of mass isn't a satisfying physical reason why the flow would speed up. Really explaining why something speeds up requires identifying the force that makes it accelerate[52].

Issues common to both versions of the Bernoulli-based explanation

[edit]

A serious flaw common to all the Bernoulli-based explanations is that they imply that a speed difference can arise from causes other than a pressure difference, and that the speed difference then leads to a pressure difference, by Bernoulli’s principle. This implied one-way causation is a misconception. The real relationship between pressure and flow speed is a mutual interaction[53].

In addition, as explained below under a more comprehensive physical explanation, producing a lift force requires maintaining pressure differences in both the vertical and horizontal directions. The Bernoulli-only explanations don't explain how the pressure differences in the vertical direction are sustained. That is, they leave out the flow-deflection part of the interaction[54].

Although the two simple explanations above are incorrect, there is nothing incorrect about Bernoulli's principle or the fact that the air goes faster on the top of the wing. In airfoil flows, Bernoulli's principle is applicable[55] to the flow outside the boundary layer and can be used correctly as part of a more complicated explanation of lift.[56]

  1. ^ Cite error: The named reference What is Lift was invoked but never defined (see the help page).
  2. ^ Kulfan (2010)
  3. ^ The amount of aerodynamic lift will be (usually slightly) more or less than gravity depending on the thrust level and vertical alignment of the thrust line. A side thrust line will result in some lift opposing side thrust as well.
  4. ^ Clancy, L. J., Aerodynamics, Section 14.6
  5. ^ Doug McLean Aerodynamic Lift, Part 1: The Science The Physics teacher, November, 2018
  6. ^ Doug McLean Aerodynamic Lift, Part 1: The Science The Physics teacher, November, 2018
  7. ^ Doug McLean Aerodynamic Lift, Part 2: A comprehensive Physical Explanation The Physics teacher, November, 2018
  8. ^ Clancy, L. J., Aerodynamics, Section 5.2
  9. ^ a b "There are many theories of how lift is generated. Unfortunately, many of the theories found in encyclopedias, on web sites, and even in some textbooks are incorrect, causing unnecessary confusion for students." NASA "Incorrect lift theory #1". Aug 16, 2000. Archived from the original on April 27, 2014. Retrieved June 27, 2021.
  10. ^ "Most of the texts present the Bernoulli formula without derivation, but also with very little explanation. When applied to the lift of an airfoil, the explanation and diagrams are almost always wrong. At least for an introductory course, lift on an airfoil should be explained simply in terms of Newton’s Third Law, with the thrust up being equal to the time rate of change of momentum of the air downwards." Cliff Swartz et al. Quibbles, Misunderstandings, and Egregious Mistakes - Survey of High-School Physics Texts THE PHYSICS TEACHER Vol. 37, May 1999 p. 300 [1]
  11. ^ "One explanation of how a wing . . gives lift is that as a result of the shape of the airfoil, the air flows faster over the top than it does over the bottom because it has farther to travel. Of course, with our thin-airfoil sails, the distance along the top is the same as along the bottom so this explanation of lift fails." The Aerodynamics of Sail Interaction by Arvel Gentry Proceedings of the Third AIAA Symposium on the Aero/Hydronautics of Sailing 1971 "Archived copy" (PDF). Archived from the original (PDF) on July 7, 2011. Retrieved July 12, 2011.{{cite web}}: CS1 maint: archived copy as title (link)
  12. ^ "An explanation frequently given is that the path along the upper side of the aerofoil is longer and the air thus has to be faster. This explanation is wrong." A comparison of explanations of the aerodynamic lifting force Klaus Weltner Am. J. Phys. Vol.55 January 1, 1987
  13. ^ "The lift on the body is simple...it's the reaction of the solid body to the turning of a moving fluid...Now why does the fluid turn the way that it does? That's where the complexity enters in because we are dealing with a fluid. ...The cause for the flow turning is the simultaneous conservation of mass, momentum (both linear and angular), and energy by the fluid. And it's confusing for a fluid because the mass can move and redistribute itself (unlike a solid), but can only do so in ways that conserve momentum (mass times velocity) and energy (mass times velocity squared)... A change in velocity in one direction can cause a change in velocity in a perpendicular direction in a fluid, which doesn't occur in solid mechanics... So exactly describing how the flow turns is a complex problem; too complex for most people to visualize. So we make up simplified "models". And when we simplify, we leave something out. So the model is flawed. Most of the arguments about lift generation come down to people finding the flaws in the various models, and so the arguments are usually very legitimate." Tom Benson of NASA's Glenn Research Center in an interview with AlphaTrainer.Com "Archived copy - Tom Benson Interview". Archived from the original on April 27, 2012. Retrieved 26 July 2012.
  14. ^ McLean, Doug (2012). Understanding Aerodynamics: Arguing from the Real Physics. p. 281. ISBN 978-1119967514. Another argument that is often made, as in several successive versions of the Wikipedia article "Aerodynamic Lift," is that lift can always be explained either in terms of pressure or in terms of momentum and that the two explanations are somehow "equivalent." This "either/or" approach also misses the mark.
  15. ^ "Both approaches are equally valid and equally correct, a concept that is central to the conclusion of this article." Charles N. Eastlake An Aerodynamicist’s View of Lift, Bernoulli, and Newton THE PHYSICS TEACHER Vol. 40, March 2002 "Archived copy" (PDF). Archived from the original (PDF) on April 11, 2009. Retrieved 10 September 2009.{{cite web}}: CS1 maint: archived copy as title (link)
  16. ^ Ison, David, "Bernoulli Or Newton: Who's Right About Lift?", Plane & Pilot, archived from the original on September 24, 2015, retrieved January 14, 2011
  17. ^ Cite error: The named reference ReferenceA was invoked but never defined (see the help page).
  18. ^ "...the effect of the wing is to give the air stream a downward velocity component. The reaction force of the deflected air mass must then act on the wing to give it an equal and opposite upward component." In: Halliday, David; Resnick, Robert, Fundamentals of Physics 3rd Ed., John Wiley & Sons, p. 378
  19. ^ Anderson and Eberhardt (2001)
  20. ^ Langewiesche (1944)
  21. ^ "When air flows over and under an airfoil inclined at a small angle to its direction, the air is turned from its course. Now, when a body is moving in a uniform speed in a straight line, it requires force to alter either its direction or speed. Therefore, the sails exert a force on the wind and, since action and reaction are equal and opposite, the wind exerts a force on the sails." In: Morwood, John, Sailing Aerodynamics, Adlard Coles Limited, p. 17
  22. ^ "Lift is a force generated by turning a moving fluid... If the body is shaped, moved, or inclined in such a way as to produce a net deflection or turning of the flow, the local velocity is changed in magnitude, direction, or both. Changing the velocity creates a net force on the body." "Lift from Flow Turning". NASA Glenn Research Center. May 27, 2000. Archived from the original on July 5, 2011. Retrieved June 27, 2021.
  23. ^ "Essentially, due to the presence of the wing (its shape and inclination to the incoming flow, the so-called angle of attack), the flow is given a downward deflection. It is Newton’s third law at work here, with the flow then exerting a reaction force on the wing in an upward direction, thus generating lift." Vassilis Spathopoulos - Flight Physics for Beginners: Simple Examples of Applying Newton’s Laws The Physics Teacher Vol. 49, September 2011 p. 373 [2]
  24. ^ "The main fact of all heavier-than-air flight is this: the wing keeps the airplane up by pushing the air down." In: Langewiesche - Stick and Rudder, p. 6
  25. ^ "Birds and aircraft fly because they are constantly pushing air downwards: L = Δp/Δt where L= lift force, and Δp/Δt is the rate at which downward momentum is imparted to the airflow." Flight without Bernoulli Chris Waltham THE PHYSICS TEACHER Vol. 36, Nov. 1998 "Archived copy" (PDF). Archived (PDF) from the original on September 28, 2011. Retrieved 4 August 2011.{{cite web}}: CS1 maint: archived copy as title (link)
  26. ^ Clancy, L. J.; Aerodynamics, Pitman 1975, p. 76: "This lift force has its reaction in the downward momentum which is imparted to the air as it flows over the wing. Thus the lift of the wing is equal to the rate of transport of downward momentum of this air."
  27. ^ "...if the air is to produce an upward force on the wing, the wing must produce a downward force on the air. Because under these circumstances air cannot sustain a force, it is deflected, or accelerated, downward. Newton's second law gives us the means for quantifying the lift force: Flift = m∆v/∆t = ∆(mv)/∆t. The lift force is equal to the time rate of change of momentum of the air." Smith, Norman F. (1972). "Bernoulli and Newton in Fluid Mechanics". The Physics Teacher. 10 (8): 451. Bibcode:1972PhTea..10..451S. doi:10.1119/1.2352317.
  28. ^ Doug McLean Aerodynamic Lift, Part 2: A comprehensive Physical Explanation The Physics teacher, November, 2018
  29. ^ Cite error: The named reference auerbach was invoked but never defined (see the help page).
  30. ^ Denker, JS, Fallacious Model of Lift Production, archived from the original on March 2, 2009, retrieved 18 August 2008
  31. ^ Wille, R.; Fernholz, H. (1965), "Report on the first European Mechanics Colloquium, on the Coanda effect", J. Fluid Mech., 23 (4): 801, Bibcode:1965JFM....23..801W, doi:10.1017/S0022112065001702
  32. ^ Cite error: The named reference scotteberhart was invoked but never defined (see the help page).
  33. ^ Cite error: The named reference raskin was invoked but never defined (see the help page).
  34. ^ Auerbach (2000)
  35. ^ Denker (1996)
  36. ^ Wille and Fernholz(1965)
  37. ^ White, Frank M. (2002), Fluid Mechanics (5th ed.), McGraw Hill
  38. ^ Burge, Cyril Gordon (1936). Encyclopædia of aviation. London: Pitman. p. 441. "… the fact that the air passing over the hump on the top of the wing will have to speed up more than that flowing beneath the wing, in order to arrive at the trailing edge in the same time."
  39. ^ Illman, Paul (2000). The Pilot's Handbook of Aeronautical Knowledge. New York: McGraw-Hill. pp. 15–16. ISBN 0071345191. When air flows along the upper wing surface, it travels a greater distance in the same period of time as the airflow along the lower wing surface."
  40. ^ Dingle, Lloyd; Tooley, Michael H. (2005). Aircraft engineering principles. Boston: Elsevier Butterworth-Heinemann. p. 548. ISBN 0-7506-5015-X. The air travelling over the cambered top surface of the aerofoil shown in Figure 7.6, which is split as it passes around the aerofoil, will speed up, because it must reach the trailing edge of the aerofoil at the same time as the air that flows underneath the section."
  41. ^ "The airfoil of the airplane wing, according to the textbook explanation that is more or less standard in the United States, has a special shape with more curvature on top than on the bottom; consequently, the air must travel farther over the top surface than over the bottom surface. Because the air must make the trip over the top and bottom surfaces in the same elapsed time ..., the velocity over the top surface will be greater than over the bottom. According to Bernoulli's theorem, this velocity difference produces a pressure difference which is lift." Bernoulli and Newton in Fluid Mechanics Norman F. Smith The Physics Teacher November 1972 Volume 10, Issue 8, p. 451 [3] [permanent dead link‍]
  42. ^ Doug McLean Aerodynamic Lift, Part 2: A comprehensive Physical Explanation The Physics teacher, November, 2018
  43. ^ Craig(1997)
  44. ^ "Unfortunately, this explanation [fails] on three counts. First, an airfoil need not have more curvature on its top than on its bottom. Airplanes can and do fly with perfectly symmetrical airfoils; that is with airfoils that have the same curvature top and bottom. Second, even if a humped-up (cambered) shape is used, the claim that the air must traverse the curved top surface in the same time as it does the flat bottom surface...is fictional. We can quote no physical law that tells us this. Third—and this is the most serious—the common textbook explanation, and the diagrams that accompany it, describe a force on the wing with no net disturbance to the airstream. This constitutes a violation of Newton's third law." Bernoulli and Newton in Fluid Mechanics Norman F. Smith The Physics Teacher November 1972 Volume 10, Issue 8, p. 451 "Archived copy". Archived from the original on March 17, 2012. Retrieved 4 August 2011.{{cite web}}: CS1 maint: archived copy as title (link)
  45. ^ Anderson, David (2001), Understanding Flight, New York: McGraw-Hill, p. 15, ISBN 978-0-07-136377-8, The first thing that is wrong is that the principle of equal transit times is not true for a wing with lift.
  46. ^ Anderson, John (2005). Introduction to Flight. Boston: McGraw-Hill Higher Education. p. 355. ISBN 978-0072825695. It is then assumed that these two elements must meet up at the trailing edge, and because the running distance over the top surface of the airfoil is longer than that over the bottom surface, the element over the top surface must move faster. This is simply not true
  47. ^ "Archived copy". Archived from the original on June 30, 2012. Retrieved 10 June 2012.{{cite web}}: CS1 maint: archived copy as title (link) Cambridge scientist debunks flying myth UK Telegraph 24 January 2012
  48. ^ Flow Visualization. National Committee for Fluid Mechanics Films/Educational Development Center. Archived from the original on October 21, 2016. Retrieved January 21, 2009. A visualization of the typical retarded flow over the lower surface of the wing and the accelerated flow over the upper surface starts at 5:29 in the video.
  49. ^ "...do you remember hearing that troubling business about the particles moving over the curved top surface having to go faster than the particles that went underneath, because they have a longer path to travel but must still get there at the same time? This is simply not true. It does not happen." Charles N. Eastlake An Aerodynamicist’s View of Lift, Bernoulli, and Newton THE PHYSICS TEACHER Vol. 40, March 2002 PDF Archived April 11, 2009, at the Wayback Machine
  50. ^ "The actual velocity over the top of an airfoil is much faster than that predicted by the "Longer Path" theory and particles moving over the top arrive at the trailing edge before particles moving under the airfoil." Glenn Research Center (Aug 16, 2000). "Incorrect Lift Theory #1". NASA. Archived from the original on April 27, 2014. Retrieved June 27, 2021.
  51. ^ J. D. Anderson (2008), section 5.19
  52. ^ Doug McLean Understanding Aerodynamics, section 7.3.1.5, Wiley, 2012
  53. ^ Doug McLean Aerodynamic Lift, Part 2: A comprehensive Physical Explanation The Physics teacher, November, 2018
  54. ^ Doug McLean Aerodynamic Lift, Part 2: A comprehensive Physical Explanation The Physics teacher, November, 2018
  55. ^ Doug McLean Aerodynamic Lift, Part 1: The Science The Physics teacher, November, 2018
  56. ^ "There is nothing wrong with the Bernoulli principle, or with the statement that the air goes faster over the top of the wing. But, as the above discussion suggests, our understanding is not complete with this explanation. The problem is that we are missing a vital piece when we apply Bernoulli’s principle. We can calculate the pressures around the wing if we know the speed of the air over and under the wing, but how do we determine the speed?" How Airplanes Fly: A Physical Description of Lift David Anderson and Scott Eberhardt "Archived copy". Archived from the original on January 26, 2016. Retrieved 26 January 2016.{{cite web}}: CS1 maint: archived copy as title (link)
Retrieved from "https://wiki.riteme.site/w/index.php?title=User:J_Doug_McLean/sandbox&oldid=1042007729"