Jump to content

Talk:Monty Hall problem/Archive 31

Page contents not supported in other languages.
From Wikipedia, the free encyclopedia
Archive 25←Archive 29Archive 30Archive 31Archive 32Archive 33→Archive 35

Devlin's probability theory

I had a better look at the 2005 article of Devlin, that Richard added to the literature. In the proposed variant Devlin comes to the conclusion that the second player wins with probability 1/3 and loses also with probability 1/3. Got it! Nijdam (talk) 16:27, 18 September 2012 (UTC)

Nijdam, why are you showing here Devlin's variant of "the forgetful host" who does not know where the prize is, and subsequently, in opening one of his two doors at random, in 1/3 inevitably will show the prize, and in that 1/3 destroys the possibility to win by switching, while in the remaining 2/3 ("car:goat" and "goat:car") the chances of the two still closed doors are "1:1" now. What is your argument? The "second player" had a choice only between 2 of 3 doors, both with 1/3 chance to hide the prize. Gerhardvalentin (talk) 17:23, 18 September 2012 (UTC)
@Nijdam: Yes, here the text of an email which I wrote to Devlin at 8th May 2012:
<start email text>
I think that your following phrase for the "variant game" is wrong:
The probability that she loses is likewise 1/2 x 2/3 = 1/3. And that's the probability that you win if you switch. Exactly the same as if you did not.
My consideration:
The probability that "she" wins is 1/3; and - of course - the probability that she loses is 2/3.
And the probability that "you win if you switch" is 1/2, not 1/3. "Exactly the same as if you did not".
The difference between the (well formulated) original game and the variant game is the following:
In the original game the probability that the host opens door t is twice as much if the contestant has chosen a door with a goat (1/3 * 1 = 1/3) as if she has chosen the car (1/3 *1/2 = 1/6)).
In the variant game the probability that the host opens door t in both cases is 1/3 * 1/2 = 1/6.
The "shock" in the variant game only arises if you believe in the following argumentation for the original game:
[1] The probability that the prize is behind door B or C (i.e., not behind door A) is 2/3.
[2] The prize is not behind door C.
Combining these two pieces of information, you conclude that the probability that the prize is behind door B is 2/3.
Indeed you could apply this argumentation to the variant game - which leads to the proof that the argumentation is wrong. The real reason why in the original game the probability for switching is 2/3 is that (1/3)/(1/3 + 1/6) = 2/3. For the variant game we have (1/6)/(1/6 + 1/6) = 1/2.
<end email text>
--Albtal (talk) 17:57, 18 September 2012 (UTC)
@Albtal: did Devlin answer? I wrote him twice and got no response. Nijdam (talk) 20:19, 18 September 2012 (UTC)
He didn't answer. And I only placed this comment here after having said good bye above, because my already existing email exactly matches your hint (and more). So concerning the following comments here: No ban is necessary for me. But since I am here once again I allow myself to write the following last lines here: In this German article we can find an analogous variant as Übungsaufgabe 1, placed there as a hint, that the consent to the 2/3-solution of the original MvS-problem almost always was not only superficial but wrong; and that the problem, combined with the 2/3-solution, which had been going around the world as a "paradox", really was a joke.--Albtal (talk) 06:34, 19 September 2012 (UTC)

NO ORIGINAL RESEARCH PLEASE. NO CITING EMAILS FROM EXPERTS, PAPERS YOU FOUND IN YOUR GRANNY'S ATTIC, OR PERSONAL GNOSIS FROM YOUR TALKING TOASTER. Elen of the Roads (talk) 20:53, 18 September 2012 (UTC)

What's the problem? I don't think Albtal has the intention to show this in the article itself. Nijdam (talk) 21:02, 18 September 2012 (UTC)
See below. Or try citing personal emails on any other talkpage and see where it gets you. Elen of the Roads (talk) 21:14, 18 September 2012 (UTC)
Re. Nijdam's original post in this thread: Yes, that is an inexplicable mistake. It probably shows the hazards of not using a copy editor who knows the subject matter. However, like Gerhardvalentin, I fail to see the relevance of dwelling on the variant problem. ~ Ningauble (talk) 21:18, 18 September 2012 (UTC)
Well, I don't comment on GerhardValentine, but the relevance is the reliability of Devlin as a source. Nijdam (talk) 21:29, 18 September 2012 (UTC)
Only in the respect that it potentially shows a lack of proper peer review, and not just a dumb typesetter and a sloppy proofreader. It would be a good source for how vos Savant isn't the only one to publish duff stuff where this problem is concerned. Elen of the Roads (talk) 21:57, 18 September 2012 (UTC)
Keith Devlin is a reliable source according to Wikipedia standards: a mathematician and popularizer of mathematics (but not a probabilist or a statistician or a games theorist). His pretty little solution -- not an academic publication but a chatty newsletter column -- to MHP had a tiny little gap in it. He got a lot of letters telling him he'd screwed up so he got scared and gave the full Bayes calculation which at least (as he said) is a reliable way to get the right answer without actually using your head, provided you are careful and patient. However, the little gap in his proof is child's play to patch and Richard Gill did that in articles on Citizendium and StatProb; the latter had peer review. No doubt other sources did it too. Richard Gill (talk) 07:14, 19 September 2012 (UTC)
So much then for Wikipedia standards. The itsy bitsy tiny winy little gap is in fact nothing more than the complete solution. Indeed child's play for a statistician, nothing to brag about. However, combining doors is highly misleading, as the basic idea is wrong. Have a look at the combining purses. Nijdam (talk) 09:21, 19 September 2012 (UTC)
On Wikipedia, Wikipedia standards rule. On the Annals of Statistics, Annals of Statistics standards rule. Here we're on Wikipedia. Next: Nijdam, your opinion is that the basic idea of combining doors is wrong. My opinion is that it's brilliant. On Wikipedia, neither of our opinions has any relevance whatsoever. "Truth" is not relevant. If you want the Truth to influence what's in Wikipedia, go and publush papers expounding the truth in major academic journals, and then sit back and wait 20 years for this info to trickle down into the standard textbooks. Richard Gill (talk) 19:04, 19 September 2012 (UTC)
Well, Devlin's idea is brilliant as is the magician's trick to produce a rabbit out of an empty high hat. Nijdam (talk) 10:13, 20 September 2012 (UTC)

Proposed text for Solution section

While there's widespread interest, can I get comments on the following proposed text (motivated to some extent by Linas's comments above)?

Proposed text for Solution section

There are two main approaches to solving the Monty Hall problem. One is to compare a strategy of switching (always switching to whichever door the host doesn't open) with a strategy of staying. The other is to evaluate the conditional probability the car is behind door 2 given the player picks door 1 and the host opens door 3. Because the problem is symmetrical, these two approaches show (and must show) the same result - switching wins the car 2/3 of the time.

Most popular sources present solutions comparing a strategy of always switching with a strategy of always staying with the player's initial choice. For example, the solution presented by vos Savant in Parade (vos Savant 1990b) shows the three possible arrangements of one car and two goats behind three doors and the result of staying or switching after initially picking door 1 in each case:

Door 1 Door 2 Door 3 result if switching result if staying
Car Goat Goat Goat Car
Goat Car Goat Car Goat
Goat Goat Car Car Goat

A player who stays with the initial choice wins in only one out of three of these equally likely possibilities, while a player who switches wins in two out of three. Thus, a player who picks door 1 should switch to whichever of door 2 or door 3 the host doesn't open, and by doing so will win the car with probability 2/3.

The other main approach to solving the problem, used primarily in academic sources, is to treat it as a conditional probability problem. The probability the car is behind any one of the doors before the host opens a door is 1/3. The probability the car is behind Door 2 after the player picks Door 1 and the host opens Door 3 is the conditional probability the car is behind Door 2, usually written as: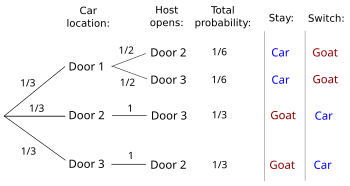

Tree showing the probability of every possible outcome if the player initially picks Door 1

This conditional probability can be determined referring to the figure below, or to an equivalent decision tree as shown to the right (Chun 1991; Grinstead and Snell 2006:137-138). In each figure, it is assumed that the contestant initially chooses door 1. The probability can also be formally derived as in the mathematical formulation shown in the appendix.

If the player picks door 1, the car is behind door 2 and the host opens door 3 with joint probability 1/3. The car is behind door 1 and the host opens door 3 with joint probability 1/6. These are the only possibilities given the player picks door 1 and the host opens door 3. Therefore, the conditional probability the car is behind door 2 is (1/3)/(1/3 + 1/6), which is 2/3. (Morgan et al. 1991) The intuition behind this solution is that the host must always open Door 3 if the car is behind Door 2, but opens Door 3 only half the time the car is behind Door 1 - so a player who switches wins twice as often as a player who doesn't.

Car hidden behind door 3 Car hidden behind door 1 Car hidden behind door 2
Player initially picks door 1
Player has picked door 1 and the car is behind door 3 Player has picked door 1 and the car is behind it Player has picked door 1 and the car is behind door 2
Host must open door 2 Host randomly opens door 2 Host randomly opens door 3 Host must open door 3
Host must open door 2 if the player picks door 1 and the car is behind door 3 Host opens door 2 half the time if the player picks door 1 and the car is behind it Host opens door 3 half the time if the player picks door 1 and the car is behind it Host must open door 3 if the player picks door 1 and the car is behind door 2
Probability 1/3 Probability 1/6 Probability 1/6 Probability 1/3
Switching wins Switching loses Switching loses Switching wins
If the host has opened door 3, these cases have not happened If the host has opened door 3, switching wins twice as often as staying

Comments on the proposed text

This is intended to follow the Proposal 2 approach (both "simple" and "conditional" in one Solution section, with neither presented as "more correct" than the other). In the article this would follow the "Extended description" section (replacing the entirety of what is now the "Solutions" section), and like any other article content here would (of course) be able to be edited at will. If you read this, could you at least add a quick "I like it" (it's in the right ballpark, you'd be able to live with this without a complete rewrite), "I hate it" (needs to be completely rewritten), "meh" (might be OK - doesn't thrill you but doesn't offend you either) sort of comment? Thanks. -- Rick Block (talk) 03:44, 22 September 2012 (UTC)

  • I like it <user sig here>
  • I hate it <user sig here>
  • meh <user sig here>

I'll say I like it, because it's broadly speaking in the right direction, but there's nevertheless much that I would change in it. It's good in that it attempts to explain the sources' POVs about the "standard MHP" in a coherent and understandable manner with due weight, and that's the right thing to do. It's the "attempts" part that I have some comments about. In addition to what I said in the Proposal: Rewrite the section on conditional probability! section, I'll say these:

  • The "strategy" thing doesn't actually distinguish between conditional and unconditional solutions, and readers will probably fail to understand it that way. You can follow a "always switch" strategy regardless of whether someone is going to analyze your chances of winning using an unconditional or conditional probability calculation. I'll reiterate that explanation using a simulation is probably the only way to get the difference understood properly.
  • "The problem is symmetrical" is not enlightening. Symmetrical how? Why does that matter? Something along the lines "It can be shown (see section [symmetry section]) that the two approaches necessarily produce the same result, because the problem and the answer remain the same if the door numbers are permuted in the problem description, making the door numbers irrelevant." would be more like it, but do we really need to even add the "because" part here and is it helpful? "It is shown in section [symmetry] that ..." would suffice, IMO.
  • The P(... | ...) is not used anywhere so mentioning it is rather pointless.
  • Here it is even more painfully obvious that the conditional solution should only have one diagram (I prefer the decision tree), as it takes up so much space in comparison to the MvS table.
  • Wikipedia article with an appendix? Ow. Rather just link to the formal solution section, and that can come as the last sentence in the text, just like "Further Reading" is usually at the bottom.

The material here is not rocket science. It can be written in a way understandable to anyone. -- Coffee2theorems (talk) 11:51, 22 September 2012 (UTC)

As it stands, it's way too complicated to be the section you use to explain the answer. Coffee2theorems I think is right that it can be written much better, so in addition to Coffee's points above, all of which are good, here's what doesn't work for me

  • putting the conditional probability tree and vos Savant's table near to each other, because they appear to contradict each other. Of course the little 1/6 and 1/3s mean that it doesn't, but put next to each other it looks as if it does, because one appears to show there is no advantage to switching. Moving them apart or laying the table out differently would both work, but I gather the table layout appears in books that way, so a redraw may suffer from the accusation of OR.
  • I found the picture table to be just incomprehensible. Putting a section in with a note 'this hasn't happened' isn't at all clear. You would need to sit down with a pencil and paper and redraw it yourself to understand it.
  • The text from "If the player picks door 1..." is equally unclear. If that's how sources write it, then we have a problem, because the average reader won't understand either 'joint probability' or 'intuition' (indeed, I'm not convinced intuition is at all the right word here, unless it has some specific meaning in maths).
  • No appendices. But the Bayes write out can comfortably go in another section.

So, too complicated as is, but I'm sure I can see the light. Elen of the Roads (talk) 17:30, 22 September 2012 (UTC)

  • I hate it because it is far too complicated.
Proposal: instead of the tree, imo it was better to show the very clear answer of this table in en.citizendium.org, section "Explicit Computations", btw this article having been cited repeatedly by academic sources meanwhile. Gerhardvalentin (talk) 18:01, 22 September 2012 (UTC)

I suck at markup, but I agree with Gerhardvalentin about using a table not a tree. The contrast between the two solution then looks something like

Your door The door Monty opens The other door has the result if switching result if staying
Car Goat Goat Goat Car
Goat Goat Car Car Goat
Goat Goat Car Car Goat



Door 1 Door 2 Door 3 result if switching result if staying
Your door has the car Monty opens to show a goat has a goat goat car
has a goat Monty opens to show a goat
Your door has a goat Monty opens to show a goat has the car car goat
Your door has a goat has the car Monty opens to show a goat Car Goat

If you take table 2 and put the probabilities in, it has a chance of making sense.Elen of the Roads (talk) 18:23, 22 September 2012 (UTC)

Strange, to me the tree is much clearer than a table — it contains the same information in essentially the same format, but the structure is visually more obvious. I'd just bold the two relevant paths (and corresponding texts) so that the reader knows what to compare with what, and add more explanation to the figure text. Also, the citizendium version's use of 1/3×1/2 and 1/3×1 instead of 1/6 and 1/3 is actually a good idea, as it shows how the values were obtained and makes it clearer that one is twice as big as the other.
If one were really going for eye candy, one could make a separate, animated figure of a simulation, where the path taken by the simulation is slowly highlighted on the tree and next to it a log of what happened and a running tally shows how the winning frequency approaches 2/3. That might be asking for too much, though :) -- Coffee2theorems (talk) 18:51, 22 September 2012 (UTC)
You're going to need a better coder than me (not hard!). I think the tree can be improved - it's the present format that troubles me. Is the use of 1/3*1/2 an issue source wise (surely not - anyone using 1/6 must have derived it). The more you start from the beginning, the clearer it is. Incidentally, is table 1 pure OR or has a published source phrased it that way (no door numbers, just 'your door', 'Monty's door' and 'the other door') Elen of the Roads (talk) 19:20, 22 September 2012 (UTC)
The content in Elen's first table is at least usually presented the way vos Savant did, i.e. with door numbers (which is the table already in the article). Using door numbers both connects it to the example given in the problem statement ("You pick a door, say No. 1, ...") and makes it clear why there are three rows (the repeating rows 2 and 3 seem very strange). Vos Savant's table makes it very difficult to see what happens in the case where the host opens door 3. The point of the conditional solution is to address this specific case, so perhaps a table like this (similar to tables I've suggested before):
Situation before the host opens a door Situation after the host opens a door
Door 1 (your door) Door 2 Door 3 probability host opens Door 2 host opens Door 3
probability result if switching probability result if switching
Car Goat Goat 1/3 1/3 x 1/2 = 1/6 Goat 1/3 x 1/2 = 1/6 Goat
Goat Car Goat 1/3 0 N/A 1/3 Car
Goat Goat Car 1/3 1/3 Car 0 N/A
This table is equivalent to the tree diagram (and the large figure as well). It shows that whatever door you see the host open, you win with probability 1/6 if you stay with your initial choice but with probability 1/3 (twice as often) if you switch (which, expressed as conditional probabilities are 1/3 and 2/3). If you're only interested in the "host opens door 3 case" you ignore the "host opens door 2" column. -- Rick Block (talk) 21:43, 22 September 2012 (UTC)

How has that been working out for you?

Looking at the recent history of this talk page, I am once again struck by the large number of comments and with the net result of pretty much nobody shifting their position even slightly. (This, of course, includes me and my comments.) This has been going on for ten years and 1.3 million talk page words. Is there anyone here who can make a reasonable argument that, if only we spend another year and put another 100,000 words on this talk page, the dispute will be resolved? Now obviously, I am right, anyone who disagrees with me is wrong, and if they would only realize this and agree with me the dispute would be resolved. Alas, there are a bunch of misguided individuals who imagine that they are right, I am wrong, and everyone should agree with them! How crazy is that? I know what to do -- I will post another thousand or so words. That should do it! Just because it didn't work the last thousand or so times, is that any reason not to keep trying it forever?

So, other than talking at each other until we all die of old age, does anyone have any idea at all of a way out of this mess? --Guy Macon (talk) 19:25, 17 September 2012 (UTC)

It has worked very badly for me to. I thought the plan was to ask users to chose between two ways of ending the long-standing conflict, which is only about the validity of the 'simple' solutions. Instead people started bringing up all sorts of other issues, which may be important, but are not the issue that has been dogging the article for years and are issues which will probably be resolved perfectly well by the normal WP processes of discussion and cooperative editing.
Hardly anyone seemed to understand the problem that Rick and I were trying to solve which was, 'How do we deal the dispute between two groups of editors, one who thing the simple solutions are OK and the other group who think they are incomplete/answer the wrong question?'. Note, we were not trying to resolve the dispute itself for, as you say, not a single person has changed their mind on that subject, rather we were trying to find a way that the two groups could agree to differ but still edit the article cooperatively. That process has clearly failed.
The only way forward now is to ask users to decide on the dispute itself, are the simple solutions (individually if you like) 'satisfactory'. satisfactory in this context implying that they can be used freely within the article without disclaimers or proximity to 'better' solutions.
Accept strictly only 'yes' or 'no' answers with no comments at all and count the supporters for each side. Abstainers should simply not vote or comment. All comments, in fact anything other than the words 'yes' or 'no', should be deleted or moved to a 'Invalid replies' section. Martin Hogbin (talk) 20:00, 17 September 2012 (UTC)
There are two separate issues here; the RfC and the outside-the-RfC comments.
I actually think the RfC is going fairly well. Yes, there are irrelevant comments, but I plan on asking an experienced, uninvolved admin to evaluate all the comments and post her/his finding as to what the consensus is, then I am going to ask another couple of experienced and uninvolved admins to review that finding and confirm that it is good. That way nobody has cause to question the result. I will make sure that these admins are well aware of the specific question we are addressing and I am sure that they will have no problem separating the wheat from the chaff, so I see no need to remove any comments. The good news is that each person is limited to 500 words in the RfC. I have seen enough of you (Martin and Rick) in action -- both reasonable people who want the best for the encyclopedia but disagree on what is best -- that I am fairly confident that this will put an end to the Rick/Martin dispute once and for all.
As for the outside-the-RfC comments, as I expected, my efforts to widely advertise this among those with an interest in math and those with an interest in dispute resolution brought in a bunch of comments from folks who are new to the topic or who had given up long ago. Also as I expected, there has been Yet Another Wall Of Text That Accomplishes Nothing. Everybody has an opinion, nobody has been convinced to change their position, and no faction seems to have a strong consensus. The good news is that the Martin/Rick dispute can be resolved while ignoring all comments that are not in the RfC. The bad news is that whoever comes out of that process as the "winner" will be faced with a new dispute with a new set of editors. --Guy Macon (talk) 23:30, 17 September 2012 (UTC)
I'm not sure if Martin is being tongue in cheek above, but if he's seriously suggesting we should attempt to resolve this dispute with a poll such as he suggests IMO he seriously misunderstands Wikipedia policies. The question is not and hasn't been for several years what editors think about "simple" solutions vs. "conditional" solutions, but how the article should present what sources say about the problem and its solution. The orgy of WP:OR this RfC has spawned above (and Guy's clearly tongue in cheek comments starting this thread) is completely consistent with a nearly universal refusal to approach this issue from a perspective of what sources say, as opposed to what editors think, about the problem. -- Rick Block (talk) 02:02, 18 September 2012 (UTC)
I am being perfectly serious. You always insist an talking as though the sources support your POV on this subject; they do not.
Existing WP policies and dispute resolution methods have failed to resolve the longstanding argument so now is the time to try something new. I am not remotely suggesting that we abandon any of the WP core policies, especially verifiability but community consensus has always been the final arbiter in content disputes. Asking for consensus on a complex question, as we have just done, has failed completely. Now is the time to ask for consensus on a very simple question. Editors will be free to look at what the sources say in making their yes/no decisions but there simply is no useful purpose in discussing the matter here, we have done that for years and got nowhere. Martin Hogbin (talk) 08:34, 18 September 2012 (UTC)
Guy, if you can extract a clear consensus for one of the proposals from the replies then then good on you, that would suit me fine. I make it 9 for proposal 1, 6, for proposal 2, and 10 other, which even from my perspective must be a 'no consensus', which leaves us with the question, 'What now?'. Martin Hogbin (talk) 08:46, 18 September 2012 (UTC)
Remember, The RfC is a long way from day 30, so don't assume we have all the data. As for the "What now?" question, "If either proposal is accepted that will be a consensus decision. If neither proposal is accepted then anyone else is quite free to make their own proposal and have an RfC on it." (Quote from Martin Hogbin, posted here at 23:40, 8 August 2012) Perhaps someone else can write an RfC that ends in a consensus.
Certainly a "no consensus" will put some arguments to bed. Want to claim that the majority supports your position? Not if the result was no consensus. Want to claim that you are following the sources and the other fellow isn't? You presented that argument to multiple editors and there was no consensus that it was a compelling argument. This, of course presumes that we know what the result will be... --Guy Macon (talk) 14:01, 18 September 2012 (UTC)
No. that is completely wrong. No consensus does not mean consensus not to. Martin Hogbin (talk) 14:34, 18 September 2012 (UTC)
I thought that one brilliant original suggestion did come out of all this discussion: don't call a solution a solution, call it an argument, approach, guide to understanding. At the same time the material on the Bayes approach should be made a whole lot more accessible, and it should not be presented in a confrontational way ("now here is the proper way to solve the problem"). So the extremists on both sides give a little, and we have a neutral framework within which any editor with knowledge of relevant sources can work to improve the article locally. Richard Gill (talk) 12:20, 18 September 2012 (UTC)
I see no reason why we should not use the term 'solution' for the simple arguments but if calling them 'arguments', for example, allows us to structure the article sensibly then I would not object. I would object if we gave the impression that these arguments were in any way defective or second rate though. Martin Hogbin (talk) 14:34, 18 September 2012 (UTC)
Richard's suggestion is actually fairly close to Proposal 2 (which, again, does not say anything like "conditional solutions are to be presented as the only correct approach"). Martin's claim above that I "always insist an talking as though the sources support your POV on this subject" is incorrect. What I insist is that there are lots of sources from the academic field of probability (a preponderance, I claim) that present only conditional solutions, and that there are numerous (I've cited 6, but there are more) that are critical of "simple" solutions. Proposal 2 does not say we include what the critical sources say early in the article, but that we include a conditional solution and present both "simple" and "conditional" solutions as equally correct. If we only present "simple" solutions early in the article, we're completely ignoring the sources that criticize these kinds of solutions and taking an editorial stance that these sources are wrong. We don't have to say in the article every time a "simple" solution is presented that there are sources that criticize such solutions, but the article can't present "simple" solutions as if they are universally understood to be complete and correct without taking the POV that sources that disagree with this are wrong. Presenting both "simple" and "conditional" as simply two different, even complementary, approaches (neither "more correct" than the other) allows us to remain neutral about this. Presenting just one (whichever one it is) is not neutral. -- Rick Block (talk) 15:08, 18 September 2012 (UTC)
I find it difficult to reconcile the claim the one of the proposals in the RfD is more neutral with the (so far) lack of a consensus among the commentators that this is so. --Guy Macon (talk) 15:31, 18 September 2012 (UTC)

Here we are, a week later, and still multiple editors are adding text to the Great Wall Of Text and making what mostly appear to be reasonable arguments.

And the result of all of this Sturm und Drang? Not a single editor has changed his or her position in any way. Not even slightly. Exactly as it has been for the last ten years. Insanity is doing the same thing over and over and expecting different results.

(Misattributed to various people, including Albert Einstein and Mark Twain. Earliest occurrence appears to have been at University of California, Irvine in social science lectures in the late 1960s.) --Guy Macon (talk) 00:39, 26 September 2012 (UTC)

Unfortunately, it does appear some people haven't changed their position at all. But you're painting with too broad of a brush.
Boris changed from "Proposal 1" to "neither". Richard seems to be changing from "Proposal 1" to "neither", and is saying things that sound remarkably like "Proposal 2". Elen currently lists herself as "neither" but is also saying things that sound remarkably like "Proposal 2". I've moved from "must include inline what various sources say about 'simple' solutions" to "must be NPOV" (years ago), and continue to offer revisions in the direction of what any reasonable persons suggests (latest, here, doesn't even mention the words "conditional probability").
It seems to me some people are trying to reach a resolution. -- Rick Block (talk) 01:31, 26 September 2012 (UTC)

Proposal: Rewrite the section on conditional probability!

Yes, I agree, it was difficult to tell apart the two proposals, which is why I voted "both". I suspect that other votes were spurious, due to the confusing wording in the proposals. To be practical, I see two issues:

  • The section in this article, on conditional probability, is written very badly, and is very difficult to understand, even if one is an expert in probability theory. This needs to be fixed.
  • It becomes clear from the arguments above that many supporters of the "conditional probability" solution have a rather weak grasp on how probability calculations are done, or even the place of conditional probability in the historical sweep of the subject. There is a failure to see how various solutions are in fact equivalent, and how different solutions can be transformed into one-another. This confuses the issues.

If the section on conditional probability is fixed so as to be clear, then perhaps much of the argumentation will fall away. So, I propose: focus on editing the conditional probability section so that ordinary readers can understand it, and then all of the other issues will fall away.

How bad is it? Its bad. Really bad. When I first read it, it seemed obviously full of errors, and I was about to start editing to fix all of them. On closer read, I saw that it was, in fact, more or less correct. But its developed in such a totally confusing, obtuse fashion, that most readers will be utterly perplexed by the "explanation" given there. And so they will react badly to it. Fix that section so that it is at least coherent. The rest will follow. linas (talk) 14:29, 21 September 2012 (UTC)

Can you clarify? Are you talking about the initial text (and figures) in Decision tree, the text (mostly formulas) in Formal solution, the text in Odds, the text in Simple solution and symmetry proof, the text in Total symmetry proof, or the text plus formulas in Bayes' theorem? These sections together have no particular flow or coherence (i.e. no one has made any attempt to consolidate them into a single section presenting them as various equivalent ways to arrive at the conditional probability). Under Proposal 2 the suggestion is to include in an initial "Solution" section only the most accessible conditional solution(s), which I think would be something like the initial text and figures in Decision tree. Would you say this section, in isolation, is "Really bad"? -- Rick Block (talk) 16:15, 21 September 2012 (UTC)
Yes I agree with Linas. For starters I think the Multiplication axiom:
should be mentioned in the description of tree diagram, as this describes what happens along each branch and makes it obvious that we are looking at conditional probabilities here.--Salix (talk): 06:59, 22 September 2012 (UTC)
One thing that might be an improvement is to use just one figure, instead of two equivalent ones (less is more). That way the reader could concentrate on just one, and the text could refer to its parts more explicitly.
The text starts calculating "conditional probability of winning by switching given which door the host opens" without explaining what that means, and how it differs from the unconditional probability. I think you end up providing the reader yet another number whose meaning they don't really understand! To understand the meaning of the unconditional and conditional probabilities here, you need to understand the difference, or you probably didn't actually understand either. I suggested a way of doing that in the "Simpler (if longer) description of the conditional solution by simulation" section, which can probably be condensed quite a bit if you don't want to actually go into simulations. Essentially, think of going to the show and filtering out the cases that match the problem description in all particulars and look at the frequency of winning among those cases, vs. doing the same without filtering. That is understandable to anyone.
The meat of the section is this paragraph:
Assuming the player picks door 1, the car is behind door 2 and the host opens door 3 with probability 1/3. The car is behind door 1 and the host opens door 3 with probability 1/6. These are the only possibilities given the player picks door 1 and the host opens door 3. Therefore, the conditional probability of winning by switching is (1/3)/(1/3 + 1/6), which is 2/3.
Taken alone, this is confusing, and no reference is made to the diagrams, so the reader is left to puzzle out that too for themselves. It would probably be clearest if we could use more concrete terminology, even if it doesn't sound all formal-like. E.g. "Consider what happens in the long run when you go on a simulated game show many times and always pick Door One yourself. If the host opens Door Three as described, there are only two possible explanations:
  1. the car is behind Door One (your door), and the host opened Door Three by sheer chance. Switching loses.
  2. the car is behind Door Two (not your door), and the host had no choice but to open Door Three. Switching wins.
Explanation (1) is clearly less likely, as it can only occur by sheer chance. The decision tree diagram (right) shows case (1) on the first bolded path and case (2) on the second bolded path, and that case (1) occurs 1/6 of the time and case (2) occurs 1/3 of the time. Case (2) occurs twice as often as case (1), because 1/3 is twice 1/6. This means that if you keep a record of the simulated show, then among the cases that exactly match the problem description (the cases (1) and (2) where the host opens Door Three), case (2) where switching wins occurs twice as often as case (1) where it loses. Therefore switching wins with probability 2/3 in the problem as described." You'd need to bold the two relevant paths in the diagram and it would be good to explain how to read the thing in the figure text, too ("The number at each branching point shows the probability that the branch is taken. One of the branches is always taken, so at each branching point the probabilities sum to one (1/3+1/3+1/3 = 1 and 1/2+1/2 = 1).", and something about the joint probabilities on the right). -- Coffee2theorems (talk) 10:04, 22 September 2012 (UTC)
The section 'formal solution' is the one that really set me off. I had skimmed the one right before, 'decision tree', because I couldn't follow it; coffee2theorems explains why. The section 'formal solution', if written correctly, would probably look like 'Bayes theorem', at the very end of the article. The title 'formal solution' is misleading; one does not need Bayes thm to solve the problem, nor is the solution somehow 'better' or 'more formal' by using it. Perhaps deleting the entire section called 'formal solution' would be best. linas (talk) 13:58, 23 September 2012 (UTC)

My proposal

Taking into account Richards comments, this is what I propose to follow the problem section. It is simple, and makes no special claims of correctness. I do not care about the order of the explanation and, do doubt, we could come up with some more imaginative titles.

Proposed text for Solution section

Answer

The contestant should always switch to the other door; contestants who switch double their chances of winning the car by switching.

Simple explanations

Explanation 1

A simple and intuitive explanation is to reason that a player whose strategy is to switch loses if and only if the player initially picks the car, which happens with probability 1/3, so switching must win with probability 2/3 (Carlton 2005).

Explanation 2

The analysis can be illustrated in terms of the equally likely events that the player has initially chosen the car, goat A, or goat B (Economist 1999):

1.
Host reveals
either goat

Player picks car
(probability 1/3) 		Switching loses.
2.
 Host must
reveal Goat B
Player picks Goat A
(probability 1/3) 		Switching wins.
3.
 Host must
reveal Goat A
Player picks Goat B
(probability 1/3) 		Switching wins.
The player has an equal chance of initially selecting the car, Goat A, or Goat B. Switching results in a win 2/3 of the time.

Explanation 3

The solution presented by vos Savant in Parade (vos Savant 1990b) shows the three possible arrangements of one car and two goats behind three doors and the result of staying or switching after initially picking door 1 in each case:

behind door 1 behind door 2 behind door 3 result if staying at door #1 result if switching to the door offered
Car Goat Goat Car Goat
Goat Car Goat Goat Car
Goat Goat Car Goat Car

A player who stays with the initial choice wins in only one out of three of these equally likely possibilities, while a player who switches wins in two out of three. The probability of winning by staying with the initial choice is therefore 1/3, while the probability of winning by switching is 2/3.

Explanation 4

Player's pick has a 1/3 chance while the other two doors have 1/3 chance each, for a combined 2/3 chance.
With the usual assumptions player's pick remains a 1/3 chance, while the other two doors a combined 2/3 chance, 2/3 for the still unopened one and 0 for the one the host opened.

Another way to understand the solution is to consider the two original unchosen doors together. Instead of one door being opened and shown to be a losing door, an equivalent action is to combine the two unchosen doors into one since the player cannot choose the opened door (Adams 1990; Devlin 2003; Williams 2004; Stibel et al., 2008).

As Cecil Adams puts it (Adams 1990), "Monty is saying in effect: you can keep your one door or you can have the other two doors." The player therefore has the choice of either sticking with the original choice of door, or choosing the sum of the contents of the two other doors, as the 2/3 chance of hiding the car has not been changed by the opening of one of these doors.

As Keith Devlin says (Devlin 2003), "By opening his door, Monty is saying to the contestant 'There are two doors you did not choose, and the probability that the prize is behind one of them is 2/3. I'll help you by using my knowledge of where the prize is to open one of those two doors to show you that it does not hide the prize. You can now take advantage of this additional information. Your choice of door A has a chance of 1 in 3 of being the winner. I have not changed that. But by eliminating door C, I have shown you that the probability that door B hides the prize is 2 in 3.'"

— Preceding unsigned comment added by Martin Hogbin (talk • contribs)

Comments on Martin's proposal

My comments on this one:

  • It starts simple, which I like. In particular, stating up front the simple fact that switching doubles the chances of winning is good, and so is Carlton's explanation.
  • I don't like the Economist explanation much, and would remove it. It's a minor and less complete variant of vos Savant's solution that doesn't match the problem description as well. vos Savant assumes that the player picks door 1 (as described) and that the car might be anywhere. The Economist assumes that the player picks any door instead of always door 1, and assumes that car is always behind door 1 (what??). Certainly it is easily completed ("cases where the car is behind other doors are similar"), but there's no reason to use it when vos Savant's version doesn't have these problems. (randomizing player's choice is necessary for optimality in certain game theoretic extensions of the problem, but that subtlety is going to be totally lost on the reader unless explicitly pointed out, whereas the car always being behind door 1 in the diagram might actually confuse someone)
  • vos Savant's solution could be made clearer by replacing the table with a diagram similar to the one in the Economist solution, except that it should of course be equivalent to vos Savant's table. It could incidentally double as an illustration for Carlton's argument.
  • "Explanation 4" section should just be deleted. There's no point in turning this into a gallery of every solution ever proposed, and these do not provide any additional insight.
  • The conditional solutions are missing, which is both a POV problem and results in lack of discussion of many of the issues involved which I'll not rehash again here.
  • Having simulations covered in this section would be good.

In short: instead of having a zillion minor variants of the same unconditional solution, have just one and explain it well, and then do the same for the conditional solution. Quantity is no substitute for quality! The text has a distinct "Oh sorry that explanation sucked so let me try again.. oh not that one either, so how about if I tweak here a little.. bear with me, I'll get around to a good one any time now!" feel to it, and that's bad. The unconditional solution would probably best be explained by a combination of Carlton's short statement and vos Savant's solution illustrated using a nice picture instead of an equivalent table. -- Coffee2theorems (talk) 11:08, 23 September 2012 (UTC)

The Economist's solution is not a minor variant of the same unconditional solution. It is a different approach, common in the economic and decision theoretic literature. It does not assume that the car is always behind Door 1. It calls the door behind which the car is located, Door 1.
Incidentally, many wikipedia editors have in the past spontaneously come up with the same approach. It represents a way of thinking about MHP (strategic/decision oriented) which is common and legitimate and different from the usual subjectivist (probabilistic) approach. Richard Gill (talk) 12:33, 23 September 2012 (UTC)
... OK. That's interesting, but most people are probably going to think of the doors numbered 1, 2, 3 from left to right on stage. The figure also has the doors ordered from left to right, and the car is always behind the leftmost door in it. It would need some kind of disclaimer that no actual spatial relationship is implied by the figure ("the leftmost door in this figure is not necessarily the leftmost door on the stage, it's instead the door we call 'door 1'"). I think that's an additional source of confusion we don't need, at least not in the first solution section. -- Coffee2theorems (talk) 21:58, 23 September 2012 (UTC)
I agree with Coffee2theorems that we don't want a zillion minor alternatives. I think we want representatives of some main classes of alternatives. I would also want to see an informal/intuitive short and snappy form of a solution based on the conditional probability approach here too. e.g.. paraphrased from Lucas et al (2009) Assume symmetry. Given: you chose door 1. The probability the car is behind door 1 and the host opens door 3 is 1/3 * 1/2 = 1/6. The probability that the car is behind door 2 and the host opens door 3 is 1/3 x 1 = 1/3. These are the only two possibilities. The second probability is twice the first. So you should switch. Richard Gill (talk) 12:38, 23 September 2012 (UTC)
I rather like this argument. It's almost as simple as Carlton's simple argument. I'd leave the rather cryptic "Assume symmetry." out and just rely on the K&W assumptions, though. (symmetry implies K&W and the other way around, so it's equivalent anyway! except that the reader can actually understand how to get the numbers "1/3" and "1/2" from the latter) -- Coffee2theorems (talk) 00:04, 24 September 2012 (UTC)
Yes, symmetry implies K&W and the other way around, so it's equivalent anyway!. This is fully correct, yes. But consider that the simple common argument of K&W ("host chooses randomly" if in 1/3 he has got "two goats" to show), what implies symmetry (Henze calls it "secrecy" regarding the car-hiding door) for years has completely been faded down, a permanent conflict. Meanwhile we know from the sources that symmetry a priori is implicit in the paradox, so that K&W and Henze more or less only are a concession to new readers who did not check that yet. But: just to avoid confusion, imo all three wordings should explicitly be mentioned: "at random", as well as "secrecy" and "symmetry". This is just my thought, and as said, just to avoid ongoing confusion and ongoing argument "can only correctly be solved by conditional probability". I would be happy if that wasn't necessary . . . Gerhardvalentin (talk) 17:26, 24 September 2012 (UTC)
My thoughts.
    • I like Carlton's initial statement and agree with Coffee2theorems that one diagram - vos Savant's table with pictures - plus Carlton's statement, is a good 'simple explanation'
    • I think you could introduce a conditional here - 'many mathematicians prefer to write out the solution taking into account which goat monty shows if you pick the car'..... with either a table or a diagram that shows clearly that it comes to the same answer.
    • Simulations, explanations in terms of game theory or strategy, and answers written in Bayesian notation should each have their own heading which describes the approach taken, avoiding falling into the trap C2t described.
    • I also think (and I seem to be alone in this) that the first section should explain why the missing assumption in vos Savant's statement of the puzzle (that Monty is forced to show a goat/that Monty will never show the car) is vital, because if Monty always picks a door at random and can show the car, there's no advantage in switching. Elen of the Roads (talk) 13:02, 23 September 2012 (UTC)
+1, my thoughts, too. - For the readers, "The article first should show the common assumptions"). Even if, for the expert, never necessary as a presupposition.
Because what one has down in black and white, it is a comfort to take home at night. Gerhardvalentin (talk) 13:35, 23 September 2012 (UTC)
I think we need a number of simple alternatives because different explanations work for different people. Explanation 4 is one that may work for people who do not like the others. If any I would drop the vos Savant table.
I am not remotely suggesting that these are the only solutions. My suggestion is that we could follow Explanation 4 with a discussion of why it matters that the host knows the position of the car as this follows naturally, from the 'Combining doors' section. From there we could lead into the possibility that the host might not choose evenly as another demonstration of the significance of conditional probability to this problem. Martin Hogbin (talk) 13:48, 23 September 2012 (UTC)
That the MHP quite often is used in textbooks teaching conditional probability theory, with various adventuresome "assumptions" for getting different values of probability to win by switching within the firm scale of 1/2 to 1 and a firm average of unchangeable 2/3 (as each "result" corresponds to the optional "adoption"), should be shown in later sections. Conditional probability theory is not "needed" to decode the paradox, but the MHP is a useful example in training conditional probability theory. And: just in order to very clearly show that a host, in exceptionally opening of that special one door that he usually is "strictly avoiding to open, if ever possible", does give the additional info that his preferred but closed door actually is most likely to hide the prize, doesn't need Bayes formula, either. Gerhardvalentin (talk) 14:45, 23 September 2012 (UTC)
vos Savant's table fits the way I would guess most schoolchildren are taught simple probability theory - you write out all the options and count 'em up, then Teech shows how you can achieve the same effect with some simple multiplication (provided you can multiply fractions). The much argued over 'conditional solution' does exactly the same thing, only allows for the fact that if your door has the car behind it, Monty has a choice of doors he can open. Using 'write out all the options' and some simple multiplication, and you really can show both solutions at the same time, because they use the same approach. This is why I think all the other approaches deserve their own sections, where you can if you like add in a bit of context for the approach. Elen of the Roads (talk) 15:11, 23 September 2012 (UTC)
I nearly completely agree with Coffee2theorems. I'm not sure it's any more clear than the table, but here's a figure version of vos Savant's table. -- Rick Block (talk) 17:12, 23 September 2012 (UTC)
Figure version of vos Savant's table
Car hidden behind door 1
(Probability 1/3) 		Car hidden behind door 2
(Probability 1/3) 		Car hidden behind door 3
(Probability 1/3)
Player picks door 1
Player has picked door 1 and the car is behind it 		Player picks door 1
Player has picked door 1 and the car is behind door 2 		Player picks door 1
Player has picked door 1 and the car is behind door 3
Host opens either door 2 or door 3 Host must open door 3 Host must open door 2
Switching to door 2 loses
Switching to door 3 loses
Switching loses 		Switching to door 2 wins
Switching wins 		Switching to door 3 wins
Switching wins
Probability 1/3 Probability 2/3

 

Rick, above I read a hint, a plausible statement to "decoding" the paradox. It says

partitioning the doors between "your door" and "Monty's doors" is the most persuasive explanation, and just suggesting the partition is often enough of a hint.

I would like to suggest, in your above clear visualization, to "enframe" all seven groups of two host's doors on the right side, as per Martin's visualization. Just to help the reader from the start to distinguish those two groups:

"group of just only one door selected" versus
"group of two unselected host's doors"

Imo such hint will help the article, and could assist the reader to "decode" the paradox, as one editor expressed above. Gerhardvalentin (talk) 19:03, 23 September 2012 (UTC)

Comment on the new figure: This may be just me and probably sounds a bit silly, but I think the Economist's format for the figure is clearer. I think it's partly because in it you have the cases stacked vertically instead of horizontally. We read from left to right and then top to bottom, and in Economist's format that tendency results in you naturally considering each case one at a time, and then looking at the whole picture once you've read all of it (also, time usually goes from left to right, not top to bottom). The arrows, the black bars separating the cases, and the numbers on the left also probably help. -- Coffee2theorems (talk) 21:34, 23 September 2012 (UTC)
Seems like this has come up before. The columnar format allows their widths to be proportional to their probability (essentially regardless of browser window size) - a fairly subtle effect but I think it helps comprehension (doing this with rows in html is much harder). Feel free to try if you want. And (BTW) I'm not sure how similar the other figure is to the actual diagram from the Economist. The online version [1] omits the figure. I believe the figure here was created independently by user:Father Goose and sourced (after the fact) to the Economist. -- Rick Block (talk) 22:05, 23 September 2012 (UTC)
How about with thick lines between the columns, per above? Another reason for this columnar orientation is it transitions very nicely to the conditional view (as shown in the next section). If we're going to have multiple figures I think it's important to pick an orientation and stick with it. -- Rick Block (talk) 15:31, 24 September 2012 (UTC)
It actually helps a bit. BTW, Krauss and Wang had plenty of suggestions about how to describe the problem. What do you think about the modified figure below?
Modified figure using K&W (&Carlton) suggestions
Case 1: Car behind door 1 Case 2: Car behind door 2 Case 3: Car behind door 3
Player picks door 1
Player has picked door 1 and the car is behind it 		Player picks door 1
Switching to door 2 wins 		Player picks door 1
Switching to door 3 wins
No matter what the host does,
switching away from the car loses 		Host must open door 3;
switching away from the goat wins 		Host must open door 2;
switching away from the goat wins
One case where switching loses Two cases where switching wins
I tried to make it similar to both vos Savant's table and K&W figure 1, and count cases instead of using probabilities as K&W say is better psychologically (note that not elaborating on Monty's behavior in case 1 is a point of theirs, presumably because splitting it into two cases might confuse when counting cases). I also tried to tie this in with Carlton's explanation by always "switching away from" whatever is behind door 1. -- Coffee2theorems (talk) 18:16, 25 September 2012 (UTC)

Towards agreement?

Although 1 and 2 are not exactly the same perhaps we could combine them in one section for brevity. I prefer the figure to the table for vos Savant's explanation. I think 4 is essential because it comes at the problem from a different angle. It also relies on the probability that door 1 hides the car remaining 1/3 after the host has opened a door. This is not the case if the host has opened an unchosen door randomly and this just happens to hide a goat to we can use this difference to show that it matters that the host knows where the car is and to lead on to discuss conditional probability. Martin Hogbin (talk) 19:45, 23 September 2012 (UTC)

The article has been opaque for years now. Please observe that the common incorrect first intuitive common appraisal of "1:1" should explicitly be addressed, immediately after section one, in showing the strange variant of a "forgetful host".
You don't need conditional probability to show that such forgetful host, by just randomly opening one of his two doors, be it the car in 1/3 or be it a goat in 2/3, inevitably will be "showing the car in 1/3", and by that is deleting the possibility to win by switching in that 1/3.
Result: probability to win by switching has been reduced to 1:1 in the remaining 2/3 ("car:goat" and "goat:car"). This should very clearly and very prominently be shown as an eye-opener. You don't need Bayes, to show that. Gerhardvalentin (talk) 20:44, 23 September 2012 (UTC)
IMO, 4 belongs in an "aids to understanding" section, not the initial explanation section. And a conditional solution needs to be included with the simple solutions. How about the following for an accessible conditional view (please note how symmetrical the figure is)? -- Rick Block (talk) 21:03, 23 September 2012 (UTC)
transition to a conditional probability view

A player who picks door 1 and switches to whichever door the host doesn't open wins the car with probability 2/3. But what about a player who picks door 1 and sees the host open door 3? [NOTE: this is here to connect to the mental model the reader almost certainly has in his/her head. We can talk about this if anyone's going to seriously be perturbed by this.]

After the player picks door 1, the host must open either door 2 or door 3. What happens in each of these two cases can be seen by rearranging one of the figures above as follows:

Car hidden behind door 3
(Probability 1/3) 		Car hidden behind door 1
(Probability 1/3) 		Car hidden behind door 2
(Probability 1/3)
Player picks door 1
Player has picked door 1 and the car is behind door 3 		Player picks door 1
Player has picked door 1 and the car is behind it 		Player picks door 1
Player has picked door 1 and the car is behind door 2
Host must open door 2 Host randomly opens door 2 Host randomly opens door 3 Host must open door 3
Switching to door 3 wins
Switching wins 		Switching to door 3 loses
Switching loses 		Switching to door 2 loses
Switching loses 		Switching to door 2 wins
Switching wins
Probability 1/3 Probability 1/6 (=1/3 x 1/2) Probability 1/6 (=1/3 x 1/2) Probability 1/3
If the player picks door 1 and the host opens door 2, switching wins with probability 1/3 and loses with probability 1/6 If the player picks door 1 and the host opens door 3, switching wins with probability 1/3 and loses with probability 1/6

Whichever door the host opens, switching wins the car with twice the probability of staying with the initial pick. For example, if the host opens door 3 either the car is behind door 2 (this occurs with probability 1/3) or the car is behind door 1 and the host randomly chose to open door 3 (this occurs with probability 1/3 x 1/2 = 1/6).

We are getting there I feel. Elen of the Roads (talk) 21:09, 23 September 2012 (UTC)

NIce. I suggest rewriting the two "punchlines" in the table as "If the player picks door 1, then the host opens door 2 and switching wins with probability 1/3 and loses with probability 1/6", and "If the player picks door 1, then the host opens door 3 and switching wins with probability 1/3 and loses with probability 1/6". The point is that the probabilities you give of 1/3 and 1/6 are probabilities given the player picks door 1, of events which include the host's choice. Richard Gill (talk) 04:13, 24 September 2012 (UTC)
Question for those more familiar with the sources: Have you seen this kind of variant of the above kind of table somewhere? (I'm sort of betting on being unable to come up with anything original about the MHP here.. :)
Door 1 Door 2 Door 3 Host's coin toss Host's door choice Result if switching Possible
Car Goat Goat Heads Door 2 Loss No
Car Goat Goat Tails Door 3 Loss Yes
Goat Car Goat Heads Door 3 Win Yes
Goat Car Goat Tails Door 3 Win Yes
Goat Goat Car Heads Door 2 Win No
Goat Goat Car Tails Door 2 Win No
You always pick door 1. The host always tosses a coin, and picks door 2 if heads and door 3 if tails, except when he is forced to pick a particular door. There are only three possibilities that match the problem description, of which two win, so the probability of winning by switching is 2/3.
The reason I'm asking this is that K&W says that counting cases is more intuitive than dealing with probabilities as such, and the former can be made possible by using a little trick like this. Otherwise it's pretty much the same thing. -- Coffee2theorems (talk) 21:03, 25 September 2012 (UTC)
This is conceptually identical to the wheel diagram used here. This particular source has been criticized earlier as perhaps not the most reliable (its an unsigned subpage of the homepage of a university course - probably created by some grad student). It's similar to the 6 mental model representation K&W describes (attributed by them to a 1999 paper I don't have by Johnson-Laird, Legrenzi, Girotto, Legrenzi, and Caverni). -- Rick Block (talk) 01:06, 26 September 2012 (UTC)
Right, such a web page is unfortunately not a stellar source. Another way to avoid probabilities would be to count the number (or proportion) of people doing something instead, and that would avoid changing the solution. Something like the following maybe?
Trying to count players instead of probabilities
Car behind door 2 Car behind door 1 Car behind door 3
Player picks door 1

Player has picked door 1 and the car is behind door 2
This happens to two in six players

Player picks door 1

Player has picked door 1 and the car is behind it
This happens to two in six players

Player picks door 1

Player has picked door 1 and the car is behind door 3
This happens to two in six players

Host must open door 3 (two in six)

Switching to door 2 wins
Switching wins

Host randomly opens door 3 (one in six)

Switching to door 2 loses
Switching loses

Host randomly opens door 2 (one in six)

Switching to door 3 loses

Host must open door 2 (two in six)
Switching to door 3 wins
Three in six players see door 3 opened.
Two of them win and one loses by switching. 		Three in six players see door 2 opened. What happens to them is irrelevant to those who see door 3 opened.
In addition to counting players instead of probabilities, I also tried removing and de-emphasizing (where removal would be more confusing) material that does not answer the question at hand (host opens door 3 case) because of the "less-is-more effect" K&W mentioned. That way, when you are trying to look where the numbers etc. came from in the next step, you're not so distracted by irrelevancies. What do you think? (there are a few modifications here, and it may also be that some are good and some bad) -- Coffee2theorems (talk) 10:16, 26 September 2012 (UTC)

Are we there yet?

Summarising the discussion so far, it seems that we get something like the below - possibly with the tables replaced by graphics or the graphics replaced by tables, and probably with changes to the wording. The sections below the 'solution' section then seem to fall naturally to me as shown - again this can be discussed further.


The Solution

Vos Savant's solution only works if - like a typical game show host - Monty Hall knows what's behind the doors only reveals the prize at the end. To do this, he must make sure never to open the door with the car behind it.

If Monty pick's his door at random, he will pick the car 1/3 of the time, and you will pick the car 1/3 of the time. There is no advantage to switching

You pick Monty picks The other door has the result if switching result if staying
Car Goat Goat Goat Car
Goat Goat Car Car Goat
Goat Car Goat Goat Goat

However, if Monty knows what's behind the doors, and is only going to reveal the prize at the end, he has to pick a door with a goat. This gives quite a different result. In this case, if you have picked a goat, Monty has to pick the other goat and leave the car hidden. You still only have a 1/3 chance of picking the car to start with, but if you switch, 2/3 of the time (the two times you picked the goat) the other door will have the car behind it.

You pick Monty picks The other door has the result if switching result if staying
Car Goat Goat Goat Car
Goat Goat Car Car Goat
Goat Goat Car Car Goat

This is true even if you use the more detailed mathematical technique known as conditional probability, which takes into account that if you pick the car, Monty has two goats to choose from.

Car hidden behind door 3
(Probability 1/3) 		Car hidden behind door 1
(Probability 1/3) 		Car hidden behind door 2
(Probability 1/3)
Player picks door 1
Player has picked door 1 and the car is behind door 3 		Player picks door 1
Player has picked door 1 and the car is behind it 		Player picks door 1
Player has picked door 1 and the car is behind door 2
Host must open door 2 Host randomly opens door 2 Host randomly opens door 3 Host must open door 3
Switching to door 3 wins
Switching wins 		Switching to door 3 loses
Switching loses 		Switching to door 2 loses
Switching loses 		Switching to door 2 wins
Switching wins
Probability 1/3 Probability 1/6 (=1/3 x 1/2) Probability 1/6 (=1/3 x 1/2) Probability 1/3
If the player picks door 1 and the host opens door 2, switching wins with probability 1/3 and loses with probability 1/6 If the player picks door 1 and the host opens door 3, switching wins with probability 1/3 and loses with probability 1/6


Other versions of the original problem

Other approaches to explaining the problem

The solution using mathematical notation

Variations on the problem

Comments

Thoughts folks --Elen of the Roads (talk) 15:47, 26 September 2012 (UTC)

I guess I'd say much the same as for Rick's proposal: "I like it, because it's broadly speaking in the right direction, but there's nevertheless much that I would change in it" :) Just different things. (both are in the "I could live with it without a complete rewrite" realm) Comments
  • Do we need to have the ignorant host case here? IMO it would fit better after the standard case has been dealt with. Immediately after (next section/subsection) would be just fine. The reason I'd put it after the solutions is that it mainly serves as a check that the reader understood the subtleties. There are people who are familiar with the MHP and yet are surprised when they hear about the ignorant host case, so it's not necessary for basic understanding of the MHP itself. It seems to me like one of those fine points one can discuss after the solution.
  • Even if we do talk about the ignorant host case, isn't starting with it a bit much? It's a different problem, after all, and modern wordings take care to exclude that interpretation.
  • I think the solutions without door numbers are hard to understand. Even if you replaced the second table with the "Economist figure" of Martin's proposal (which is much clearer), it's still confusing for the reasons I explained there. The reader is probably thinking in terms of the door numbers (1, 2, 3 from left to right on stage) anyway, and there are good solutions (vos Savant..) with them, so why not use them?
  • Emphasizing the mathematical-ness of the conditional solutions and in general making it sound difficult is not desirable. When people feel that they are expected to not understand something, it reduces the chances that they will.
  • The post-solution sections are probably best discussed after we succeed in getting consensus about the solution section :)
That's about it. -- Coffee2theorems (talk) 19:11, 26 September 2012 (UTC)
See notes below as well. Based on my limited pool of test subjects, everyone looks at (or imagines) the two remaining doors (theirs and the one Monty hasn't opened) and says "Well, it's 50/50 innit.It's either here or there." Showing that the special provision that Monty won't show the car alters the outcome is what makes the lightbulb come on. Other than that, happy to go with a different placement, and whatever others think best on the table layouts. I like the three cell display you use below. --Elen of the Roads (talk) 22:20, 26 September 2012 (UTC)

Ban all the mathematicians

OK, so I'm only partly serious, but LOOK at this talkpage. A whole bunch of mathematicians trying to convince each other that THEIR solution is the right one, that all the others are wrong, and that no-one else is clever enough to understand them.

Wikipedia:Arbitration/Requests/Case/Monty Hall problem contains the following principle

Mathematics (use of sources)

11.4) If editors disagree on how to express a problem and/or solution in mathematics, citations to reliable published sources that both are directly related to the topic of the article and directly support the material as presented must be supplied by the editor(s) who wishes to include the material. Novel derivations, applications or conclusions that cannot be supported by sources are likely to constitute original research within the definition used by the English Wikipedia.

So there should be no maths on this page that you cannot reference to a source suitable for inclusion in the article, and it should only be on this page to discuss whether the layout works, how to use Math Jax or similar. If I went through and hatted off all the non-sourced solutions, we might get a talkpage that focused on how to lay out the article in accordance with Wikipedia policies, ie by describing what the different sources say, since none of them agree with each other.

It is apparent that there is not a single agreed iteration of the problem, and there is not a single agreed solution. The sources seem to go forward in time, each disagreeing with all the previous ones, lets go thru it in iterations from vos Savant forwards in time. I know this is a physics model, not a maths one, but it gets everything in, and it MAKES NO DIFFERENCE if one of you thinks a particular 'solution' is 'wrong' because if there is a source that says it is wrong, it can't have been written until after the offending solution came out, so it is obvious that it should be placed in the article in a section subsequent to the section with the offending solution in it. Elen of the Roads (talk) 21:13, 18 September 2012 (UTC)

Hi Elen, if you look above, at how all of the mathematicians chimed in on this debate, you will notice that they all agreed with your positions, more or less. The people who are arguing against you are not mathematicians, they are average, ordinary users who are simply trying to grapple with this problem using whatever means they can. Unfortunately, they mis-use the mathematics, because, I'm presuming, they never got good at it, because, I guess, its just plain taught badly in school. Thus is the plight. Coping the attitude that you do certainly doesn't make anything any better; it just adds to the illiteracy problem. linas (talk) 03:37, 19 September 2012 (UTC)
Elen might not be right with her notion there, but your reply is definitely wrong. Most people are active here are not just ordinary users but have science backgrounds, some of them are mathematicians in particular the one Elen reacted to.--Kmhkmh (talk) 06:18, 19 September 2012 (UTC)
Who, exactly? I've been editing math articles here for years, and I have a pretty good idea who the mathematicians are on WP. I recognize a handful of names above, but the vast majority of people arguing here, including those arguing incorrectly, simply never edit any math articles. This, coupled with the fact that many of the arguments above are rather childish, when not flat-out wrong, and I must conclude they are math-illiterate. linas (talk) 13:16, 21 September 2012 (UTC)
Nijdam is a mathematician (so are Richard, Boris and myself among possibly others). If you look at edits of involved editors or their use pages (assuming they do anything else than MHP), you can quickly see that many/most seem to have a background in math/sciences/engineering. As far as "childish" (or occasionally flat out wrong) arguments/opinions/reactions go mathematicians/academics are capable of them as well and in fact have produced plenty on this subject, starting with some of the letters to vos Savant all to the way to some peer reviewed publications (an error in Morgan's paper got corrected almost 20 years later). In some way much of the ongoing discussion here is just mimicking the ongoing (academic) arguments/struggles outside WP.--Kmhkmh (talk) 06:07, 22 September 2012 (UTC)
Note that it says "If editors disagree [...] must be supplied by the editor(s) who wishes to include the material." Most of the discussions you are referring to are not about trying to include material in the article, but about trying to reach consensus. Once that is reached, the first part, i.e. "If editors disagree", becomes false, and the point moot. Most of the discussions appear friendly and honestly aimed at finding consensus, too. It's quite usual on talk pages to not source every claim, because most of that will be a part of the iceberg that will not reach the article, and the editors agree on most of those points anyway, perhaps after a bit of discussion. Editorial judgement on how to tie the sources together to form a good article is also not something that can be sourced and requires common understanding of the material.
One might argue that trying to reach consensus is a futile quest, but obviously not everyone has given up on it. It's only because the discussion has taken so long that people lose patience with the usual consensus building process. Consider, though, that the world will not end even if it takes another ten years to resolve the dispute, and we're not on a deadline here. In the large scheme of things, the MHP is just a curiosity, nothing more. A good-sized jug of coffee with a sugary lump of MHP in it ought to put things in the right perspective :) -- Coffee2theorems (talk) 05:26, 19 September 2012 (UTC)
Spot on, Coffee2theorems: "Editorial judgement on how to tie the sources together to form a good article is also not something that can be sourced and requires common understanding of the material". Especially since the number of sources is huge, they are written in hugely varying contexts (for hugely varying audiences with hugely varying backgrounds), and finally because some of them appear to disagree with one another vehemently. It is very hard to come up with genuinely new maths on MHP. A.V. Gnedin did it, his new solution was reported on in the newspapers. His research was triggered by mine, and that was triggered by the discussions here (but I only wrote up the elementary facts which everyone with a maths background agrees on, most of it well known and/or well sourceable). Richard Gill (talk) 07:28, 19 September 2012 (UTC)

Really, a good idea. I, a mathematician, ban myself for 3 years. Hey, mathematicians, follow me! Find a better hobby. We'll see what will happen here. :-) Boris Tsirelson (talk) 06:25, 19 September 2012 (UTC)

Re: "Consider, though, that the world will not end even if it takes another ten years to resolve the dispute, and we're not on a deadline here.", is there anyone here who is willing to make the argument that 20 years of discussion will reach a resolution that 10 years of discussion has utterly failed to reach? --08:33, 19 September 2012 (UTC) (Edit of Guy Macon)

Many of you seem not to have understood the two proposals here

For the reason Richard quotes above, '...the number of sources is huge, they are written in hugely varying contexts (for hugely varying audiences with hugely varying backgrounds), and finally because some of them appear to disagree with one another vehemently.' it is not possible to resolve the argument as to which solutions are correct. For that reason the proposals made by both myself and Rick accept this fact and both proposals aim to give the 'simple' and 'conditional' solution equal prominence within the article. Neither claims that either solution is better than the other.

The difference between the two proposals is only the editorial decision as to how the article is structured. As Coffe2Theorems, quoted by Richard, says, "Editorial judgement on how to tie the sources together to form a good article is also not something that can be sourced and requires common understanding of the material".

Can I as those of you who have not made a decision on the two proposals to go back and re-read them both. As you will see, it is not the case that I am proposing that the simple solutions are 'right' or 'sufficient' and Rick is saying that they are 'incorrect' or 'deficient', that is a fight that we have both withdrawn from (well I certainly have) as it can never been resolved. The question is, 'How can we as editors use our own judgement to structure the article to make it of most use and benefit to our readers without making any judgement over the 'sufficiency' of the simple sources. Simple first is what WP:technical suggests and whay most text books and encyclopedia articles do so that is my proposal. It does not in any way assert that the simple solutions are 'correct' in fact we will specifically mention the claimed deficiencies in them in the article. Martin Hogbin (talk) 09:29, 19 September 2012 (UTC)

You do not quite say but imply that "simple first" is what distinguishes the two proposals. That is not so. Rick's proposal does not say outright that the simple solution(s) should come first in the "Solution" section, but that is probably the intent (like it currently is in the article), and in any case it does not say that conditional solutions should come first! So both proposals would put simple solutions first. What you want is to leave the conditional solutions out of the main "Solution" section altogether, and Rick does not. -- Coffee2theorems (talk) 09:53, 19 September 2012 (UTC)
Imho the discussion here has developed very well in the matter. The article should be useful and "gettable" for the reader. For years it has been a tohu-bohu, concealing more than it explains. The clear "paradox" (two doors having double chance than one door) was told by a "story". For the story that had been told there are reams of variants to interpret / reinterpret /misinterpret. The article should show the clean paradox, and it should show variants where the paradox simply doesn't exist. The principal duty is to distinguish those variants and to keep them very clearly apart, and not to present a mingle-mangle as before, completely inapprehensible for the reader. The discussion here did help a lot to come closer to attain that goal. Gerhardvalentin (talk) 11:02, 19 September 2012 (UTC)
Coffee2theorems. where do I even say that there will be a main "solution" section? All I am asking is that we give the simple solutions first, with a reasonable and simple discussion of the other issues (such as the host knowing where the car is before we discuss in full' criticism of the simple solutions, and all other solutions and variants. Martin Hogbin (talk) 18:27, 19 September 2012 (UTC)
I didn't say that you said there would be one, it was my own assessment of the proposal. If there is a section called "Solution" (no qualifications), after which comes a lengthy section called "Aids to understanding", and after that comes a section that is called something else than "Solution" (no qualifications), as I understood your proposal to be, then I think it is fair to call the "Solution" section the "main" solution section.
The other proposal isn't about "discuss[ing] in full criticism of the simple solutions, and all other solutions and variants" before "a reasonable and simple discussion of the other issues" — it says that would go in a later section. Again you characterize your proposal in a way that is not characteristic of it, but characteristic of both proposals. When you are pitting off two alternative proposals, that is misleading, because people easily assume that you are talking about differences, not shared qualities. -- Coffee2theorems (talk) 10:24, 22 September 2012 (UTC)

To be absolutely clear, in Proposal 2 simple solutions would (of course) come first. The major difference in the two proposals is whether there is an initial section called "Solution" followed by one or more other sections (e.g. "Aids to understanding") mentioning only simple solutions ("with no disclaimers that they do not solve the right problem or are incomplete" - Martin has previously rejected even a forward reference to any other kind of solution) as opposed to a more inclusive initial "Solution" section with both simple and conditional solutions ("with neither presented as 'more correct' than the other"). Martin's claim that both proposals aim to give the 'simple' and 'conditional' solution equal prominence within the article is, IMO, obviously untrue. Proposal 2 does (it even says this), but Proposal 1 clearly gives much more prominence to simple solutions and furthermore creates an editorial stance that these solutions are "perfectly correct" (also implying conditional solutions "are an unimportant academic extension to the problem"). Anyone who has followed this page knows that this is precisely Martin's and Gerhard's POV (not even the POV of any particular published source), and that this POV conflicts with the POV published by numerous reliable sources that simple solutions are insufficient (not wrong per se - anyone who may be interested in this should read the discussion Martin and I has about these sources here). -- Rick Block (talk) 17:26, 19 September 2012 (UTC)

Rick the wording that you have challenged was agreed by both of us, after a very length discussion. You seem unable to distinguish between my own POV (which is that the simple solutions are what the MHP is all about) and my proposed compromise (which gives both POV's equal prominence within the article). It is pointless to say that this is untrue because it is true by definition. If my suggestion is accepted all editors could hold me to that statement by insuring that your POV gets equal prominence, provided that the simple solutions come first, unfettered. Martin Hogbin (talk) 18:23, 19 September 2012 (UTC)

All this fixation on solutions. All this fixation on right and wrong. Different sources have given different analyses, different arguments (making different assumptions, writing for different audiences). If someone can compose three or four simple English sentences which correspond to a clever derivation of a conditional probability under, say, K&W conditions, (and which moreover paraphrase a published reliable source) then this belongs right at the beginning of the article alongside other simple intuitive arguments and explanations. I begin to shift my allegiances from "Proposal 1" to "Neither" since both proposals put undue weight on the POV that there is some kind of conflict. I think that this (the opinion there is a conflict and it's important) is a minority opinion as far as reliable sources are concerned. Richard Gill (talk) 19:22, 19 September 2012 (UTC)

Richard - Proposal 2 avoids the conflict (in the article) and does precisely what you're suggesting. One reason to structure the article this way is because there are conflicting opinions (both in sources and among editors), but this conflict in no way is emphasized in the article text under Proposal 2 (the conflict "informs" the presentation, but is not mentioned). A suggestion for the three or four simple English sentences (doesn't have to be these - this is just a suggestion) are the two paragraphs and figures currently in the article starting here, up to (not including) the subsequent "Formal solution" section. This text says nothing even remotely like "simple solutions are wrong" or "conditional solutions such as this one are the only right solution". -- Rick Block (talk) 20:36, 19 September 2012 (UTC)
Two paragraphs and several figures are much more than a couple of sentences of plain English, Rick! Your specific suggestion only works for people who take the trouble to get pencil and paper and work all their way through the calculations. It'll take them half an hour. It does not provide instant insight. My favourite three sentences would be: Suppose all doors are initially equally likely to hide the car, and that Monty is equally likely to open either door to reveal a goat, if he has a choice. By symmetry, specific door numbers are now irrelevant to the problem. The player's initial choice hides the car with probability 1/3, and the other door left closed by the host therefore has probability 2/3 to hide the car. — Preceding unsigned comment added by Gill110951 (talk • contribs)
Richard - I think you're seriously underestimating the difficulty of transitioning from the mental model of having picked door 1 and seeing the host open door 3, to a more abstract mental model considering other alternatives (I've cited what K&W say about this numerous times - search for "once formed" in the archives). Your sentences make perfect sense, but they require far more from the reader than slogging through a few extremely simple calculations. I really don't understand the reluctance to address the conditional probability head-on. The text in the "Decision tree" section uses only high school level probability concepts (not even Bayes) and 6th grade math (addition and division of fractions) - and directly addresses the mental model K&W say nearly all readers form after reading the problem statement (97%, 35 out of their sample of 36). What you're effectively asking the reader to do is to put this mental model on hold, shift back to thinking about the probability the car is behind door 1 before the host opens a door (obviously 1/3), and understand that this must remain the same after the host opens a door if the host is equally likely to open either door (and must open a door). This seems to me to be a far more sophisticated (and complicated) way to look at the problem than the straightforward computation. We can certainly say this (it can definitely be sourced), but isn't the direct computation far more prevalent - and even easier to understand? -- Rick Block (talk) 15:47, 20 September 2012 (UTC)
This is a good point Rick. Yes, I *am* asking the reader to put their earlier formed mental model on hold, and try to create another. Remember, MHP is a *trick* question (it's famous, and so there's a big article on it on Wikipedia, because it's a very popular brain-teaser; not because it's a standard exercise in a conventional Probability 101 course, from the lecture on Bayes' theorem). Vos Savant's question is deliberately posed to entice the reader/listener into forming the mental picture which so dominates his/her brain that (s)he jumps to the wrong conclusion (the static picture of two doors closed, one goat and one car behind them, no point in switching). So, helping people to get deep understanding of MHP involves helping them see a different mental model which leads to the opposite conclusion. After that they may be prepared to slog through some calculations, figure out some subtelties. Different people's minds work in different ways. I saw the light with a flash of insight after realizing that a stayer/switcher wins/loses according to whether or not the car is behind the initial door. I personally didn't see the light from doing some algebra or arithmetic. Maybe some readers will appreciate your solution. Still, for me, it doesn't fall in the category "intuitive arguments which open your eyes to an alternative reality", different from the one (the false one) which Vos Savant's carefully crafted words trapped you in. Richard Gill (talk) 19:55, 20 September 2012 (UTC)
Proposal 2 is also materially different from Proposal 1 in expressly calling for a section on "criticism of the 'simple' solutions". I strongly oppose this because it is a tendentiously non-neutral way to characterize sources that are talking about different interpretations and definitions of what the question is. Execrably so when a source says solution S1 does answer problem P1 but not problem P2.

These differences are essential context for the solutions but, unfortunately, attempts to contextualize solutions in the article have been met with strenuous objections about "health warnings" or "disclaimers" about the relevance of the solutions. This is why I cannot support Proposal 1 either: it simply does not settle the dispute.

Using the colorful example in Rosenthal (2005a) where Monty opens door #3 unintentionally because he slips on a banana peel, one side appears to be insisting that simple solutions must be criticized because they don't work when Monty slips on a banana peel, and the other side appears to be insisting we must not mention that simple solutions do work if Monty doesn't slip on a banana peel. This is nutty as a fruitcake.

I fully endorse Richard Gill's remark that "both proposals put undue weight on the POV that there is some kind of conflict." The conflict is between the contributors, not between the substance of the sources (notwithstanding the uncollegial tone adopted by at least one of the sources). ~ Ningauble (talk) 18:55, 20 September 2012 (UTC)

I agree with Ningauble and Richard Gill on this one. For those who haven't been following this for years, a bit of history might be of interest. When I saw this dispute after the arbcom decision, I figured that Wikipedia's standard dispute resolution process should be given a fair chance. This has been through a number of the steps (third party mediation, ect.) but at the time I determined that the specific dispute had not been the subject of an RfC. It took months to get that to happen. The problem? Rick and Martin would not accept any description of the dispute written by the other. No matter what either of them wrote, the response from the other disputant was was "that's not what this dispute is about! This is what the dispute is about!" Finally, after taking several long wikibreaks from trying, I was finally able to get them to agree on RfC wording.
Each of them was pretty confident that the consensus would support their proposal, and both of them made a commitment to stop disputing if the consensus was against them. The RfC is still young, and things may change, but it is pretty clear that there is not going to be a clear consensus for either proposal. It hasn't happened yet, but a clear consensus for "neither" could emerge. If it does, both Martin and Rick should graciously accept that consensus and stop fighting over two positions that have both been rejected by the community.
I am really hoping that comments like "Many of you seem not to have understood the two proposals here" are not precursors for a future position of "because many here did not understand the two proposals I will now ignore the lack of consensus for my position and continue battling" or "because my opponent mischaracterized my proposal I will now ignore the lack of consensus for my position and continue battling" --Guy Macon (talk) 19:58, 20 September 2012 (UTC)
Guy, as I have already said to you, I accept that there is no consensus for my compromise proposal or for Rick's proposal and if that is the final result of this RfC I am quite happy not to propose it again. I will stick to my original POV from now on.
It is extremely uncharitable for you to attempt to characterise this dispute as a fight between myself and Rick. We both tried to find compromise solutions to an ongoing battle between two groups of editors and we were the only two who actually produced concrete proposals that could be comment upon. You mentioned the history of this dispute. Here is the history of the dispute. As you will see many other editors have argued over which are the best solutions for this article and over a considerable period of time. I (and Rick) tried to find a way to resolve this dispute but accept that we have failed. The problem now is that the original dispute continues with no sign of any resolution or consensus. Martin Hogbin (talk) 20:58, 20 September 2012 (UTC)
@Ningauble - if you're objecting to calling the section "criticism" how about if we call this section "Detailed discussion of solutions" (or some such). The intent of Proposal 2 is that this is essentially the same as the section Proposal 1 characterizes as "a full and scholarly exposition of the 'conditional' solutions" and is where the specific differences between "simple" and "conditional" solutions would be described (of course in neutral terms). -- Rick Block (talk) 20:14, 21 September 2012 (UTC)
It is unfortunate that the proposals (and sometimes the proposers) aren't entirely clear on what is the same and what is different about the two. Would it be too late to add some clarifications on that to the RfC? -- Coffee2theorems (talk) 11:09, 22 September 2012 (UTC)
@Rick: – A rose by any other name would be as thorny. Rather than a discussion of the merits of various solutions, or some such, I would like to see a discussion of various ways of interpreting the problem. I think the "Criticism of the simple solutions" section should be gone; and the "History" section (which should lose the mere mentions that do not contribute to or illustrate the historical development of the problem, or at least move them to "In popular culture") should be re-cast to show various perspectives on such matters as, e.g., the initial ambiguity about whether Monty must open an unchosen door revealing a goat, the issue of the objective(-ist) significance Monty's choice of goats, and the issue of the subjective(-ist) insignificance of, say, door numbers.

What I am earnestly opposed to is placing undue weight on disputation about solutions, thereby obscuring the very thing that complementary views might help to illuminate, to wit, understanding the Monty Hall problem. ~ Ningauble (talk) 17:57, 28 September 2012 (UTC)

OK, I give up

I have made it as clear as it is possible to do that my proposal does not claim that any solution is right or wrong yet even Richard is now saying that I have a fixation with right and wrong. It seems that words no longer carry any meaning and that this is percieved purely as a battle between the 'simplists' and the 'conditionalists' who both claim to have absolute right on their side. It is rather like a debate on law and order. No matter what anyone says there are only two views that a person can be perceived to take, 'hard liner' or 'liberal'. Rational discussion is no longer possible. Whatever happened to WP:assume good faith? Martin Hogbin (talk) 08:32, 20 September 2012 (UTC)

Martin, I do not accuse you of having such a fixation, nor lack of good faith. My point is that the discussion degenerates into a debate on whether some "solutions" are "right" and some are "wrong". I think that informal arguments, intuitive arguments, arguments which aid insight and intuition, should go first in the article. We should restrict ourselves to arguments which are actually *correct*, even though this is a concept alien to Wikipedia policies. But I do hope everyone here agrees that it is correct to say that if your initial choice has 1/3 chance of hitting the car, then the chance the car is behind one of the other two doors is 2/3. This argument is found all over the literature, not difficult to source it. Notice: I do not say that this is a "solution" to MHP. I say: this is what many notable sources have written about MHP, and many readers of Wikipedia will find it illuminating, and it is correct. Some readers will see it as a solution, some won't, who cares.
I would like to ban the word "solution" from the first sections of the article. If there is a simple and correct argument, which can be expressed in one or two sentences only, and which most readers will understand, and which actually (for the insiders) corresponds to a derivation of a conditional probability, then it can be there in the beginning of the article (I think there are several such). Again you see I am veering to Boris Tsirelson's opinion that the article should start simple, without being incorrect. It's my opinion that this would allow some variants of "the full solution" (including an informal version of the notion of conditioning) to also be present in the early part of the article.
I'd like to see mathematicians and non-mathematicians to be able to work together on the article, and both mathematicians and non-mathematicians to find it a fabulous resource. Richard Gill (talk) 12:02, 20 September 2012 (UTC)
I really do not understand you. You say, 'All this fixation on right and wrong.', then you insist that, 'the article should start simple, without being incorrect'. It would seem that my attempt to find a compromise has failed so the fight is likely to go on for a few more years. Martin Hogbin (talk) 20:32, 20 September 2012 (UTC)
Dr Gill, I'm with you. Martin, I think he's saying 'correct' only as in 'the arithmetic/logic is correct', 'Sooty's magic wand is not invoked' or (I would add) 'correct allowing that the puzzle specification was incomplete' (for vos Savant). Not 'correct' as in whatever Nijdam's 'correct' explanation is that he will never tell us, or Rick Block's correct explanation that probably is correct but requires a four paragraph exposition of the problem and three screens of maths to set out the answer. Elen of the Roads (talk) 21:25, 20 September 2012 (UTC)
Elen, I think you will find that Richard has a rather stricter idea of 'correct' than you think, but let us not speculate. Richard, would you say the following solutions are correct, exactly as they are (these are solutions copied from various versions of this article)?
I'll comment per example later this weekend. But you use the word "solutions". We can't discuss whether a solution is right or wrong till we have fixed the problem it is supposed to address, and the level of detail which is acceptable for the context. I prefer to study the presented argument. Is each step in the argument logically correct? If assumptions are needed to justify argument steps, are they stated explicitly? If assumptions are made explicitly, are they used in some step of the argument? Is the stated conclusion of the argument the same as the actually justified conclusion? A completely separate question is whether or not a given, correct, argument can be considered to be a solution of the original problem.
One can evaluate the correctness of a written argument without use of external information, objectively. One simply inspects the internal logical coherence of the argument. One clearly cannot evaluate the correctness of a solution to Vos Savant's question objectively, since it's a matter of taste how the informal verbal question should be converted into an unambiguously mathematically formalizable question. Richard Gill (talk) 08:04, 22 September 2012 (UTC)
The argument of the Economist is a correct argument for the statement that the player who chooses a door at random and then switches, whichever door is opened by the host, will win the car with probability 2/3, while the player who chooses his door at random and then stays, whichever door is opened, wins with probability 1/3. Note: no K&W assumptions! Randomness is in the choice of the player! I like this.
The argument is also given by several academic mathematics sources, in particular by your truly. It's the important part of a game-theoretic (decision theoretic) argument. The specialist can go on to remark that no strategy whatsoever can do better than 2/3 (there are of course a whole lot of more complicated strategies than the two particular ones "choose at random, always switch" and "choose at random, always stay").Richard Gill (talk) 16:04, 22 September 2012 (UTC)
The argument called combining doors seems to take a subjectivist notion of probability. The only assumption which is used, is that the car is equally likely behind any of the three doors. (The competitor's choice is fixed). It ignores the possibility that you might want to do something different depending on whether the host opened door 2 or door 3. It's a good argument for lazy competitors who are not interested in which door is chosen. If they switch they'll get the car with probability 2/3. Competitors who are not so lazy might wonder about whether or not it could be useful to do something different in case the host opens door 2 and in case the host opens door 3. However, if indeed they are using probability in the subjectivist sense, they'll correctly consider the specific door number irrelevant. Their prior information is symmetric in the door numbers so the specific door numbers opened in a specific case are irrelevant. Possibly this is in the back of the mind of all those who only compare "always switch" with "always stay". The door numbers are arbitrary labels, nothings is changed in the problem by renumbering then. Hence we need only consider strategies which are the same however the doors are numbered. Laziness is completely justified. It seems to be a matter of taste whether one explicitly mentions that one has reduced the problem by symmetry, or not, and many writers probably do it subconsciously. Richard Gill (talk) 16:25, 22 September 2012 (UTC)
The argument from Parade uses the same assumption as the combining doors argument: the car is initially equally likely behind any of the three doors; the competitor's choice is fixed. It shows completely correctly that by switching (whatever door is opened) you'll get the car with probability 2/3. The possibility that it might be to your advantage to switch in one case but to stay in the other (depending on which door is opened) is not raised. Of course, once you've seen that always staying and always switching have success probabilities of 1/3 and 2/3 respectively, it would seem quite inconceivable that a mixed strategy could go above 2/3; one would very naturally suppose that it would do something in between. The mathematician might like to add a little mathematical analysis which shows that this is indeed the case. For instance, Gnedin's dominance argument shows very easily that it is a waste of time to consider staying in any situation whatsoever. Richard Gill (talk) 16:34, 22 September 2012 (UTC)
In summary, the arguments presented in all three sources are correct, it seems to me. By this I mean that the author's derivation of the author's stated conclusion under the author's stated assumptions is correct. The assumptions are different and the arguments are different and the conclusions are different! What do we learn from this? The controversy here has arisen from earlier wikipedia editors who created the false impression that there's only one MH problem, namely that "Marylin is asking for a conditional probability", and hence that solutions can be classified right or wrong according to whether or not they present a logically correct and explicit derivation of a conditional probability. No. Stupid. Each different source converts Marilyn's words into a different problem and solves it in an appropriate way. (Morgan et al even deliberately misquoted Marilyn in order to twist the problem towards their solution. And imposed a frequentist interpretation on probability, thus making the problem more difficult). One of the three arguments here is decision theoretic (and makes the competitor also active in his choice of door choice strategy). The other two use subjective probability and symmetry, partly explicitly partly implicitly. All the argumentments are simple, intuitive, insight-increasing... and correct. Richard Gill (talk) 05:35, 23 September 2012 (UTC)
Just to clarify (since I've inadvertantly upset him) - I'm not criticizing Rick because his solution is long, and I apologise if he thought I was. It takes as much space as it needs (and I was exaggerating a tad). My concern is that I don't think it's particularly intelligible for the reader. In terms of the described outcome, it's plainly not any more 'right' than vos Savant's original solution(they both say you'll win twice as many times by switching), so in that sense it's just a more complicated way of getting to the same answer.Elen of the Roads (talk) 22:25, 21 September 2012 (UTC)
Apology accepted. However, the point is not whether one solution is more 'right' than another, and how intelligible the conditional solution night be is mostly irrelevant. Per WP:MTAA "It is important not to oversimplify material in the effort to make it more accessible", but we should of course make it as intelligible as is humanly possible. The point is how often and in what kind of sources these solutions appear. The fact is that many, many sources publish conditional solutions. In addition, sources keep being published distinguishing "simple" and conditional solutions, and explicitly expressing a preference for conditional solutions (Morgan et al. in 1991, Gillman in 1992, Eisenhauer in 2001, Rosenthal in 2005, Grinstead and Snell in 2006, Lucas et al in 2009). Per NPOV the article must represent "fairly, proportionately, and as far as possible without bias, all significant views that have been published by reliable sources". Omitting a conditional solution from the initial "Solution" section (as Proposal 1 would) IMO creates a strong bias. -- Rick Block (talk) 03:25, 22 September 2012 (UTC)
And other sources keep criticizing the sources you've named for deliberately misrepresenting Vos Savant, narrow-mindedness regarding interpretation of the the problem, dogmatism, and apparent unawareness of alternative ways to express a simple argument in more formal mathematical terms. If door numbers are irrelevant by symmetry then insisting on emphasizing that the 2/3 chance of winning by switching is also the conditional chance of wining by switching given ... is obfuscation. But, if there are short sharp insight-increasing simple solutions built around conditional probability (there are: the Bayes theorem odds form argument) then they belong at the front of the article. (Simple Bayes: Assume symmetry. Initially the odds are 1:1 that the car is behind your chosen door, door 1, or behind door 2. The host is twice as likely to open door 3 in the second case than in the first case: in the second cases he's certain to do so, in the first case there's only a 50% chance he'll do so. So after he's opened door 3 the odds on door 1 versus door 2 have changed to 1:2, by Bayes' rule. The reader who doesn't know what odds are and what Bayes' rule is, is hopefully enticed to read on and learn more. There are reliable sources aplenty who give this argument.) Richard Gill (talk) 06:00, 23 September 2012 (UTC)
The Lucas et al. paper is excellent. It does *not* say that unconditional solutions are wrong. It *is* all about conditioning and about showing how Bayes helps you solve all kinds of complicated variants of MHP. It does have a short verbal argument in the intro. Assume symmetry. Given: you chose door 1. The probability the car is behind door 1 and the host opens door 3 is 1/3 * 1/2 = 1/6. The probability that the car is behind door 2 and the host opens door 3 is 1/3 x 1 = 1/3. These are the only two possibilities. The second probability is twice the first. So you should switch. This is a correct argument and it's simple. It belongs among the "simple solutions". Richard Gill (talk) 06:41, 23 September 2012 (UTC)
Which "other sources keep criticizing the sources [I've] named for ..."? Please be specific. Even if you can cite such sources my point remains valid. In your opinion (and presumably the opinion of any sources you might be able to cite), the sources I'm citing are full of crap. However, this simply further demonstrates the fact that this is a POV issue. Wikipedia simply cannot take sides, regardless of what anyone (even Richard Gill) thinks (unless we're talking fringe opinions, which we're not). "Many sources say 'simple' solutions are inadequate" is a true statement. This is not the same as saying "simple solutions are inadequate" - which seems to be a matter of opinion. Do you agree? -- Rick Block (talk) 07:01, 23 September 2012 (UTC)
A number of the published discussion contributions to Morgan et al. criticised them soundly. Also, if I remember correctly, Jason Rosenhouse criticises them too. So does R.D. Gill. And finally, Morgan et al. retracted their original harsh criticism of Vos Savant. I do not say that the sources you are citing are full of crap. They contain much that is of value. What they say should be taken in context. I agree that it's a matter of opinion as to whether or not simple solutions are inadequate. And depends very much on which simple solution you are talking about. They are not all the same. A guy called Bell in the discussion of Morgan's original paper already wrote that it was a matter of taste whether or not one was explicit about symmetry assumptions. And of course Wikipedia can't take sides. The question is how much weight should be paid to this "matter of taste". In my opinion, not a great deal. I would prefer to treat Wikipedia readers as intelligent persons who can do any judging for themselves. The level of precision and expliciteness which one might require in a probability class is not the same as the level of precision and expliciteness which one requires in a popular discussion of a popular brain-teaser. Moreover we saw again when comparing the arguments given by the Economist and by Parade, that writers from economics and decision theory have a very different way of looking at MHP. No K&P assumptions at all, probability is entirely in the hands of the contestant! I'd say that it's up to those who are fond of the conditional solution to present it as attractively and accessibly as possible to the readers. Preferably at two levels; first short and informal/intuitive, among the other short informal/intuitive arguments; secondly at greater length and with the level of mathematical precision one would find in an introductory probability text. If this had been done long ago we wouldn't be arguing about these things today. Richard Gill (talk) 12:24, 23 September 2012 (UTC)

Back to where we started

We are now pretty much back where we started with, as Gerhard says, an opaque article which confuses the reader with conditional probability before addressing the crucial issue of why it matters that the host knows where the car is. If you look through the talk pages you will see that that issue comes up time after time. On the other hand the door opened by the host is never mentioned.

I am not saying that Rick's table should not be in the article just that we need to address the most common questions that newcomers wish answered. Conditional probability is of much more interest to the more expert reader and in my opinion it should be addressed in a more scholarly manner. Martin Hogbin (talk) 15:02, 24 September 2012 (UTC)

I think it is important to address conditional probability in an unscholarly manner! Make it accessible to all. Rick's table shows that the probability of winning by switching is twice the probability of winning by staying, both when the host opens door 2 and when the host opens door 3. There is no mention of conditional probability. If this is considered too much detail early on in the article, why not just reproduce the Lucas, Rosenhouse, and Schepler (2009) verbal argument: Given: you chose door 1. The probability the car is behind door 1 and the host opens door 3 is 1/3 * 1/2 = 1/6; the "times half" is here because the host has two alternative choices! The probability that the car is behind door 2 and the host opens door 3 is 1/3 x 1 = 1/3; the "times one" is here because the host has no choice in this case. These are the only two possibilities. The second probability is twice the first. So you should switch. Note that Rosenhouse is the guy who wrote a whole book on MHP, also appeared in 2009. Richard Gill (talk) 16:40, 24 September 2012 (UTC)
Richard - you are sounding more and more like a Proposal 2 kind of guy. Can I ask you to review your response to the RfC? AFAICT, if you think it is important to address conditional probability (in any manner, scholarly or not) you are not in favor of Proposal 1. -- Rick Block (talk) 18:35, 24 September 2012 (UTC)
I am veering more and more to a "neither" guy as far as proposal 1 versus proposal 2 is concerned. I would like to see the initial parts of the article present various *different* arguments, all of them accessible to a broad audience, and all of them correct: these should represent the broad range of ways that different people have approached MHP. It would be obvious that the different arguments make *different* assumptions. If reliable sources have presented simple arguments which from a mathematician's point of view are actually formalizations of a conditional probability argument, then I fail to see why they should be excluded from the early part of the article. Richard Gill (talk) 11:59, 25 September 2012 (UTC)
This is an article on the MHP not a text book on probability. 95% of those who read this article will not have any interest in conditional probability at all but they will want to know the probability of winning by switching and why it matters what the host knows. Martin Hogbin (talk) 19:45, 24 September 2012 (UTC)
Martin, all this is doing is saying "...mathematicians prefer to write out a longer version of the solution, that takes into account that if the contestant picks the door with the car behind it, Monty has two goats to choose from. Whether you use the simple version or this more detailed explanation, the result is still the same..." It's not mentioning conditional probability (as an academic discipline) at the moment. Elen of the Roads (talk) 20:16, 24 September 2012 (UTC)
I agree with you Martin on the needs of 95% of the readers. Do you think that the Lucas et al. argument is too difficult for 95% of our readers? Richard Gill (talk) 12:24, 25 September 2012 (UTC)
Yes, it is far too complicated to start with. The argument needs to be convincing and therefore very simple or there is no point in going on. If the reader does not believe that the answer is 2/3 they are not going to read through an argument that is more complicated but which has no more explanatory or convincing power than the simple ones do. Once the reader has been convinced, and also understood why the host's knowledge matters, then the Lucas explanation is fine, along with many others. The Morgan argument is then quite straightforward to understand and believe. As Morgan did in their paper, the argument for taking account of the host's choice of door is most easily introduced by considering a variant of the problem in which the host does not choose evenly. That the conventional formulation can be considered as a special case of this is trivial for anyone who gets this far. To introduce that piece of pointless pedantry at too early a stage can only put people off. We should never underestimate the power of the basic problem to confuse. Martin Hogbin (talk) 12:55, 25 September 2012 (UTC)
Elen, I do not accept that, '...mathematicians prefer to write out a longer version of the solution'. Some may do in some circumstances, but why do we need to make that at the start. It is of far less interest to nearly all readers than is the startling difficulty of the main problem. The problem here is that the article is being written by editors in order to prove some point or other rather than being written for the benefit of our readers. Martin Hogbin (talk) 12:55, 25 September 2012 (UTC)
Real mathematicians prefer to write a short verbal solution which uses mathematical insight and which at the same time is complete and easily (for the professional) formalizable. Unreal mathematicians prefer to write out a long explicit formal calculation. For instance, if you want a subjectivist probability solution, then this particular mathematician would write the following: if we have no a priori knowledge about how the game show organizers hide the car or how the host chooses a door to open when he has a choice, then the problem is symmetric in the door numbers, and the specific door numbers in a particular instance are irrelevant. The only question are the relationships between the door chosen by the player, the door hiding the car, the door opened by the host, and the door left closed by the host. By symmetry there is probability 1/3 that the competitors initial choice is the same as the door hiding the car. In that case, the other closed door hides a goat, otherwise, the other closed door hides a car. Therefore the probability that the other closed door hides a car is 2/3, independently of the specific door numbers involved in any particular instance.
On the other hand, if you want a strategic/decision oriented solution, like the writer of the Economist, then this particular mathematician would write the following: if you choose your door initially completely at random and then switch you'll get the car 2/3 of the time. It's an easy exercise to show that this is the minimax solution, i.e., no other strategy is guaranteed to win at least 2/3 of the time.
Please note: the real mathematician has different solutions to offer, depending on the taste of his client, the consumer so to speak. They make different assumptions, they are both short and sweet and a professional easily fills in any details. As a class-room exercise in a course on elementary probability or on elementary game theory (or mathematical economics) one might write some things out in more detail. Richard Gill (talk) 13:52, 26 September 2012 (UTC)
Richard I like your subjectivist probability solution and I think we need to say this somewhere in the article, but not at the start. At the start we need some very clear diagrams, as I have proposed. Martin Hogbin (talk) 18:04, 27 September 2012 (UTC)
Who is the audience for this? Martin Hogbin (talk) 08:38, 25 September 2012 (UTC)
The article should show that, for the common scenario, conditional probability is not *needed*. In the common scenario, it is futile to use it, but of course you can use it.
The conflict here for years has been: Is the claim that conditional probability being "the only correct approach to solve the paradox" relevant or irrelevant in the common scenario. Gerhardvalentin (talk) 09:37, 25 September 2012 (UTC)
The notion of "probability of winning by switching" is ambiguous. There are three common meanings of it here: (1) what proportion of all switchers win?, (2) what proportion of switchers who choose door 1 win?, and (3) what proportion of switchers who choose door 1 and see host open door 3 to show a goat win?. Given what most non-mathematicians would consider to be mild assumptions (i.e. K&W), these are all 2/3. Many people think (3) is the quantity they want to know after reading the problem description. The simple solutions show that the answer to (1) or (2) is 2/3 and leave the step of going from there to (3) as "obvious", but it isn't obvious to everyone, so connecting the dots for them is nice.
As to why it matters that the host knows (and is constrained to pick a goat), the simple conditional solution answers it easily: if the host knows, the alternatives are 1/3×1/2 and 1/3×1 (explained already), whereas if the host doesn't know, they are 1/3×1/2 and 1/3×1/2 (the host always has two 50:50 options). -- Coffee2theorems (talk) 21:20, 24 September 2012 (UTC)

Or in my seriously clunky table version

Door 1 Monty opens door 2 Door 3 result if switching result if staying
Car Goat Goat Goat Car
Goat Car Goat Goat Goat
Goat Goat Car Car Goat
Door 1 Door 2 Monty opens door 3 result if switching result if staying
Car Goat Goat Goat Car
Goat Goat Car Goat Goat
Goat Goat Car Car Goat

If Monty doesn't know where the car is, there is no advantage to switching.Elen of the Roads (talk) 22:45, 24 September 2012 (UTC)


If Monty doesn't know where the car is, then the common intuitive answer will be the case (1:1, no difference).
  • In one out of three the host got two goats, he will show one goat and switching loses the already first selected car.
  • In one out of three the host got the car and a goat, and (in that one out of three) inevitably, by chance, will show the goat, and switching wins.
  • But: in one out of three the host got a goat and the car, and (in that one out of three) inevitably will show the car, and *game-over*.
Door A Door B Monty opens door C result if switching result if staying
Car Goat Goat Goat Car
Goat Car Goat Car Goat
Goat Goat Car game-over game-over
If Monty doesn't know where the car is, there is no advantage to switching, the common intuitive estimation (1:1) applies, irrespective of the location of the doors and irrespective of any door numbers. Regardless whether the doors have numbers or the door first selected by the guest has been "called" #1, regardless whether the car is behind door 1, 2 or 3. Regardless whether the guest first selected door 1, 2 or 3. And regardless whether the host has opened door 1, 2 or 3. It is useless to distinguish and to condition on the location of the doors or to condition on door numbers.
It applies for the case that the guest has selected door 1 and the host has opened any other door, so it also applies for the case that the guest has selected door 1 and the host has opened door 3. It applies in any case, so it applies in every case and it applies for "always" switching / staying also. Distinguishing "before" the host has opened a door and "thereafter" is pointless. In the given scenario, that the host knows the location of the car, probability to win by switching 2/3 applies for any permutation. And if the host does "not" know the location of the car, probability to win by switching 1/2 "also applies for any permutation". To distinguish "before" the host has opened a door and "thereafter", in the given scenario, shapes up as an error in reasoning. Gerhardvalentin (talk) 01:15, 25 September 2012 (UTC)
You're right. I couldn't figure out if that presentation was adequate, but it is very effective. You can eliminate the door designations - I tried this above, but some folks found it even more confusing. What do you think --Elen of the Roads (talk) 15:44, 25 September 2012 (UTC)


You pick a The other door has a Monty Picks a result if switching result if staying
Car Goat Goat Goat Car
Goat Car Goat Car Goat
Goat Goat Car game-over game-over

Yes, this is exactly was Richard once said: Not "door numbers" are of relevance, but relevant it is the role / the function of a door: Door first selected / door opened by the host / and door offered as an alternative to switch on.

And as to me, the question was about a "one time problem", as Tom did underline. "This show", in exactly this way, never occurred in reality, and the famous question is a tricky brain teaser. Now IMO you can regard the problem with the view of a subjectivist / Bayesian, or with a frequentist's view, both is okay. But IMO Morgan et al. did overshoot the mark by telling that in observing "available log lists" (perormance-lists) regarding the host's behaviour in opening one of his two doors, you can detect whether he "had" resp. still "has" some special preference to open just only one of his two doors. (Not to forget: The famous question is about a "one time problem" that never happened in exactly this way in reality, where "secrecy" regarding the car-hiding door is implied therefore: One-Time-Problem). MCDD said that the host could have been telling additional info on the actual location of the car, additional info revealed by "which-one" of his two door he actually did open: "No secrecy" regarding the actual car-hiding door.
In exceptionally opening of his "strictly avoided door", he could divulge e.g. the additional information that the car actually is very likely to be behind his second still closed door, that he usually prefers to open, if any possible, but actually had to left it closed. Imo no more "frequentist's view", but a complete new story of some quite OTHER game show that since long has been ongoing. Nothing to do with the famous "one time problem". But the article insolently pretends MDCC did address the "original" scenario as per MvS.

All of that "conditioning on door numbers" is interesting and helpful in class rooms and textbooks teaching probability theory, but without impact on the famous question "Is it to your advantage to switch". So that quite "other scenario" should be shown, yes, but should be shown later, saying that all of this addresses quite another "problem", that this addresses not MvS, but addresses repeated shows. Yes, "conditioning" on door numbers may be shown, but never mingle-mangled with first of all helping the reader in decoding the famous paradox. Gerhardvalentin (talk) 17:28, 25 September 2012 (UTC)

Yes indeed. All of that guff needs to be relegated to a section headed "Variations" or "Exploring the possibilities" or some such. It's a one shot puzzle - what isthe answer to this one occurrence. Since it's the same answer in simple or conditional versions, let's put in both. No suggestion that the simple one is wrong - it's not - just that the conditional one is a bit more detailed. Morgan and his fantasies about Monty's motivations belong right down at the bottom of the article....or maybe in another article Variations on the Monty Hall problem --Elen of the Roads (talk) 15:19, 26 September 2012 (UTC)

Who are we writing this for?

There is a lot of discussion above that seems aimed at convincing other editors here of some subtle point or other.

WP is intended to be an encyclopedia, not a debating forum. Disputes on the benefits various solutions may be OK in the talk pages but they should not spill over into the article. There we should be only addressing the needs of our readers, not trying to prove some mathematical point.

How do we know that problems our readers will have? In the case of the MHP this is easy, we have two sources of information, the talk pages here and reports of the many letters that vos Savant received. In both cases the problems were: believing that the answer is 2/3; and understanding why it matters that the host knows where the car is. There is no record of a single person saying to vS, 'but what if Monty had opened door 2 to reveal a goat?'; for nearly everyone this is a non-issue. That is not to say that we should never mention this possibility but it can only ever be of interest to those that have completely understood the first two points. Martin Hogbin (talk) 09:49, 25 September 2012 (UTC)

The point of the article is not to convince our readers of anything, but to be (per WP:NOT) "a summary of accepted knowledge regarding its subject". It is accepted knowledge that in the standard version of the MHP 2/3 is both the overall probability of winning the car by switching (per many published "simple" solutions) and the probability of winning the car by switching from door 1 to door 2 if the host opens door 3 (per many published "conditional" solutions).
Vos Savant's explanations all said in one way or another that 2/3 is the overall probability of winning by switching (with or without having picked door 1, i.e. what Coffee2theorems describes above as the proportion of all switchers or the proportion of switchers who choose door 1). This is the characteristic that effectively defines your notion of "simple". We know for a fact that Vos Savant found this kind of explanation not convincing and resorted to challenging her readers to do a simulation.
Yet you keep insisting that "simple" explanations are, if not easy to understand, at least obviously easier to understand than a solution explaining the probabilities in the specific case where the player picks door 1 and the host opens door 3. What is this insistence based on? Are there any sources that say this? K&W say the problem statement creates a mental model that prevents "access" to a "simple" solution. Have you considered the possibility that your mental model of the problem is not that same as the mental model of most of our readers? It seems to me you want to structure the article based on your mental model, with an extensive selection of solutions none of which fit the mental model K&W say nearly all people (97%!!) have after reading the problem statement, all similar to solutions vos Savant's readers (for whatever reason) couldn't accept. Don't you think it might be better to include a solution that addresses the mental model 97% of our readers will have? -- Rick Block (talk) 18:06, 25 September 2012 (UTC)
If readers are not persuaded that the article gives the correct answer they will disregard any information that we present to them. Do you think this serves our encyclopedic purpose.
My understanding of K&W is diametrically opposed to yours. Nowhere does it say that readers consider it important which (of the legal possibilities) door the host opens. If you want to discuss this I suggest we do it on my or your talk pages.
Can you present any evidence that clearly and unambiguously says that a more complex solution is more convincing? Martin Hogbin (talk) 18:32, 25 September 2012 (UTC)
It just is not true that an article about conditional probability theory can help the reader to easily decode the famous simple paradox that has been presented in the form of a story about the sequence of a one-time problem: Devolution brought forth "two still closed doors", one hiding the prize, the other door hiding a nullity. But in the given scenario, and by the given sequence, one door has double chance to hide the prize than the other door. It could easily be decoded by the reader if the article addresses this very aim. But for years now the article never says clearly what it is just talking about. Concealing more than it explains. Presenting a textbook in conditional probability theory, showing that a duad / a fixed combined group of two host's doors never can nor will have a better chance compared to one single door (see the story of the host's two "purses" above, it just depicts the conflict in the article).

Help the reader first to decode the paradox, and stop claiming that conditional probability is the only way to grasp that the group of the host's two doors has a better chance to hide the prize than one single door first selected.

Look at the archive of this talk page, confused readers have been left helpless and alone, thanks to the position of few incorrigible editors. Stop the article to be a textbook on unimportant negligibility. Gerhardvalentin (talk) 19:50, 25 September 2012 (UTC)

FWIW, I took K&W to mean the same as Rick did, I think. Assuming that we expose the reader to the standard version (with door numbers) before the solution section, it would make sense to backtrack at the beginning of the solution section and tell the reader to ignore the "door 3" part and to consider the proportion of winners among all switchers who pick door 1 instead. It is easily established that this is 2/3. Then, we can consider the proportion of winners among all switchers who pick door 1 and see door 3 opened in a simple way (what Gill calls the Lucas version here, suitably illustrated so that it is easy to understand). At that point, we will have addressed the question the reader was thinking of, whichever it was. K&W do suggest that it is the latter one:
"Although, semantically, door 3 in the standard version is merely named as an example ('Monty Hall opens another door, say number 3"), most participants take the opening of door 3 for granted and base their reasoning on this fact. [...] Note that once formed, this assumption prevents the problem solver from gaining access to the intuitive solution illustrated in Figure 1 [essentially the vos Savant solution].",
and
"The additional specification of the door opened by Monty Hall in the standard version of the problem leaves only two of the three arrangements [...] (A1 and A2). [Ax is the situation where you picked door 1 and the car is behind door x]. A3 is impossible because Monty Hall cannot open the door concealing the car. As a result, one cannot simply count and compare [...], but rather one has to reason in probability terms to reach the Bayesian solution. That is, Monty Hall's opening door 3 has a lower probability in A1 than A2, because in A1 he could have opened either door 2 or door 3, whereas in A2 he had to open door 3."
The reasoning in the last part here is essentially the "Lucas solution", and they are essentially suggesting that you have to use it for the standard version because of the mental model it induces.
The reason I suggested backtracking is this part from their article:
"The easiest way to make sure that participants' reasoning processes are not impeded by knowing which door Monty Hall opens, is simply not to give them this information. The corresponding formulation would be: 'Monty Hall now opens another door and reveals a goat."
If we have to use the standard version from the beginning (and I suppose we do, due weight and all that), then the next best thing to doing as K&W suggest is to tell the reader to put the mental model they have on hold for a moment, right? But just for a moment; the conditional solution should follow immediately after. Telling people to put a thought on hold like that for a long time tends not sit in well with many people (and it needs to be worded properly, not literally "ignore this part for now" but "one interpretation of the question is..." or something, so it's more palatable). -- Coffee2theorems (talk) 19:52, 25 September 2012 (UTC)
Yes yes. One of my monumentally clunky tables was an attempt to explain just as 'your door' 'Monty's door' 'the other door' without door numbers. I think it's possible to go "here's the problem....the significant thing to remember is that Monty can't show the car. If Monty just picked a door at random, there would be no benefit to switching, but because he can't show the car.....(cue simple tables)" Then (as I rather keep saying - sorry) - mathematically you can write this out in a more detailed way (one of the conditional tables above). Now I have realised that the reason I couldn't originally understand the conditional version was because of the way it was written out (sorry Rick or whoever constructed it), I am convinced it can be written out clearly enough for anyone with a decent maths GCSE to follow. I think that sequence, followed by the other iterations of the problem, the other approaches to a solution (formal or thought experiment) is far clearer that presenting the editor with half a dozen apparently different 'solutions' immediately. Elen of the Roads (talk) 22:04, 25 September 2012 (UTC)

A necessary and sufficient condition

As we seem to be getting nowhere again, I am going to suggest a completely different approach:

Suppose we give the vS/Whitaker problem statement with the following addition. 'It is generally assumed that the host takes no action and no other event occurs that changes the probability that the player has originally chosen the car'.

This innocuous statement covers all the angles, the host must offer the swap, he must always reveal a goat, and he must choose a legal door uniformly. It avoids the need for conditional probability since the posterior probability that the player has chosen the car is equal to the prior probability by definition.

We can then relax this condition to include the host opening an unchosen door randomly that happens to reveal a goat, the host having a preference for a specific door or goat, the host indicating by some other means where the car is, and the host only offering the swap when the player has chosen the car. The MHP and 10 years of argument in a nutshell. Martin Hogbin (talk) 00:06, 29 September 2012 (UTC)

I'm really not sure if you're kidding or not. Assuming you're not, how would you reference this suggested statement? You're basically saying it is generally assumed the probability the car is behind the player's originally chosen door remains 1/3 (which it obviously is before the host opens a door) after the host opens a door. It seems to me this is the entire point of the problem, and if you simply assume this you're assuming the answer. Rather than argue about this, let's just say [[citation needed]. -- Rick Block (talk) 05:20, 29 September 2012 (UTC)
I am sure that we could find a reference for this somewhere. We are writing an encyclopedia not a puzzle book, so if the problem statement starts to lead the reader in the right direction that is an advantage. Is my statement even contentious? Is there anyone who thinks that the problem is not generally (and intentionally) set up in such a way that host's action does changes the probability that the player has originally chosen the car? Martin Hogbin (talk) 09:09, 29 September 2012 (UTC)
I did just give you a reference. RD Gill (2011). Let's approach MHP with the layman's notion of probability, which is the same as the notion of the founders of probability, Huygens, Pascal, Laplace, Boole... Probability is in your mind, it's about your knowledge or lack thereof, and if you have no reason to think otherwise, then alternative possibilities are equally likely. This is probability based on symmetry, which unfortunately is apparently a too difficult word for editors and readers of wikipedia. However probability in the classical sense is all about symmetry. Probability in our readers minds is all about symmetry. Symmetry is why everyone initially thinks that the chance the car is behind Door 1 and behind Door 2 must be the same. But at this stage of the problem, Door 1 and Door 2 are doors with two different roles: the door initially chosen by the competitor and the different door left closed by the host. Because these doors have different histories they no longer have the same probabilities to hide the car.
Let's imagine being told the MHP story bit by bit and let's think about how our opinion changes as our knowledge grows. Initially the chance for us is 1/3 that the car is behind the competitor's door, Door 1 (symmetry). Then we are told that the host opens a different door revealing a goat, something which he always can do because he knows where the car is, and something he always does do. Our information about whether there's a goat or a car behind Door 1 hasn't changed. So for us the chance is still 1/3 that the car is behind Door 1. Finally we are told that the host in fact opened Door 3. We know he was going to open Door 2 or Door 3, and the number on the door which he opens is irrelevant to whether or not there is a car behind Door 1, since we have no information to the contrary (symmetry).
Please delete the word symmetry everywhere from these remarks if it is too expensive for you. The solution I have just told you is published by R D Gill and it's a combining doors solution: it talks about the chance that the car is behind Door 1 versus the chance that the car is behind Door2 or Door 3. Richard Gill (talk) 09:26, 29 September 2012 (UTC)
So we now have a reference. My suggestion is to make this point part of the problem statement, not part of the the solution. The problem was intentionally set up this way right from the start by Selvin. If doing things this way makes the solution easier, or even obvious, that we have achieved a great success. Martin Hogbin (talk) 09:32, 29 September 2012 (UTC)
No Martin. MHP is solved by different authorities in fundamentally different ways. The choice of which route to take is free. I think there are three basic ways: (1) Some authorities solve MHP by strategic thinking (the location of the car is fixed but unknown, probability is in the choice of initial door by the competitor), "choose at random and switch" is the minimax solution. (2) It is solved by some authorities, e.g. Morgan et al., by frequentist probability, probability is used to model the actual long run behaviour of the quiz-show team and of the host. Assumptions as to what these long run probabilities are have therefore to be made explicitly. These "input" probabilities have to be part of the complete problem statement. (3) It is solved by other authorities using subjective probability and therefore symmetry. Invoking symmetry to get the subjective probabilities of this and that (thus: not how the team hides the car, and how the host makes his choice, but the facts that you, the problem solver, have no information about either process) is part of the subjectivist's solution. The probabilities are part of the solution, not part of the problem. Richard Gill (talk) 11:23, 29 September 2012 (UTC)
Yes, the probabilities are part of the solution. That's why I think we should avoid potentially confusing "changing probabilities" in the initial Solution section, because we can. It can readily enough be read in a Bayesian way without shoving that interpretation down the reader's throat.
Incidentally, you are wrong about subjective probability implying symmetry. It is only the objective Bayesian (the people who like reference priors and suchlike) who is forced to use a 50:50 prior; a subjective Bayesian may choose whatever prior they please. Perhaps they think that the doors are numbered from left to right and the host is probably right-handed and that right-handed people probably pick the right-hand door more often so the host probably favors door 3 vs door 2; in any case, if a subjectivist tells you they believe that and you disagree, they are not obliged to agree with you just because you claim your subjective prior is "objective" or "the right one" or whatever. If you want to have only one unambiguous subjective Bayesian solution, you must specify the prior; there's no way around that. -- Coffee2theorems (talk) 11:55, 29 September 2012 (UTC)
I meant the objective subjective probability of Laplace, Boole and so on. And I reiterate that I think that this is the way most laypersons think about probability. — Preceding unsigned comment added by Gill110951 (talk • contribs) 12:07, 29 September 2012
Exactly. Nobody is making a subjective guesses about the a priori right-handedness of Monty or how this might influence his choice of goats. The symmetry lies in applying the principle of indifference, i.e., the uninformative prior that uses symmetry when there is no knowledge indicating unequal probabilities.

There may be some terminological confusion about "objectivist Bayesian" and "subjectivist Bayesian", and even some debate about whether all Bayesianism is subjective at root; but in the course of discussions here, "subjective" has been used to contrast "Epistemic probability" with "frequentist statistics". In these pages, for the variant in which Monty's method of choosing goats is unspecified, the so-called "subjectivists" treat the absence of information about Monty's preference as an epistemological fact and calculate a "probability" expressing the state of ("subjective") knowledge; and the so-called "frequentists" treat Monty's preference as an ontological unknown and formulate a "probability" in terms of an open parameter to express a state of ("objective") reality. They really do not mean the same thing by the word "probability".~ Ningauble (talk) 15:48, 4 October 2012 (UTC)

The statement "It is generally assumed that the host takes no action and no other event occurs that changes the probability that the player has originally chosen the car" is not true; that is not generally assumed, but is often considered to be something to be shown. It is also very confusing to some people who are not familiar with belief update or probabilities changing or the Bayesian way of phrasing things. Consider e.g. these comments from the talk page of the Sleeping Beauty problem where a conditional/unconditional interpretation issue also arises:
"What is your credence now for the proposition that our coin landed heads?" This could mean "What probability should she give to the coin actually landing heads?" and is definitely a question about her knowledge of the ratio of throws H:T that actually occur (or land). It appears to (erroneously) imply that this probability could vary which might explain why it is often interpreted as the quite different question "What probability should she give to her correctly guessing that the coin had landed heads?"
and:
The HALFERS define the Bernoulli trial as the the coin flip. The waking of Sleeping Beauty is a secondary event. When awakened, Sleeping Beauty is being asked to reflect on an event that occurred in the past. The halfers imagine the interviewer asking "Hey we flipped a coin on Sunday, what do you think the chances are that it came up heads?" Beauty will say 1 out of every 2 trials regardless of the number of times she was awakened.
The THIRDERS define the Bernoulli trial as the waking of Sleeping Beauty. Sleeping Beauty is being asked about the present. The thirders imagine the interviewer asking "See that bowl there? What do you think the chances are that the coin under it reads heads?" Beauty will answer 1 out of every 3 trials. It could be argued that these trials are not truly independent.
and further:
The Sleeping Beauty problem is not a mathematical paradox. The two probabilities are the result of calculating probability in different ways, the unconditional probability being 1/2 and the conditional probability (given the condition that Sleeping Beauty be awake) being 1/3. Neither of these is "the right way"; both are valid approaches.
If someone tossed a coin and asked me what my credence was for the proposition that the coin landed heads, I would respond 50% for obvious reasons. To me, "what is my credence" is just one of many ways of asking what the probability is. So I can see no reason why Sleeping Beauty should answer differently. The business with Mondays and Tuesdays and putting to sleep and amnesia-inducing drugs is just window dressing added by the problem's poser to mislead the solver into giving a false answer. It's not unusual to do that, and in fact considering how people normally try to solve this it's clear why the problem was posed that way.
Try thinking of it like this. If the problem asked "What is your credence now for the proposition that the coin landed heads, given that you are awake?" the answer would clearly be 1/3. So why hasn't the poser asked it that way? My answer is, because that's not what he is asking for. He has deliberately added the trappings of a conditional probability problem in order to trick the reader into giving the wrong answer. You've fallen for his trick.
How utterly confused is that? You are begging for the same kind of confusion here. People who think probability is immutable think that "the probability that the player has originally chosen the car" means the unconditional probability, no matter what happens afterwards. Suppose you are told which door the car is behind. To these people, the probability you speak of is still 1/3, because, you know, that's what it originally was and that's what it still is, (unconditional) probabilities don't ever change, this is mathematics, once something takes on some value it will forever be that value.. etc. -- Coffee2theorems (talk) 10:38, 29 September 2012 (UTC)
Who says that all the probabilities are part of the solution and not part of the question? It was clearly Selvin's and vS/Whitakers intention that the action of the host should not change the probability that the car is behind door 1. If we state this as part of the problem then all solutions become much clearer. Martin Hogbin (talk) 13:25, 29 September 2012 (UTC)
Perhaps you interpret Selvin/vS/Whitaker that way (though I don't really see how). Perhaps they even intended it that way. That doesn't really matter much. The assumption is not generally made; there are many who do not make it, and in many sources it is not something to be assumed but something to be proved.
Aside from that, even if what you say were the case, it would be much clearer to state the assumption this way:
It is generally assumed that when door 3 is opened, the probability that the car is behind door 1 remains unchanged.
The argument against the 50:50 misconception then becomes very simple indeed: the probability that the car is behind door 1 was 1/3, and by assumption it still is so; therefore it is not 1/2. -- Coffee2theorems (talk) 14:04, 29 September 2012 (UTC)
I agree with Coffee2theorems. Everybody who writes down some probability assumptions to solve the problem is solving the problem by introducing probability assumptions. Solving such a problem entails motivating some formal assumptions and then proceeding to use those assumptions (and just those assumptions) in a logically correct way to get to a formal conclusion, and then finally translating the formal conclusion back to a real world statement. In the academic context we perform each of these three steps explicitly. In a popular context ordinary people don't draw the boundaries between the three phases distinctly and may skip discussion of steps which everyone finds obvious. But if challenged, one must be prepared to make distinctions and one must be prepared to fill in "obvious" steps. Not everyone makes the same assumptions. That's why the article can't start by saying that these are the assumptions we have to make. It can start by saying that certain assumptions are very commonly made.
In my opinion, most laypersons approach MHP using (symmetry driven) subjective probability. Thus without comment they automatically assume that, for them, the car is equally likely behind any of the three doors and that if the host has a choice of door to open it is equally likely, for them, that he opens either. This does not mean that the car is hidden by using an unbiased random generator and that the host chooses a door to open by using an unbiased random generator. It means that we don't know anything about how these tasks are performed, and hence that what we do know about it, is unaffected by renumbering the doors anyway we like. This implies that subjective probabilities of events involving numbered doors won't change if we renumber those doors. Therefore the subjective probability of winning by switching given any particular door initially chosen and any particular door opened by the host does not depend on what those particular door numbers happened to be. Therefore these subjective probabilities are all equal to the overall subjective probability of winning by switching, which anyone can quickly be convinced equals 2/3. OMG, what an ado about nothing.
But many laypersons approach MHP with quite a different mindset. See The Economist for instance. Strategic thinking! The location of the car is fixed but unknown. The only randomness which we know about is in the choice of initial door by the competitor. Richard Gill (talk) 14:11, 29 September 2012 (UTC)
As the famous story about a one-time problem has asked the question "is it to your favor to switch", the "behavior" of the host may not (since cannot) be misinterpreted. Doing it though, means forgetting about this one-time problem and creating another new story with quite another new different question. But the paradox might not "appear" in other quite different stories and questions. So N.Henze, in addressing the paradox, explicitly says that the host is "observing secrecy" regarding the door that hides the car. I see that to be a clear and logical consequence for that one-time problem. So: No maths in the problem description. Gerhardvalentin (talk) 14:26, 29 September 2012 (UTC)
As to the MHP sources say that, under explicit suppositions, the conditional probability to win by staying can / may vary within the fixed range of 0 to max. 1/2, under all possible suppositions, with an average of 1/3. And they say that the conditional probability to win by switching can / may vary within the fixed range of at least 1/2 to 1, under all possible suppositions, with an average of 2/3. And sources say that simple solutions correctly give an overall probability to win by staying of 1/3, and correctly give an overall probability to win by switching of 2/3.

And sources say that the overall probability to win by switching of 2/3 "impossibly can be enhanced" by any "staying" on any single occasion. Sources say that, as a consequence, it never will be necessary to base the conditional probability to win by switching on "which" door has been opened by the host who even is "invited" to be biased. The door opened by the host may never be considered as a basis to your decision to stay or to switch, this would be a fallacy, as you know already from the very beginning that staying forever is definitively excluded. Excluded just from the outset. So the simple solution is fully correct in neglecting "which" door has been opened by the host. Regarding the MHP conditional probability, based on the door opened by the host, is once and forever unnecessary, as per the sources. Switching gives an overall success rate of 2/3 that cannot be augmented by any "staying".

So imo it is superfluous to try to reconsider the probability of the door first selected to hide the car, based on which one of his two doors has been opened by the host. Imo the article should base on reliable sources. Gerhardvalentin (talk) 00:42, 30 September 2012 (UTC)

I think that I should clarify what I meant, 'that the host takes no action and no other event occurs that changes the probability that the player has originally chosen the car' is a necessary and sufficient condition for the simple solutions to be valid. If the condition is not fulfilled then the simple solutions can be criticised for requiring unstated assumptions. If the condition is satisfied the simple solutions are all perfectly correct. That is all I am saying.

Coffee2theorems, I do not see how Selvin's problem statement can be interpreted any other way that implying the above condition. Are you suggesting that Selvin envisaged that some action of the host would change the probability that the player had chosen the car? When pressed on that point he made it clear that he was not considering that possibility.

Vos Savant also made clear that she interpreted the question (that she actually formulated) in the same way. In both formulations the original intent is clearly that the host takes no action that changes the probability that the player has originally chosen the car. Martin Hogbin (talk) 22:49, 29 September 2012 (UTC)

Your statement
the host takes no action and no other event occurs that changes the probability that the player has originally chosen the car
is already basically implied by the K&W assumptions, as is shown by the conditional solutions. The simple fact that the problem statement implies some thing X doesn't mean that we should incorporate X as a part of the problem statement. The problem statement also implies that the player should switch, and Selvin probably didn't envisage that staying would ever be better either. That doesn't mean that we should add "It is generally assumed that the player should switch." as a part of the problem statement. That would be a total cop-out. Likewise, anything that allows us to basically say
the probability that the car is behind door 1 was 1/3, and by assumption it still is so; therefore it is not 1/2
is a total cop-out. We proved that it's not 50:50 by assumption! I don't think anyone would accept that. That assumption is too strong in some vague cop-out sense (technically e.g. the assumption "the player should switch" is weaker than "the host is unbiased", but the former is stronger in this vague cop-out sense). You also can't escape this by obfuscating the assumption somehow; by doing that you merely replace the original MHP paradox by a new one that arises entirely from your choice of obfuscation method. The widely accepted obfuscation method (accepted to do no violence to the problem) is to say that the host chooses uniformly among his choices, and we shouldn't invent our own.
I'm also not convinced that your assumption is necessary or sufficient for validity of the simple solutions. You could argue that it is not sufficient because it is not explicitly referred to by the solutions. You could argue that it is not necessary because it is obvious (given unbiased host / symmetric beliefs) that the optimal strategy must either be "always switch" or "always stay", and unconditional probability suffices to show that the former is better, and hence optimal.
Be the result of such an argument as it may, the unconditional solutions are valuable because they intuitively resolve a part of the paradox: The fact that switching is optimal in a particular situation is not paradoxical or even the least bit surprising after you understand that always switching is twice better than always staying. Conditional solutions provide the rest of the resolution, showing why the 50:50 "argument" (if you can call it that) is wrong, as well as dotting the i's and crossing the t's for those who care. -- Coffee2theorems (talk) 02:15, 30 September 2012 (UTC)
The condition is necessary for the simple solutions to be valid in that if it is not met, in other words if the host does do something which does change the probability that the player has chosen the car then the simple solutions are wrong, not just in that they might be considered incomplete but in that they give the wrong answer. The condition is the only one which guarantees an answer of 2/3. The arguments you give only apply if other conditions are met, for example that the host always offers the swap and the host always reveals a goat. My condition is actually at the core of the problem, without it the problem is actually insoluble. The conditions in K&W are in fact rather poor substitutes for my condition and do not, as I have shown here actually guarantee the right answer in all cases.
I do understand that spotting the condition might be regarded as being a key part to solving the problem rather than an explicit part of the problem statement and if we were setting a puzzle for readers I would not add that condition because it might weaken the power of the MHP to confuse. However, we are writing an encyclopedia and therefore weakening the problem might actually be to our advantage. We want people to understand exactly what is going on and the key fact in understanding that is the assumption (I think obviously intended by both problem authors) that the host action does not change the posterior probability that the player has chosen the car. Martin Hogbin (talk) 09:19, 30 September 2012 (UTC)
Martin: first of all you are restricting yourself to the K&W conditions or what one could call: solution by subjective probability, symmetric case (probability as expression of ignorance). Fine to concentrate on this case, but please remember it isn't the only way people approach MHP, whether academic of amateur. Secondly, IMHO, you are assuming what needs to be proved. Thirdly, your approach is completely novel. Or is it your idea that many amateur writers take this approach without making it explicit? Still, that's a novel interpretation of what went on in the mind of Vos Savant and other popular writers. Richard Gill (talk) 10:04, 30 September 2012 (UTC)
I am restricting myself to every case, in which the initial probability that the player has chosen the car is 1/3 and to which the answer is 2/3. The answer is 2/3 iff the probability that the host has chosen the car remains 1/3, regardless of how the problem is set up, what model of probability is used, and what approach is used to calculate the answer. This is why I want include the condition in the problem statement not in any particular solution.
I am not assuming quite what needs to be proved, which is that the probability that the player will win by switching is 2/3 but I do accept that I have moved some way in that direction. I am stating the one and only essential assumption for the answer to have the standard/conventional value of 2/3. It is the other assumptions that are not always necessary and generally not sufficient to ensure the correct answer. I agree that we are leading the reader by the nose by stating this essential assumption as part of the standard/conventional formulation of the problem but is that a bad thing here? We are supposed to be trying to help the reader not fool them. It would be very interesting to present people with the vS/W problem statement plus my condition and see how many get the right answer. My guess would be more than usual but far from everyone (even though the correct answer follows from a simple and obvious calculation).
I think vS went some way towards stating my condition as a central assumption, as did Selvin. Whether what they said is clear enough to be used as a source I cannot say without reading what they wrote carefully again. Martin Hogbin (talk) 13:27, 30 September 2012 (UTC)
So you are asking for the conditional probability (this is what you mean by "the answer"), or in less expensive words, you are asking that the probability does not depend on specific door numbers. You assume that the car is initially equally likely behind any of the three doors (this rules out the Economist's solution). What you call an assumption is what other people call a deduction. You are correct in noticing the mathematical fact that the logic works both ways. Your way: answer is 2/3 implies the host's choice is completely random. Other way: host's choice is completely random implies answer is 2/3. I think your observation is technically speaking OR, I did not see it in the literature.
Selvin (1975a) assumes car location and initial choice are independent and completely random. He looks at 9 equally likely combinations. In essence he gives the combining doors solution. Given the location of the car and the initial choice, he compares "switching" with "staying". He doesn't differentiate which door is chosen by the host when the host has a choice since it doesn't make a difference to whether switching or staying wins or loses, in his table of 9 combinations. He ignores the possibility that one might like to make one's choice of switch or stay depend on which particular doors are involved in a particular case.
Selvin (1975b) was written in response to many letters he got about his first article. One of the letters was from Monty Hall himself, who gave a short sharp version of the combining doors argument (the chance that the car is behind door 1 is not changed by subsequent events).
Selvin (1975b) explicitly adds the assumption that the choice of the host is completely random and he states explicitly that his earlier solution is based on this assumption, even though was no mention of the assumption and no explicit use of the assumption in Selvin (1975a). He goes on to give a new solution by calculating the conditional instead of the unconditional probability of switching. The suggestion that he wants to give is that the two solutions are the same. A pedant could have avoided the explicit calculation but instead added the phrase, "the chance of winning by switching doesn't depend on which particular doors are chosen and opened (symmetry!) since the host is equally likely to open either door if he has the opportunity" to his first solution. (As discussants of Morgan et al. also did, later).
Vos Savant is mainly concerned with preserving her image of being an immensely smart person who always gets it right. She does not waste ink getting into technical niceties. I don't think she ever explicitly said that she was silently assuming the host's choice as being completely at random, and I don't think she ever explicitly argued that this assumption was needed in order to solve the problem. (Discussants of Morgan et al. considered this a matter of taste). I do think that she implicitly did want us to assume complete symmetry, by using the words "say, Door 1" and "say, Door 3". This leads to a formal solution where one invokes symmetry at the outset to forget door numbers altogether. By symmetry, the only thing that interests us are the chances that the initially chosen door hides the car and the other closed door hides the car. These chances have to be the same as the conditional chances of the same events, given the specific door numbers, e.g. 1 and 2. But there is no particular interest in saying so. It can be added, for completeness, and if you do so it will please people like Nijdam. A matter of taste. Richard Gill (talk) 09:48, 1 October 2012 (UTC)
As you know, I would rather say nothing at all about conditions. I have no objection to using the term 'conditional probability' but I see no reason why the door number opened by the host should be regarded as the only significant condition of the problem.
It's a common way the word probability is used, to always understand "conditional on everything that you know at this point in time". When people ask "wnat is the probability", they mean "what is the probability, given what you know". As some history of events unrolls in time, "the probability" of anything in particular is always the probability relative to some observer, and hence it evolves in time as the observer gets more information. For Monty, the probability the car is behind door 2 is fixed at 1, or 0, at the start of the show. For the competitor it changes as his actions stimulate reactions from Monty, which give him more information. From decision theory, theory of dynamic programming, we know that if you want to maximize your overall succes-rate at some task, then at any point in time at which you have to make a choice, you shoukd make the choice which gives you the best conditional chance of success given what you know so far. Pretty intuitive, right? This is the reason why the conditionalists keep saying "you have to compute the conditional probability to solve the problem". They don't know the reason why, for them it is a dogma. But understanding why the dogma is actually sound wisdom, we see that we can do without it. If we can succeed with unconditional probability 2/3 by always switching, and if there is no way to do better, then there is no point at all in figuring out conditional probabilities. I think this is the reason why ordinary folk are completely satisfied with simple solutions. It's obvious you can't do better than 2/3, so no more needs to be said.Richard Gill (talk) 20:21, 1 October 2012 (UTC)

I think this "dogma" is generally taken as axiomatic to the Bayesian approach because it is just too dang obvious. People do not generally ask "Why should it be optimal to take into account everything I know when making a decision, and why should failing to do so generally not be optimal?". People also do not prove obviosities like this or the intermediate value theorem because they suspect they are false, but to check that there are no problems in the formalization and to get the precise range of applicability. Was there ever any doubt?!

I agree with you that the "strategy with best guarantees" result is very nice. That is perhaps the only kind of result that has any serious claim to objectivity (in a "the solution of tic-tac-toe" sense). But the 2/3 number in it is not the probability of winning by switching at any time after the player's initial choice (this would be a likely misconception). Those probabilities, as well as initial probabilities of car location, are completely unknown with so weak assumptions, but insisting that they are unknown is unlikely to go over well.

That solution is assuming that when nothing definite is known, you should not bother with beliefs, but take the guaranteed result. While it gives a good result here, that kind of thinking in general has its own problems, as it focuses solely on worst opponent performance, ignoring average opponent performance (I've heard that e.g. at poker that approach sucks; you do much better by exploiting people's tendencies, even if you don't have enough data on them to satisfy a frequentist). As a general approach, reliance on beliefs in the face of uncertainty has its strong points (understatement?), and it is only natural that complete rejection of the approach ("car location probabilities are completely unknown!") will appear ludicrous, even if it has a strong justification in this idealized case.

All in all, I think these are nicely complementary approaches. Also, if it's assumed that everyone except perhaps the particular player under consideration plays optimally under certain fairly natural adversarial conditions (read: "obviously the host/producer will want to maintain total secrecy!"), then even a frequentist would agree with the Bayesian (we get K&W that way). If you ask someone: "What is the probability that you win at tic-tac-toe?", they'll probably respond: "Against a good opponent? Zero." Same with the MHP: "Against a good opponent? 2/3." -- Coffee2theorems (talk) 12:15, 2 October 2012 (UTC)

My condition is the one single condition which totally defines the MHP as the simple puzzle that it was intended to be. The only other thing required is that the probability that the player has originally chosen the car is 1/3. I do not,as you suggest above, require the car and goats to be uniformly placed. You say in your edit summary that, 'answer is 2/3 if and only if host's choice is random', but that is not true. The answer is not 2/3 if the host does not know where the car is or if the host only uses the word 'pick' if the player has originally chosen the car, or if the host only offers the swap if the player has originally chosen the car. All these things, and many others, are ruled out by my condition. It is the essential core of the problem statement, not part of the solution.
Maybe my proposed condition is OR but I was rather hoping that you would see the benefits of using it and find a sources that might justify its inclusion. Martin Hogbin (talk) 15:20, 1 October 2012 (UTC)
When I said "answer is 2/3 iff host's choice is random" I meant it to be understood, that all the other standard ingredients are present: the host must open a different door revealing a goat, and the car is initially equally likely to be anywhere. Since we are talking about MHP it is understood that the host must open a different door revealing a goat. And please distinguish the technical meaning of the word "conditional" in the expression "conditional probability", from the meaning of the word "condition" as in assumptions, initial conditions, and so on. No relation at all! Completely different things. ... But regarding your new discovery: no I am not aware of any sources which had this insight, before you. You may well be right. But still I see it as something to be deduced, not something to be assumed. You are close to saying: the answer is 2/3 if we assume the answer is 2/3. Impeccable logic, but not so interesting. Richard Gill (talk) 18:36, 1 October 2012 (UTC)
Yes I do understand that some of the points I mentioned are part of the standard setup of the MHP but I still think that it is worthy of note that my condition ensures all that at a stroke. However, some of the points are not normally even mentioned. The possibility that the host gives the player a helping hand by changing his wording, for example, when the player has originally chosen the car. In a real game show that is a more likely scenario than the host's having a door preference.
By 'condition' I am referring to the bit in italics in say, P(C=1|Host reveals Nanny). Is that the correct terminology?
If you want to compute a conditional probability, anything you want to condition on is called a condition, yes. If you think a problem should be solved by computing a probability then you also have to make up your mind whether it should be conditional on anything, and if so what. Part of your "solution" should be to motivate why you want to condition, in the technical probabilistic sense, on anything. I see MHP as a decision problem: should you switch, yes or no? Computing probabilities or conditional probabilities are a means to solving the problem, not the solution itself. So if you solve MHP by calculating some conditional probability you have to explain why this particular conditional probability. If you solve it by calculating some unconditional probabilty you have to explain why that was sufficient for your present purposes.
Now, if you have to choose between doors 1 and 2 and you want to achieve the highest possible (unconditional / overall) chance of picking the door with the car, then you should condition on everything which you know and which can influence the chance that the car is behind door 1 or 2. Then you'll be guaranteed of the highest possible overall chance of getting the car. In MHP it's not difficult to see (given what we're told about the problem) that there's no way to get a better overall success chance than 2/3. We also know that "always switching" gives you overall success rate 2/3. So 2/3 is attainable. So no need to compute conditional probabilities: you know you are doing the best that can be done anyway.
Alternatively you might like to argue, by symmetry, a priori, that the chance of winning by switching does not depend on the specific door numbers chosen and open. In that case there is no point in conditioning on them because you know this information doesn't alter the chances anyway.
But OK, if you don't like either of the preceding two solutions, you can say that you'll compute the conditional probability the car is behind door 2 given everything which the player has seen so far, since if he bases his choice on this conditional probability, he's guaranteed of doing the best overall.
I would say that imagining situations where information is revealed by seeing whether the goat behind door 3 is a boy goat or a girl goat is silly. When you tackle a problem like MHP you start by deciding what you're going to take account of, what not. Selvin and Vos Savant tell us only a few things and it's understood that we're only supposed to reason with the information we've been told, not to start building fantasy variant-problems where there is more information around or different information. Richard Gill (talk) 14:05, 2 October 2012 (UTC)
Perhaps then you can explain to me why conditioning on the goat that is revealed is sillier than conditioning on the door that is opened. Martin Hogbin (talk) 22:35, 2 October 2012 (UTC)
Because we're told about the door that is opened and we can't help but discuss door numbers in some way or another in setting up a mathematical model of the problem. We're not told anything about the goats and we're obviously not supposed to start imagining that there's a boy goat and a girl goat and this should make a difference. However we are told about the door numbers. Under K&W assumptions, they are irrelevant: their irrelevance can be deduced from those assumptions. So if you explicitly write out the complete K&W assumptions but just give a simple solution and don't explain why the specific numbers in a particular case are irrelevant, there's something strange going on: you're making an assumption but not showing where you used it in your argument. It's not a big deal: one word would be enough; but unfortunately there seems a lot of opposition here to use of the word "symmetry". This is sad. It's a fundamental insight into the problem (under K&W assumptions) that permuting the door numbers changes nothing, hence there is no need to evaluate strategies which depend on door numbers: the solution must be the same for all door numberings. I am not a supporter of blanket use of K&W, by the way. I think that the simple solutions are legitimate precisely because they give the consumer useful information while making less assumptions. Hence the simple solutions have wider applicability. Richard Gill (talk) 11:27, 4 October 2012 (UTC)
Richard, I have replied to your original response in my user space to avoid logging up the discussion here. Martin Hogbin (talk) 11:40, 5 October 2012 (UTC)
You are suggesting that the answer is obvious if we include my condition in the problem statement but here is the thing - which part of the MHP is not obvious? Yet most people get it wrong. Despite K&W we still do not really know why people cannot answer what should be a very straightforward question. Why they still do not believe you when you carefully explain the correct answer. Including my condition in the problem statement would be an interesting way of helping readers to reach the right conclusion. We can then go back and explain the standard requirements for my condition to be true. Martin Hogbin (talk) 21:19, 1 October 2012 (UTC)
Without a whole slew of references saying something fairly similar to what you're suggesting (enough to convincingly show that this is how the problem is generally interpreted) including your condition in the problem statement is prohibited WP:OR. -- Rick Block (talk) 01:02, 2 October 2012 (UTC)

OK, I proposed a new way of trying to improve the article but no one liked it. I am happy to discuss the subject further in my user space with anyone interested but this is probably enough for the MHP talk page. The original dispute rumbles on unresolved. Martin Hogbin (talk) 08:23, 2 October 2012 (UTC)

Focus on the article

While I'm sure all this debate is fascinating, could I remind everyone that This is the talk page for discussing changes to the Monty Hall problem article itself. Please place discussions on the underlying mathematical issues on the Arguments page.

You guys are all starting to sound like a bunch of turkeycocks in a dust up - and this page is not for trying to persuade each other that your own approach to the problem is the right one. Can we return focus on the article. Does it in fact need any changes? Where does it need changes? What changes would make it better. Or do most of the folks here just prefer to keep this endless argument going? --Elen of the Roads (talk) 12:31, 5 October 2012 (UTC)

In particular, there's a proposal above [2] for new text for the "Solution" section. Is there anyone other than Martin who would be opposed to introducing this text into the article? It would of course be subject to normal editing policies, so the question is not "is this text perfect" but rather is this text an improvement over what's there now? -- Rick Block (talk) 15:39, 5 October 2012 (UTC)
I think the structure is excellent. I think the actual words could do with a few tweaks, but this can be done in the normal course of editing - none of them are a major rewrite. I think before the last picture it needs to say something about this is because Monty is selectively picking a goat, not picking the door at random, but that can be added afterwards if desired. --Elen of the Roads (talk) 15:53, 5 October 2012 (UTC)


I propose to add the table from citizendium.org plus this one (guest has selected door 1, host has shown a goat):
 Door 1    Door 2    Door 3    result if staying at door #1    result if switching to the door offered  
Car Goat Goat Car Goat
Goat Car Goat Goat Car
Goat Goat Car Goat Car
Immediately followed by showing that the paradox (chance to win by switching 2/3) only exists if the host is intentionally showing a goat, but if the host should randomly be opening just one of his two doors, be it a goat in 2 out of 3 or the be it the car in 1 out of 3, he would inevitably show the car in 1 out of 3, eliminating one half of the chances to win, assimilating the chance to win by switching to the chance to win by staying. To help the reader to see the causation why comparing the two still closed doors in the latter case is 1:1, but is 1:2 only if the host intentionally shows a goat in this famous paradox. Gerhardvalentin (talk) 17:22, 5 October 2012 (UTC)


Elen Rick, it is not good enough to ask, 'Is there anyone other than Martin who would be opposed to introducing this text into the article?'. Why do you not also ask, 'Is there anyone else who want's to add it?' as well? If there is a clear consensus then we should proceed. I have started a section below. — Preceding unsigned comment added by Martin Hogbin (talk • contribs)
True enough. Since you don't like it, you could actually say why not, though. Maybe it can be improved? Your comment to the first version was very vague, referring to past discussions, which are voluminous. -- Coffee2theorems (talk) 18:22, 5 October 2012 (UTC)
I'm not the one who said it. Read again. Elen of the Roads (talk) 12:56, 6 October 2012 (UTC)
Sorry Elen. My mistake. Martin Hogbin (talk)
Prompted by Rick's question, I have commented on that draft at the above linked section. It took me a while to compose my response, and I had not noticed intervening discussion of it in this thread. I encourage everybody to strive toward making these discussions easier to follow by (1) posting comments about a proposal or inquiry in the thread where it was posed, and (2) limiting comments under any thread to the putative subject of that thread. Following discussions about this article is excruciatingly difficult and, though perfection is unattainable, we can certainly do better. ~ Ningauble (talk) 18:49, 5 October 2012 (UTC)
Ninguable, I understood that we were seeking to establish a consensus (or not) for Coffee2theorems' proposal rather discuss it at great length, that is why I added the section below but please feel free to move the section to where you think it is best if you think that is important. Martin Hogbin (talk) 18:55, 5 October 2012 (UTC)
Martin, that was not directed to you individually. My point was that the purpose of this thread is the meta-issue of focusing on the article. It is entirely appropriate here to call attention to proposals which reflect that focus, as Rick did, but discussion about those proposals should be kept together with the proposals, and the introduction of new proposals should use new threads. ~ Ningauble (talk) 19:26, 5 October 2012 (UTC)

Symmetry

For the record, notwithstanding my earlier wisecrack about a five-dollar word, I do not really object to using it. My only reservation is that, although the shorthand "by symmetry" speaks loud and clear to mathematicians, and although laypersons appreciate the concept, some laypersons might not readily associate the concept with the shorthand. Nevertheless, it is a fundamental and readily accessible insight that greatly simplifies matters. ~ Ningauble (talk) 16:39, 4 October 2012 (UTC)
Since I have criticized the cryptic name-dropping of "symmetry" before, let me clarify: I'm not trying to ban that word from the English language or this article. It just seems to me that such cryptic name-dropping is sometimes proposed as a replacement for a proper explanation in cases where someone does not want the reader to actually understand something that is in the proposer's personal opinion too "academic".
It's easy enough to understand how this impression can arise: for example, you just basically said that it is appropriate to reduce the explanation of a fundamental insight into one word! I don't think that's reasonable outside of Zen koans. -- Coffee2theorems (talk) 20:24, 4 October 2012 (UTC)
I guess we need one of the non-mathematician editors to write a few sentences about the consequences of symmetry in words which other non-mathematicians will understand. I certainly don't use the word because I don't want the reader to understand something which I think is too academic! It's the complete opposite: I use the word because I do want the reader to understand something which is not academic at all; it's easy to understand, it's in fact so obvious that many take it for granted!
Take MHP with the usual K&W conditions, as for instance when we take a classical Bayesian approach: probabilities refer to our knowledge about the world, are they are equal when we have no information to the contrary (probability from symmetry!). It's now clear that the answer (switch or stay) can't depend on whether Vos Savant says "say, door 1, and say, door 3", or if she says "say door 3, and say, door 2". Or whatever else. There are altogether six questions she could have posed. They must all have the same answer. So we only need to compare "always switching" with "always staying". We know that the difference between these two strategies is the famous 2/3 versus 1/3. Since 2/3 is the probability of winning by switching and because of the just mentioned symmetry this probability can't depend on the case at hand - the probability of winning by switching is is 2/3, in every one of the six "versions" of Vos Savant's question (and if you want to use an expensive word here, you could say "conditional probability". But I'm trying to avoid expensive words which the layman doesn't grasp! That's exactly why I want the notion of symmetry brought to the fore! Richard Gill (talk) 04:39, 5 October 2012 (UTC)
We agree on a lot of things, then (also on avoiding the term "conditional probability" initially). However, what you have assumed here is not K&W but something different. I'd prefix your argument with: "If the problem is to have a unique answer at all..." Note that this implies K&W and the other way around (assuming figuring out the probability is a part of the answer, as is usually done). This assumption is not as obvious as it appears: see e.g. Bertrand paradox. Seeing where it leads and verifying that the result is consistent does provide one (partially) satisfying resolution to the paradox. Note that the point about consistency is not so obvious either: generally, one finds that "that a probability assignment can be made to respect some symmetry is trivial; that one can be made to respect all symmetries is contradictory". -- Coffee2theorems (talk) 07:35, 5 October 2012 (UTC)
The type of ambiguity found in Bertrands' paradox does not arise in discrete, finite problem spaces like the Monty Hall problem. There is no ambiguity about what equidistribution means when selecting "[uniformly] at random" from a finite set of discrete doors.

The argument from symmetry is not merely showing a result consistent with symmetry, but one that follows from symmetry. For the non-K&W assumptions, where we are uninformed about Monty's goat selection method, one can use the principle of indifference, which is quintessentially based on symmetry, and plug the numbers into Bayes' formula. This is completely standard Bayesian methodology. Or one can use the underlying symmetry directly to reason about the epistemic probability. For the K&W assumptions, where Monty's uninformative goat selection method is given, one can take the same numbers (though they have a different sense), and plug them into Bayes' formula. This is completely standard frequentist methodology. Or one can use the stated symmetry directly to reason about the frequentist statistics.

I would amend Richard Gill's remark above in one minor but significant respect: the symmetry and its consequence is in fact so blindingly obvious that many take it for granted; but it is only obvious after one recognizes that Monty is providing selective evidence about the two unchosen doors, not merely showing one of the three doors. The latter is evidently not at all obvious, as evidenced by the nearly universal error of thinking the final two closed doors are equivalent – people are seeing a symmetry that does not exist. ~ Ningauble (talk) 15:12, 5 October 2012 (UTC)

I did not mean something as restricted as "let's put a uniform distribution on X" (which will of course work), but something like "S(x) is a parameterized problem description with parameter x. If we plug in any value x from some set, the answer ought to be the same. Let's assume it is so!". In the MHP, S(x) is the problem statement and x is any permutation of (1, 2, 3). This is what Gill was talking about. ("six questions [...] must all have the same answer")
That approach is not in general consistent; you can get too many constraints. In this case it works, but as a general definition for what "the" answer to a vague problem is, it's not perfect. In the Bertrand paradox the symmetry approach actually also works (see Jaynes); the point was that it works a little too well! It uniquely resolves all ambiguities for a problem that many think is genuinely ambiguous. Whether that is a bug or a feature is a matter of opinion (Jaynes sure liked it).
IMO, the K&W assumptions are good, but mostly for a different reason (though I like the symmetry approach too; I'm more in the "feature" than "bug" camp). It's the best strategy for Monty (against a good player). Why do people assume the car could equally well be anywhere? Well, it would be a silly game if it was always behind door 1, now wouldn't it? Monty doesn't want you to have it easy (no fun!), so he makes it as difficult as he can (more exciting for everyone!). The intuitive way to do that is to randomize, to not give anything away. In this case it works. Look at the objections people have to a biased host: they don't want Monty giving you hints about the car location. Why not? Because it's assumed Monty doesn't want to give you the car. -- Coffee2theorems (talk) 18:14, 5 October 2012 (UTC)
The frequency distributions for car placement and goat selection in the K&W formulation are not assumptions, they are stipulations. As such they need no justification (beyond a requirement for internal consistency, e.g. you can't stipulate that Monty must show a goat and does show a car), they are to be taken as given. The article really cannot say anything about this version of the problem being right or wrong. The symmetries follow from these stipulations, they are not additional assumptions.

In my opinion, the reason many authors use K&W style stipulations is because the Bayesian principle of indifference cannot be used in frequentist statistics, and the stipulations provide the same probability inputs via explicit frequency distributions. It is very much a case of fitting the problem definition to the desired closed-form solution. (To quote Richard Hamming again: "The idea that theorems follow from the postulates does not correspond to simple observation. If the Pythagorean theorem were found to not follow from the postulates, we would again search for a way to alter the postulates until it was true. Euclid's postulates came from the Pythagorean theorem, not the other way around." – "The Unreasonable Effectiveness of Mathematics", The American Mathematical Monthly 87:2, February 1980, pp. 81-90.) It would be good to find a source that gives this reason explicitly enough that we can state it in the article. ~ Ningauble (talk) 20:50, 5 October 2012 (UTC)

I think we'ld make progress if everyone tried to write attractive (popular) descriptions of other people's favourite approaches. For instance: let Martin understand the point of symmetry and use it to compose a simple solution which at the same time is complete.
The choice is not between simple solutions which only talk about the chance of winning by switching, overall, and solutions which go through the motions of computing conditional probabilities by going back to the definition of conditional probability. The reason we are still quarelling here is because no-one sees that there are simple solutions which give conditional probabilities without even using the word "conditional". Instead, use the word "independent" or the word "symmetry". These are not expensive words - they are words connecting immediately to intuition. Richard Gill (talk) 04:39, 5 October 2012 (UTC)
Well, if no-one(!) sees such solutions, how do we know they exist. If you mean that the editors who claim the solution is essentially in terms of conditional probability, insist on using the term "conditional", you must have been sleeping part of the time, and myabe there all your dreams stem from. Me, nor Rick, want to use the term "conditional" in the first explanation, but for the discussion here we have to use the term to make sure it is understood. Using words like `'independent" or "symmetry" seems to me also to be undesirable. Nijdam (talk) 09:44, 5 October 2012 (UTC)
Perhaps the main source of the conflict lies in authors who have supported erroneous explanations and are not willing to admit this, and henceforth try to change the problem formulation in order to fit their way of solving. Nijdam (talk) 09:57, 5 October 2012 (UTC)
The "problem formulation" is told as a tricky story about the development of a one-time problem that results in a paradoxical situation:

Two still closed doors, one hiding the prize for sure, the other one hiding a nullity.

The paradox: But the chances on the prize of those two still closed doors are not 1:1, but chances are exactly 1:2 in this one-time show, caused by the development of that one-time show, where this famous paradox pops up.

But there are several divergent stories, where this paradox may never pop up. Gerhardvalentin (talk) 12:41, 5 October 2012 (UTC)

I'm convinced. Richard, you know very well all about the MHP. And, as you said above, the door numbers matter insofar, that there are six combinations that may be considered. all equivalent. Hence considering one is enough. And the symmetry (maybe we have to explain this in different wording) guarantees the answer is the same in all combinations. But just saying the overall chance on winning by switching is 2/3, is not enough as an explanation. In every combination the conditional (maybe we have to explain this in different wording) probabilities have the same value and hence have the same value as the overall chance. This would be a correct solution. Nijdam (talk) 11:14, 5 October 2012 (UTC)
Richard, I do not think that just saying the word symmetry gets us very far. To the general reader this is likely only to confuse. Most people's understanding of the word is restricted visual symmetry and similar.
I am not clear exactly what you mean by the word. We can take the W/vS statement to indicate symmetry with respect to door number and goat number by noting that no information is given which breaks this symmetry but this is just the Bayesian understanding of the problem, so why not just say that? Martin Hogbin (talk) 12:17, 5 October 2012 (UTC)
Sigh... Have you read my papers on this subject, Martin? Nijdam? Especially Martin? I am offering you a royal route to making the simple solutions complete and mathematically formalizable, by giving the completele and completely rigorous mathematical reason why the specific door numbers of any particular case are irrelevant under standard K&W assumptions. If a Nijdam complains about a simple solution, then you just have to retort: K&W conditions imply complete symmetry and this implies that the numbering of the doors is independent of the roles of the doors. Thus if we want to decide whether to switch or stay we can ignore the numbers written on the doors in the particular case at hand. The probability of winning by switching is 2/3, independently of which door numbers apply to the case at hand (for instance: "say, door 1, and say, door 3"). Only a masochist is going to waste time computing the conditional probability of winning by switching by going back to first principles, definition of conditional probability etc etc. After some calculations he'll find the answer 2/3, the same as the unconditional probability of winning by switching, but we knew in advance it would be the same. This is a legitimate simplification of the problem which most lay persons take automatically. According to a number of authorities it is a matter of taste whether or not one writes about this step explicitly. In my opinion, this is a matter which needs to be talked about explicitly in a first probability class in a university mathematics course, but is hardly of any interest or relevance to the lay person. Richard Gill (talk) 12:07, 8 October 2012 (UTC)
Retrieved from "https://wiki.riteme.site/w/index.php?title=Talk:Monty_Hall_problem/Archive_31&oldid=551512011"