Unit circle: Difference between revisions
ClueBot NG (talk | contribs) m Reverting possible vandalism by Peeliss to version by Yobot. False positive? Report it. Thanks, ClueBot NG. (1873311) (Bot) |
Pelinnnnnn (talk | contribs) dil |
||
| Line 1: | Line 1: | ||
Birim çember |
|||
<!--Hey can we get a picture (including the tangent values) of the points of interest on the unit circle with the tangent values shown also?-->[[Image:Unit circle.svg|Unit circle|right|thumb|186px|Illustration of a unit circle. The variable ''t'' is an [[angle]] measure.]] |
|||
Matematikte,yarıçapı bir birim olan çembere birim çember denir.Çoğunlukla,özellikle trigonometride,Öklid düzlemine göre Kartezyen koordinat sisteminde,merkezi orijin üzerinde (0,0) olan ve yarıçapı bir birim olan çemberdir.Birim çember sıklıkla ''S''<sup>1</sup>; olarak ifade edilir.Genellikle daha büyük boyutları ise birim küredir. |
|||
In [[mathematics]], a '''unit circle''' is a [[circle]] with a [[radius]] of [[1 (number)|one]]. Frequently, especially in [[trigonometry]], the unit circle is the circle of radius one centered at the origin (0, 0) in the [[Cartesian coordinate system]] in the [[Euclidean plane]]. The unit circle is often denoted ''S''<sup>1</sup>; the generalization to higher dimensions is the [[unit sphere]]. |
|||
(x,y) birim çember üzerinde bir nokta olduğunda, |x| ve |y|,dik olan ve hipotenüsü bir olan üçgenin diğer kenar uzunluklarıdır.Bu nedenle,Pisagor teoremine göre,x ve y bu denklemi karşılamaktadır, |
|||
If (''x'', ''y'') is a point on the unit circle, then |''x''| and |''y''| are the lengths of the legs of a [[right triangle]] whose hypotenuse has length 1. Thus, by the [[Pythagorean theorem]], ''x'' and ''y'' satisfy the equation |
|||
:<math>x^2 + y^2 = 1.</math> |
:<math>x^2 + y^2 = 1.</math> |
||
Bir birim çember örneklemesidir.t değeri ölçülen açının değerine eşittir. |
|||
[[Image:Unit circle.svg|Unit circle|right|thumb|186px|bir birim çemberin çizimi. değişkeni ''t''olan bir açı ölçer.]] |
|||
Since ''x''² = (−''x'')² for all ''x'', and since the reflection of any point on the unit circle about the ''x''- or ''y''-axis is also on the unit circle, the above equation holds for all points (''x'', ''y'') on the unit circle, not only those in the first quadrant. |
|||
Bütün x değerleri için ''x''² = (−''x'')² olduğu için,birim çember üzerinde x ve y eksenlernin herhangi bir noktası yine birim çember üzerindedir.Yalnızca birinci bölgedeki değil,birim çember üzerinde alınan bütün noktalar(x,y) bu denklemi sağlamaktadır. |
|||
Ayrıca,diğer diğer birim çemberleri tanımlamak için farklı uzaklık kavramları da kullanılabilir;Rieman çemberi gibi.Extra örnekler için matematik standartlarındaki başlıklara bakabilirsin. |
|||
One may also use other notions of "distance" to define other "unit circles", such as the [[Riemannian circle]]; see the article on [[norm (mathematics)|mathematical norms]] for additional examples. |
|||
==Karmaşık düzlemlerde== |
|||
Birim çember,karmaşık sayıların temeli olarak düşünebiliriz. |
|||
==In the complex plane== |
|||
The unit circle can be considered as the set of [[complex numbers]] ''z'' of the form |
|||
:<math> z = \,\mathrm{e}^{i t}\, = \cos(t) + i \sin(t) \,</math> |
:<math> z = \,\mathrm{e}^{i t}\, = \cos(t) + i \sin(t) \,</math> |
||
Bu formül Euler eşitliğidir. |
|||
for all ''t''. This relation is [[Euler's formula]]. |
|||
==Birim çemberde trigonometrik fonksiyonlar== |
|||
Bir trigonometrik fonksiyon olan cosinüs and sinüs birim çember üzerinde tanımlanabilir.(x,y) birim çember üzerinde bir nokta olsun, origin(0,0) ve (x,y) arasında oluşturulan çizgi pozitif x ekseninden bir t açısı oluşturur(saat yönünün tersinde döndüğünde positif yöndedir). |
|||
==Trigonometric functions on the unit circle== |
|||
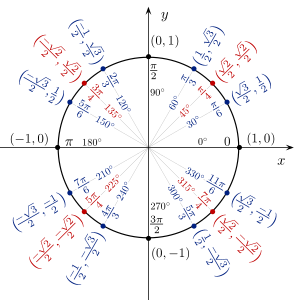
[[Image:Circle-trig6.svg|right|thumb|300px|'' |
[[Image:Circle-trig6.svg|right|thumb|300px|açısı''θ'' olan bütün trigonometrik fonksiyonlar merkezi 0 olan birim çember geometrik olarak oluşturulabilir.]] |
||
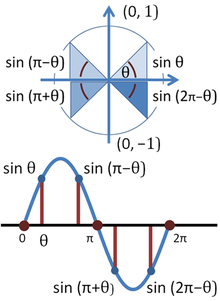
[[File:Periodic sine.PNG|thumb| |
[[File:Periodic sine.PNG|thumb|birim çemberde sinüs fonkisiyonu ve grafiği)]] |
||
The [[trigonometric function]]s cosine and sine may be defined on the unit circle as follows. If (''x'', ''y'') is a point of the unit circle, and if the ray from the origin (0, 0) to (''x'', ''y'') makes an [[angle]] ''t'' from the positive ''x''-axis, (where counterclockwise turning is positive), then |
|||
:<math>\cos(t) = x \,\!</math> |
:<math>\cos(t) = x \,\!</math> |
||
:<math>\sin(t) = y. \,\!</math> |
:<math>\sin(t) = y. \,\!</math> |
||
Bu denklem ''x''<sup>2</sup> + ''y''<sup>2</sup> = 1 şu bağıntıyı verir |
|||
:<math> \cos^2(t) + \sin^2(t) = 1. \,\!</math> |
:<math> \cos^2(t) + \sin^2(t) = 1. \,\!</math> |
||
Birim çember ayrıca sinüs ve cosinüs fonksiyonlarının periyodik fonksiyon olduklarını da gösterir, |
|||
The unit circle also demonstrates that [[sine]] and [[cosine]] are [[periodic function]]s, with the identities |
|||
:<math>\cos t = \cos(2\pi k+t) \,\!</math> |
:<math>\cos t = \cos(2\pi k+t) \,\!</math> |
||
:<math>\sin t = \sin(2\pi k+t) \,\!</math> |
:<math>\sin t = \sin(2\pi k+t) \,\!</math> |
||
Herhangi bir k tamsayısı için. |
|||
for any [[integer]] ''k''. |
|||
Birim çember üzerinde kurulan üçgenler de trigonometrik fonksiyonların periyodikliğini göstermek için kulanılabilir.birim çember üzerinde seçilen bir P(x,y) noktası originle QA yarıçapını oluşturmaktadır ve pozitif x ekseni kolunda bir t açısına 0 < ''t'' < π/2 sahiptir.şimdi bir Q(''x''<sub>1</sub>,0) noktası düşünün,kesişimleri PQ <math>\perp</math> OQ.Sonuç,bir dik üçgendir ΔOPQ ile ∠QOP = ''t''.Çünkü,PQ ''y''<sub>1</sub> uzunluğuna, OQ ''x''<sub>1</sub> uzunluğuna ve QA’nın uzunluğu 1’dir, |
|||
sin(''t'') = ''y''<sub>1</sub> and cos(''t'') = ''x''<sub>1</sub>.Bu eşdeğerliğini kuran,OR yarıçaplı aynı açılı çember üzerinde bir nokta olan R(−''x''<sub>1</sub>,''y''<sub>1</sub>) x ekseninin negatif kolundadır.Şimdi bir nokta düşünün S (''−x<sub>1</sub>'',0) ve kesişimleri RS <math>\perp</math> OS. Sonuç bir dik üçgendir ΔORS ile ∠SOR = ''t''.Bu,bu nedenle görülebilir,çünkü ∠ROQ = π−''t'', R (cos(π−''t'')noktası,sin(π−''t'')) aynı yöntemle P (cos(''t''),sin(''t''))noktasıdır.Bunun sonucu olarak, (−''x''<sub>1</sub>,''y''<sub>1</sub>) ifadesi (cos(π−''t''),sin(π−''t'')) ifadesine ve (''x''<sub>1</sub>,''y''<sub>1</sub>) ifadesi de (cos(''t''),sin(''t'')) bu ifadeye denktir.Bu doğru sin(''t'') = sin(π−''t'') ve −cos(''t'') = cos(π−''t''). Bu benzer bir tarzla anlamlandırılabilir tan(π−''t'') = −tan(''t'') bu yüzden, tan(''t'') = ''y''<sub>1</sub>/''x''<sub>1</sub> and tan(π−''t'') = ''y''<sub>1</sub>/(−''x''<sub>1</sub>).yukarıdaki basit gösterim bir denklemde görülebilir sin(π/4) = sin(3π/4) = 1/sqrt(2). |
|||
Dik bir üçgen,sinüs,cosinüs ve diğer trigonometric fonksiyonlarla çalışıldığında yalnızca 0’dan büyük π/2’den küçük olan açılar anlamlandırılabilir.Ancak,birim çember ile tanımlanmış bu işlevler için ölçülen açısı 2π den büyük olanlarda bile bu gerçek değerleri elde etmek mümkündür.Aslında,altı standart trigonometrik fonksiyonlar; sinüs,cosinüs,tanjant,kotanjant,sekant,cosecant gibi arkaik fonksiyonları versine ve exsecant ,sağda gösterildiği gibi bir birim çemberin açısından geometrik olarak tanımlanabilir. |
|||
Birim çember kullanarak,birçok açı için herhangi bir trigonometrik fonksiyon değeri ,toplam ve fark formüllerini kullanarak bir hesap makinesi kullanmadan hesaplanabilir. |
|||
When working with right triangles, sine, cosine, and other trigonometric functions only make sense for angle measures more than zero and less than π/2. However, when defined with the unit circle, these functions produce meaningful values for any [[real number|real]]-valued angle measure – even those greater than 2π. In fact, all six standard trigonometric functions – sine, cosine, tangent, cotangent, secant, and cosecant, as well as archaic functions like [[versine]] and [[exsecant]] – can be defined geometrically in terms of a unit circle, as shown at right. |
|||
| ⚫ | |||
==Çember grubu== |
|||
Using the unit circle, the values of any trigonometric function for many angles other than those labeled can be calculated without the use of a calculator by using the [[Trigonometric identity#Angle sum and difference identities|Sum and Difference Formulas]]. |
|||
Kompleks sayılar Öklid düzlemi üzerindeki noktalar ile tespit edilebilir.Yani, ''a'' + ''bi'' sayısı (''a'', ''b'') noktası olarak tanımlanabilir.Bu tanımlama altında ,birim çember ,çember grubu diye bilinen çarpmanın altında bir gruptur.Düzlemde çarpma <math>\cos \theta + i \sin \theta</math> &theta açısıyla saat yönünün tersinde bir dönme oluşturur.Bu grup matematikte ve bilimde önemli uygulamalara sahiptir. |
|||
==Karmaşık düzlemlerde== |
|||
| ⚫ | |||
==Circle group== |
|||
[[Complex number]]s can be identified with points in the [[Euclidean plane]], namely the number ''a'' + ''bi'' is identified with the point (''a'', ''b''). Under this identification, the unit circle is a [[group (mathematics)|group]] under multiplication, called the [[circle group]]. On the plane multiplication by <math>\cos \theta + i \sin \theta</math> gives a counterclockwise rotation by θ. This group has important applications in mathematics and science.{{Such as?}} |
|||
==Complex dynamics== |
|||
{{Main|Complex dynamics}} |
{{Main|Complex dynamics}} |
||
[[Image:Erays.png|right|thumb| |
[[Image:Erays.png|right|thumb|kompleks dinamiklerde birim çember]] |
||
Julia seti ve ayrık olmayan dinamik sistemi ile evrim fonksiyonu : |
|||
[[Julia set]] of [[Dynamical system (definition)|discrete nonlinear dynamical system]] with [[evolution function]]: |
|||
:<math>f_0(x) = x^2 \,</math> |
:<math>f_0(x) = x^2 \,</math> |
||
Bu bir birim çemberdir.Bu,yaygın olarak dinamik sistemlerin çalışmasında kullanılan çok basit bir durumdur. |
|||
==Referanslar== |
|||
*[http://wiki.riteme.site/wiki/Jacobi_identity İngilizce vikipedi] |
|||
==Dış bağlantılar== |
|||
is a unit circle. It is a simplest case so it is widely used in study of dynamical systems. |
|||
==See also== |
|||
*[[Angle|Angle measure]] |
|||
*[[Circle group]] |
|||
*[[Pythagorean trigonometric identity]] |
|||
*[[Riemannian circle]] |
|||
*[[Unit disk]] |
|||
*[[Unit sphere]] |
|||
*[[Unit hyperbola]] |
|||
*[[Unit square]] |
|||
*[[Z-transform]] |
|||
==External links== |
|||
{{Wikibooks|Trigonometry/The unit circle}} |
{{Wikibooks|Trigonometry/The unit circle}} |
||
{{Wiktionary|unit circle}} |
{{Wiktionary|unit circle}} |
||
| Line 70: | Line 46: | ||
*[http://www.dudefree.com/unitcircle/ Flash animation for learning the unit circle] |
*[http://www.dudefree.com/unitcircle/ Flash animation for learning the unit circle] |
||
*[http://glab.trixon.se/ GonioLab]: Visualization of the unit circle, trigonometric and hyperbolic functions |
*[http://glab.trixon.se/ GonioLab]: Visualization of the unit circle, trigonometric and hyperbolic functions |
||
[[Category:Circles]] |
|||
[[Category:One]] |
|||
[[Category:Trigonometry]] |
|||
[[Category:Fourier analysis]] |
|||
[[Category:Analytic geometry]] |
|||
Revision as of 07:29, 13 June 2014
Birim çember Matematikte,yarıçapı bir birim olan çembere birim çember denir.Çoğunlukla,özellikle trigonometride,Öklid düzlemine göre Kartezyen koordinat sisteminde,merkezi orijin üzerinde (0,0) olan ve yarıçapı bir birim olan çemberdir.Birim çember sıklıkla S1; olarak ifade edilir.Genellikle daha büyük boyutları ise birim küredir. (x,y) birim çember üzerinde bir nokta olduğunda, |x| ve |y|,dik olan ve hipotenüsü bir olan üçgenin diğer kenar uzunluklarıdır.Bu nedenle,Pisagor teoremine göre,x ve y bu denklemi karşılamaktadır,
Bir birim çember örneklemesidir.t değeri ölçülen açının değerine eşittir.

Bütün x değerleri için x² = (−x)² olduğu için,birim çember üzerinde x ve y eksenlernin herhangi bir noktası yine birim çember üzerindedir.Yalnızca birinci bölgedeki değil,birim çember üzerinde alınan bütün noktalar(x,y) bu denklemi sağlamaktadır. Ayrıca,diğer diğer birim çemberleri tanımlamak için farklı uzaklık kavramları da kullanılabilir;Rieman çemberi gibi.Extra örnekler için matematik standartlarındaki başlıklara bakabilirsin.
Karmaşık düzlemlerde
Birim çember,karmaşık sayıların temeli olarak düşünebiliriz.
Bu formül Euler eşitliğidir.
Birim çemberde trigonometrik fonksiyonlar
Bir trigonometrik fonksiyon olan cosinüs and sinüs birim çember üzerinde tanımlanabilir.(x,y) birim çember üzerinde bir nokta olsun, origin(0,0) ve (x,y) arasında oluşturulan çizgi pozitif x ekseninden bir t açısı oluşturur(saat yönünün tersinde döndüğünde positif yöndedir).

Bu denklem x2 + y2 = 1 şu bağıntıyı verir
Birim çember ayrıca sinüs ve cosinüs fonksiyonlarının periyodik fonksiyon olduklarını da gösterir,
Herhangi bir k tamsayısı için. Birim çember üzerinde kurulan üçgenler de trigonometrik fonksiyonların periyodikliğini göstermek için kulanılabilir.birim çember üzerinde seçilen bir P(x,y) noktası originle QA yarıçapını oluşturmaktadır ve pozitif x ekseni kolunda bir t açısına 0 < t < π/2 sahiptir.şimdi bir Q(x1,0) noktası düşünün,kesişimleri PQ OQ.Sonuç,bir dik üçgendir ΔOPQ ile ∠QOP = t.Çünkü,PQ y1 uzunluğuna, OQ x1 uzunluğuna ve QA’nın uzunluğu 1’dir, sin(t) = y1 and cos(t) = x1.Bu eşdeğerliğini kuran,OR yarıçaplı aynı açılı çember üzerinde bir nokta olan R(−x1,y1) x ekseninin negatif kolundadır.Şimdi bir nokta düşünün S (−x1,0) ve kesişimleri RS OS. Sonuç bir dik üçgendir ΔORS ile ∠SOR = t.Bu,bu nedenle görülebilir,çünkü ∠ROQ = π−t, R (cos(π−t)noktası,sin(π−t)) aynı yöntemle P (cos(t),sin(t))noktasıdır.Bunun sonucu olarak, (−x1,y1) ifadesi (cos(π−t),sin(π−t)) ifadesine ve (x1,y1) ifadesi de (cos(t),sin(t)) bu ifadeye denktir.Bu doğru sin(t) = sin(π−t) ve −cos(t) = cos(π−t). Bu benzer bir tarzla anlamlandırılabilir tan(π−t) = −tan(t) bu yüzden, tan(t) = y1/x1 and tan(π−t) = y1/(−x1).yukarıdaki basit gösterim bir denklemde görülebilir sin(π/4) = sin(3π/4) = 1/sqrt(2). Dik bir üçgen,sinüs,cosinüs ve diğer trigonometric fonksiyonlarla çalışıldığında yalnızca 0’dan büyük π/2’den küçük olan açılar anlamlandırılabilir.Ancak,birim çember ile tanımlanmış bu işlevler için ölçülen açısı 2π den büyük olanlarda bile bu gerçek değerleri elde etmek mümkündür.Aslında,altı standart trigonometrik fonksiyonlar; sinüs,cosinüs,tanjant,kotanjant,sekant,cosecant gibi arkaik fonksiyonları versine ve exsecant ,sağda gösterildiği gibi bir birim çemberin açısından geometrik olarak tanımlanabilir. Birim çember kullanarak,birçok açı için herhangi bir trigonometrik fonksiyon değeri ,toplam ve fark formüllerini kullanarak bir hesap makinesi kullanmadan hesaplanabilir.
Çember grubu
Kompleks sayılar Öklid düzlemi üzerindeki noktalar ile tespit edilebilir.Yani, a + bi sayısı (a, b) noktası olarak tanımlanabilir.Bu tanımlama altında ,birim çember ,çember grubu diye bilinen çarpmanın altında bir gruptur.Düzlemde çarpma &theta açısıyla saat yönünün tersinde bir dönme oluşturur.Bu grup matematikte ve bilimde önemli uygulamalara sahiptir.
Karmaşık düzlemlerde

Julia seti ve ayrık olmayan dinamik sistemi ile evrim fonksiyonu :
Bu bir birim çemberdir.Bu,yaygın olarak dinamik sistemlerin çalışmasında kullanılan çok basit bir durumdur.
Referanslar
Dış bağlantılar
- Weisstein, Eric W. "Unit circle". MathWorld.
- Flash animation for learning the unit circle
- GonioLab: Visualization of the unit circle, trigonometric and hyperbolic functions










