Triangle: Difference between revisions
m Reverted edits by 75.172.235.190 to last revision by The Thing That Should Not Be (HG (Custom)) |
|||
| Line 1: | Line 1: | ||
{{about|the basic geometric shape}} |
|||
Black people are called Niggers. White power, ya coons. Even your little IP bans can't stop me. |
|||
{{Redirect|Isosceles|the trapezoid|Isosceles trapezoid}} |
|||
{{Infobox Polygon |
|||
| name = Triangle |
|||
| image = Triangle illustration.svg |
|||
| caption = A triangle |
|||
| edges = 3 |
|||
| schläfli = {3} |
|||
| coxeter = |
|||
| symmetry = |
|||
| area = |
|||
| angle = |
|||
| properties = |
|||
}} |
|||
A '''triangle''' is one of the basic [[shape]]s of [[geometry]]: a [[polygon]] with three corners or [[Vertex (geometry)|vertices]] and three sides or edges which are [[line segment]]s. A triangle with vertices ''A'', ''B'', and ''C'' is denoted {{trianglenotation|ABC}}. |
|||
In [[Euclidean geometry]] any three non-[[collinear]] points determine a unique triangle and a unique [[Plane (mathematics)|plane]] (i.e. a two-dimensional [[Euclidean space]]). |
|||
== Types of triangles ==<!-- This section is linked from [Pythagorean theorem] --> |
|||
=== By relative lengths of sides === |
|||
Triangles can be classified according to the relative lengths of their sides: |
|||
* In an '''equilateral triangle''' all sides have the same length. An equilateral triangle is also a [[regular polygon]] with all angles measuring 60°.<ref>{{MathWorld|title=Equilateral Triangle|urlname=EquilateralTriangle}}</ref> |
|||
* In an '''isosceles triangle''', two sides are equal in length.<ref>Euclid defines isosceles triangles based on the number of equal sides, i.e. ''only two equal sides''. An alternative approach defines isosceles triangles based on shared properties, i.e. ''equilateral triangles are a special case of isosceles triangles''. [http://en.wiktionary.org/wiki/isosceles_triangle Wiktionary definition of isosceles triangle], {{MathWorld|title=Isosceles triangle|urlname=IsoscelesTriangle}}</ref> An isosceles triangle also has two angles of the same measure; namely, the angles opposite to the two sides of the same length; this fact is the content of the [[Isosceles triangle theorem]]. Some definitions state that isosceles triangles have only two equal sides, whereas others state that an isosceles triangle is one with ''at least'' two equal sides.<ref>{{MathWorld|title=Isosceles Triangle|urlname=IsoscelesTriangle}}</ref> The latter definition would make all equilateral triangles isosceles triangles. |
|||
* In a '''scalene triangle''', all sides are unequal.<ref>{{MathWorld|title=Scalene triangle|urlname=ScaleneTriangle}}</ref> The three angles are also all different in measure. |
|||
<table align="center"><tr align="center">. |
|||
<td>[[Image:Triangle.Equilateral.svg|Equilateral Triangle]]</td> |
|||
<td width="125">[[Image:Triangle.Isosceles.svg|Isosceles triangle]]</td> |
|||
<td>[[Image:Triangle.Scalene.svg|Scalene triangle]]</td> |
|||
</tr> |
|||
<tr align="center"> |
|||
<td>Equilateral</td><td>Isosceles</td><td>Scalene</td> |
|||
</tr> |
|||
</table> |
|||
=== By internal angles === |
|||
Triangles can also be classified according to their [[internal angle]]s, measured here in [[degree (angle)|degree]]s. |
|||
* A '''right triangle''' (or '''right-angled triangle''', formerly called a '''rectangled triangle''') has one of its interior angles measuring 90° (a [[angle|right angle]]). The side opposite to the right angle is the [[hypotenuse]]; it is the longest side of the right triangle. The other two sides are called the ''legs'' or '''catheti'''<ref> |
|||
{{cite book | last1=Zeidler | first1=Eberhard | title=Oxford User's Guide to Mathematics | publisher=[[Oxford University Press]] | isbn=978-0-19-850763-5 | year=2004 | page=729 }} |
|||
</ref> (singular: '''[[wiktionary:cathetus|cathetus]]''') of the triangle. Right triangles obey the [[Pythagorean theorem]]: the sum of the squares of the lengths of the two legs is equal to the square of the length of the hypotenuse: ''a''<sup>2</sup> + ''b''<sup>2</sup> = ''c''<sup>2</sup>, where ''a'' and ''b'' are the lengths of the legs and ''c'' is the length of the hypotenuse. [[Special right triangles]] are right triangles with additional properties that make calculations involving them easier. The most famous is the 3-4-5 right triangle, where ''3''<sup>2</sup> + ''4''<sup>2</sup> = ''5''<sup>2</sup>. In this situation, 3, 4, and 5 are a [[Pythagorean Triple]]. |
|||
* Triangles that do not have an angle that measures 90° are called '''oblique triangles'''. |
|||
* A triangle that has all interior angles measuring less than 90° is an '''acute triangle''' or '''acute-angled triangle'''. |
|||
* A triangle that has one angle that measures more than 90° is an '''obtuse triangle''' or '''obtuse-angled triangle'''. |
|||
A triangle that has two angles with the same measure also has two sides with the same length, and therefore it is an isosceles triangle. It follows that in a triangle where all angles have the same measure, all three sides have the same length, and therefore such triangle is equilateral. |
|||
<table align="center"> |
|||
<tr align="center"> |
|||
<td>[[Image:Triangle.Right.svg|Right triangle]]</td> |
|||
<td width="185">[[Image:Triangle.Obtuse.svg|Obtuse triangle]]</td> |
|||
<td width="185">[[Image:Triangle.Acute.svg|Acute triangle]]</td> |
|||
</tr> |
|||
<tr align="center"> |
|||
<td>Right</td><td>Obtuse</td><td>Acute</td> |
|||
</tr> |
|||
<tr align="center"> |
|||
<td> </td><td colspan="2" align="center"><math>\underbrace{\qquad \qquad \qquad \qquad \qquad \qquad}_{}</math></td> |
|||
</tr> |
|||
<tr align="center> |
|||
<td> </td><td colspan="2" align="center">Oblique</td> |
|||
</tr> |
|||
</table> |
|||
== Basic facts == |
|||
Triangles are assumed to be two-[[dimension]]al [[plane figure]]s, unless the context provides otherwise (see [[Triangle#Non-planar_triangles|Non-planar triangles]], below). In rigorous treatments, a triangle is therefore called a ''2-[[simplex]]'' (see also [[Polytope]]). Elementary facts about triangles were presented by [[Euclid]] in books 1–4 of his ''[[Euclid's Elements|Elements]]'', around 300 BC. |
|||
[[File:Remint3.svg|340px|right|thumb|A triangle, showing exterior angle d]] |
|||
The measures of the interior angles of a triangle in [[Euclidean space]] always add up to 180 degrees. This allows determination of the measure of the third angle of any triangle given the measure of two angles. An ''[[interior angle|exterior angle]]'' of a triangle is an angle that is a linear pair (and hence [[supplementary angle|supplementary]]) to an interior angle. The measure of an exterior angle of a triangle is equal to the sum of the measures of the two interior angles that are not adjacent to it; this is the [[exterior angle theorem]]. The sum of the measures of the three exterior angles (one for each vertex) of any triangle is 360 degrees.<ref>The ''n'' external angles of any ''n''-sided [[Wiktionary:Convex|convex]] polygon add up to 360 degrees.</ref> |
|||
The sum of the lengths of any two sides of a triangle always exceeds the length of the third side, a principle known as the ''[[triangle inequality]]''. Since the vertices of a triangle are assumed to be non-collinear, it is not possible for the sum of the length of two sides be equal to the length of the third side. |
|||
Two triangles are said to be ''[[similarity (geometry)|similar]]'' if every angle of one triangle has the same measure as the corresponding angle in the other triangle. The corresponding sides of similar triangles have lengths that are in the same proportion, and this property is also sufficient to establish similarity. |
|||
A few basic [[theorem]]s about similar triangles: |
|||
* If two corresponding internal angles of two triangles have the same measure, the triangles are similar. |
|||
* If two corresponding sides of two triangles are in proportion, and their included angles have the same measure, then the triangles are similar. (The ''included angle'' for any two sides of a polygon is the internal angle between those two sides.) |
|||
* If three corresponding sides of two triangles are in proportion, then the triangles are similar.<ref>Again, in all cases "mirror images" are also similar.</ref> |
|||
Two triangles that are [[Congruence (geometry)|congruent]] have exactly the same size and shape:<ref>All pairs of congruent triangles are also similar; but not all pairs of similar triangles are congruent.</ref> all pairs of corresponding interior angles are equal in measure, and all pairs of corresponding sides have the same length. (This is a total of six equalities, but three are often sufficient to prove congruence.) |
|||
Some '''[[sufficient condition]]s for a pair of triangles to be congruent''' are: |
|||
* SAS Postulate: Two sides in a triangle have the same length as two sides in the other triangle, and the included angles have the same measure. |
|||
* ASA: Two interior angles and the included side in a triangle have the same measure and length, respectively, as those in the other triangle. (The ''included side'' for a pair of angles is the side that is common to them.) |
|||
* SSS: Each side of a triangle has the same length as a corresponding side of the other triangle. |
|||
* AAS: Two angles and a <u>corresponding</u> (non-included) side in a triangle have the same measure and length, respectively, as those in the other triangle. |
|||
* Hypotenuse-Leg (HL) Theorem: The hypotenuse and a leg in a right triangle have the same length as those in another right triangle. |
|||
* Hypotenuse-Angle Theorem: The hypotenuse and an acute angle in one right triangle have the same length and measure, respectively, as those in the other right triangle. This is just a particular case of the AAS theorem. |
|||
An important case: |
|||
* Side-Side-Angle (or Angle-Side-Side) condition: If two sides and a corresponding non-included angle of a triangle have the same length and measure, respectively, as those in another triangle, then this is ''not'' sufficient to prove congruence; but if the angle given is opposite to the longer side of the two sides, then the triangles are congruent. The Hypothenuse-Leg Theorem is a particular case of this criterion. The Side-Side-Angle condition does not by itself guarantee that the triangles are congruent because one triangle could be obtuse-angled and the other acute-angled. |
|||
Using right triangles and the concept of similarity, the [[trigonometric function]]s sine and cosine can be defined. These are functions of an [[angle]] which are investigated in [[trigonometry]]. |
|||
[[Image:Pythagorean.svg|Pythagorean.svg|thumb|The Pythagorean theorem]] |
|||
A central theorem is the [[Pythagorean theorem]], which states in any right triangle, the square of the length of the [[hypotenuse]] equals the sum of the squares of the lengths of the two other sides. If the hypotenuse has length ''c'', and the legs have lengths ''a'' and ''b'', then the theorem states that |
|||
:<math>a^2 + b^2 = c^2.\,</math> |
|||
The converse is true: if the lengths of the sides of a triangle satisfy the above equation, then the triangle has a right angle opposite side ''c''. |
|||
Some other facts about right triangles: |
|||
* The acute angles of a right triangle are [[Complementary angles|complementary]]. |
|||
:<math>a + b + 90^{\circ} = 180^{\circ} \Rightarrow a + b = 90^{\circ} \Rightarrow a = 90^{\circ} - b</math> |
|||
* If the legs of a right triangle have the same length, then the angles opposite those legs have the same measure. Since these angles are complementary, it follows that each measures 45 degrees. By the Pythagorean theorem, the length of the hypotenuse is the length of a leg times √2. |
|||
* In a right triangle with acute angles measuring 30 and 60 degrees, the hypotenuse is twice the length of the shorter side, and the longer side is equal to the length of the shorter side times √3 : |
|||
::<math>c = 2a\,</math> |
|||
::<math>b = a\times\sqrt{3}.</math> |
|||
For all triangles, angles and sides are related by the [[law of cosines]] and [[law of sines]] (also called the ''cosine rule'' and ''sine rule''). |
|||
== Points, lines and circles associated with a triangle == |
|||
There are hundreds of different constructions that find a special point associated with (and often inside) a triangle, satisfying some unique property: see the references section for a catalogue of them. Often they are constructed by finding three lines associated in a symmetrical way with the three sides (or vertices) and then proving that the three lines meet in a single point: an important tool for proving the existence of these is [[Ceva's theorem]], which gives a criterion for determining when three such lines are [[concurrent lines|concurrent]]. Similarly, lines associated with a triangle are often constructed by proving that three symmetrically constructed points are [[collinear]]: here [[Menelaus' theorem]] gives a useful general criterion. In this section just a few of the most commonly-encountered constructions are explained. |
|||
[[Image:Triangle.Circumcenter.svg|thumb|right|The [[circumcenter]] is the center of a circle passing through the three vertices of the triangle.]] |
|||
A [[bisection|perpendicular bisector]] of a triangle is a straight line passing through the [[midpoint]] of a side and being perpendicular to it, i.e. forming a right angle with it. The three perpendicular bisectors meet in a single point, the triangle's [[circumcenter]]; this point is the center of the [[circumcircle]], the [[circle]] passing through all three vertices. The diameter of this circle can be found from the law of sines stated above. |
|||
[[Thales' theorem]] implies that if the circumcenter is located on one side of the triangle, then the opposite angle is a right one. If the circumcenter is located inside the triangle, then the triangle is acute; if the circumcenter is located outside the triangle, then the triangle is obtuse. |
|||
[[Image:Triangle.Orthocenter.svg|thumb|left|The intersection of the altitudes is the [[orthocenter]].]] |
|||
An [[altitude (triangle)|altitude]] of a triangle is a straight line through a vertex and perpendicular to (i.e. forming a right angle with) the opposite side. This opposite side is called the ''base'' of the altitude, and the point where the altitude intersects the base (or its extension) is called the ''foot'' of the altitude. The length of the altitude is the distance between the base and the vertex. The three altitudes intersect in a single point, called the [[orthocenter]] of the triangle. The orthocenter lies inside the triangle if and only if the triangle is acute. |
|||
[[Image:Triangle.Incircle.svg|thumb|right|The intersection of the angle bisectors is the center of the [[incircle]].]] |
|||
An [[angle bisector]] of a triangle is a straight line through a vertex which cuts the corresponding angle in half. The three angle bisectors intersect in a single point, the [[incenter]], the center of the triangle's [[incircle]]. The incircle is the circle which lies inside the triangle and touches all three sides. There are three other important circles, the [[excircle]]s; they lie outside the triangle and touch one side as well as the extensions of the other two. The centers of the in- and excircles form an [[orthocentric system]]. |
|||
<br clear=left> |
|||
[[Image:Triangle.Centroid.svg|thumb|left|The intersection of the medians is the [[centroid]].]] |
|||
A [[median (geometry)|median]] of a triangle is a straight line through a [[vertex (geometry)|vertex]] and the [[midpoint]] of the opposite side, and divides the triangle into two equal areas. The three medians intersect in a single point, the triangle's [[centroid]]. The centroid of a rigid triangular object (cut out of a thin sheet of uniform density) is also its [[center of gravity]]: the object can be balanced on its centroid. The centroid cuts every median in the ratio 2:1, i.e. the distance between a vertex and the centroid is twice the distance between the centroid and the midpoint of the opposite side. |
|||
[[Image:Triangle.NinePointCircle.svg|thumb|right|[[Nine-point circle]] demonstrates a symmetry where six points lie on the edge of the triangle.]] |
|||
The midpoints of the three sides and the feet of the three altitudes all lie on a single circle, the triangle's [[nine-point circle]]. The remaining three points for which it is named are the midpoints of the portion of altitude between the vertices and the [[orthocenter]]. The radius of the nine-point circle is half that of the circumcircle. It touches the incircle (at the [[Feuerbach point]]) and the three [[excircle]]s. |
|||
<br clear=left> |
|||
[[Image:Triangle.EulerLine.svg|thumb|left|[[Euler's line]] is a straight line through the centroid (orange), orthocenter (blue), circumcenter (green) and center of the nine-point circle (red).]] |
|||
The centroid (yellow), orthocenter (blue), circumcenter (green) and barycenter of the nine-point circle (red point) all lie on a single line, known as [[Euler's line]] (red line). The center of the nine-point circle lies at the midpoint between the orthocenter and the circumcenter, and the distance between the centroid and the circumcenter is half that between the centroid and the orthocenter. |
|||
The center of the incircle is not in general located on Euler's line. |
|||
If one reflects a median at the angle bisector that passes through the same vertex, one obtains a [[symmedian]]. The three symmedians intersect in a single point, the [[symmedian point]] of the triangle. |
|||
<br clear=all> |
|||
== Computing the area of a triangle == |
|||
[[Image:Triangle.GeometryArea.svg|300px|thumb|The area of a triangle can be demonstrated as half of the area of a [[paralellogram]] which has the same base length and height.]] |
|||
Calculating the area of a triangle is an elementary problem encountered often in many different situations. The best known and simplest formula is: |
|||
:<math>\mathrm{Area}=\frac{1}{2}bh</math> |
|||
where ''b'' is the length of the base of the triangle, and ''h'' is the height or altitude of the triangle. The term 'base' denotes any side, and 'height' denotes the length of a perpendicular from the point opposite the side onto the side itself. |
|||
Although simple, this formula is only useful if the height can be readily found. For example, the surveyor of a triangular field measures the length of each side, and can find the area from his results without having to construct a 'height'. Various methods may be used in practice, depending on what is known about the triangle. The following is a selection of frequently used formulae for the area of a triangle.<ref>{{MathWorld|title=Triangle area|urlname=TriangleArea}}</ref> |
|||
=== Using vectors === |
|||
The area of a [[parallelogram]] embedded in a three-dimensional [[Euclidean space]] can be calculated using [[Vector (geometric)|vectors]]. Let vectors ''AB'' and ''AC'' point respectively from A to B and from A to C. The area of parallelogram ABDC is then |
|||
:<math>|{AB}\times{AC}|,</math> |
|||
which is the magnitude of the [[cross product]] of vectors ''AB'' and ''AC'', and is equal to |
|||
:<math>|{h}\times{AC}|,</math> |
|||
where '''h''' represents the altitude ''h'' as a vector. |
|||
The area of triangle ABC is half of this, |
|||
:<math>\frac{1}{2}|{AB}\times{AC}|.</math>. |
|||
The area of triangle ABC can also be expressed in terms of [[dot product]]s as follows: |
|||
:<math> |
|||
\frac{1}{2} \sqrt{(\mathbf{AB} \cdot \mathbf{AB})(\mathbf{AC} \cdot \mathbf{AC}) -(\mathbf{AB} \cdot \mathbf{AC})^2} =\frac{1}{2} \sqrt{ |\mathbf{AB}|^2 |\mathbf{AC}|^2 -(\mathbf{AB} \cdot \mathbf{AC})^2}.\, |
|||
</math> |
|||
In two-dimensional Euclidean space, expressing vector ''AB'' as a [[Euclidean vector#In Cartesian space|free vector in Cartesian space]] equal to (''x''<sub>1</sub>,''y''<sub>1</sub>) and ''AC'' as (''x''<sub>2</sub>,''y''<sub>2</sub>), this can be rewritten as: |
|||
:<math> |
|||
\frac{1}{2}\,|x_1 y_2 - x_2 y_1|.\, |
|||
</math> |
|||
[[Image:Triangle.TrigArea.svg|frame|left|Applying trigonometry to find the altitude ''h''.]] |
|||
=== Using trigonometry === |
|||
The height of a triangle can be found through an application of [[trigonometry]]. Using the labelling as in the image on the left, the altitude is ''h'' = ''a'' sin γ. Substituting this in the formula ''Area'' = ½''bh'' derived above, the area of the triangle can be expressed as: |
|||
:<math>Area = \frac{1}{2}ab\sin \gamma = \frac{1}{2}bc\sin \alpha = \frac{1}{2}ca\sin \beta</math> |
|||
(where α is the interior angle at A, β is the interior angle at B, γ is the interior angle at C and c is the line AB). |
|||
Furthermore, since sin α = sin (''π'' - α) = sin (β + γ), and similarly for the other two angles: |
|||
:<math>Area = \frac{1}{2}ab\sin (\alpha+\beta) = \frac{1}{2}bc\sin (\beta+\gamma) = \frac{1}{2}ca\sin (\gamma+\alpha).</math> |
|||
=== Using coordinates === |
|||
If vertex A is located at the origin (0, 0) of a [[Cartesian coordinate system]] and the coordinates of the other two vertices are given by B = (''x''<sub>B</sub>, ''y''<sub>B</sub>) and C = (''x''<sub>C</sub>, ''y''<sub>C</sub>), then the area can be computed as ½ times the [[absolute value]] of the [[determinant]] |
|||
:<math>Area=\frac{1}{2}\left|\det\begin{pmatrix}x_B & x_C \\ y_B & y_C \end{pmatrix}\right| = \frac{1}{2}|x_B y_C - x_C y_B|. </math> |
|||
For three general vertices, the equation is: |
|||
:<math>Area=\frac{1}{2} \left| \det\begin{pmatrix}x_A & x_B & x_C \\ y_A & y_B & y_C \\ 1 & 1 & 1\end{pmatrix} \right| = \frac{1}{2} \big| x_A y_B - x_A y_C + x_B y_C - x_B y_A + x_C y_A - x_C y_B \big| </math> |
|||
:<math>Area= \frac{1}{2} \big| (x_A - x_C) (y_B - y_A) - (x_A - x_B) (y_C - y_A) \big|. </math> |
|||
In three dimensions, the area of a general triangle {A = (''x''<sub>A</sub>, ''y''<sub>A</sub>, ''z''<sub>A</sub>), B = (''x''<sub>B</sub>, ''y''<sub>B</sub>, ''z''<sub>B</sub>) and C = (''x''<sub>C</sub>, ''y''<sub>C</sub>, ''z''<sub>C</sub>)} is the [[Pythagorean sum]] of the areas of the respective projections on the three principal planes (i.e. ''x'' = 0, ''y'' = 0 and ''z'' = 0): |
|||
:<math>Area=\frac{1}{2} \sqrt{ \left( \det\begin{pmatrix} x_A & x_B & x_C \\ y_A & y_B & y_C \\ 1 & 1 & 1 \end{pmatrix} \right)^2 + |
|||
\left( \det\begin{pmatrix} y_A & y_B & y_C \\ z_A & z_B & z_C \\ 1 & 1 & 1 \end{pmatrix} \right)^2 + |
|||
\left( \det\begin{pmatrix} z_A & z_B & z_C \\ x_A & x_B & x_C \\ 1 & 1 & 1 \end{pmatrix} \right)^2 }. </math> |
|||
=== Using Heron's formula === |
|||
The shape of the triangle is determined by the lengths of the sides alone. Therefore the area can also be derived from the lengths of the sides. By [[Heron's formula]]: |
|||
:<math>Area = \sqrt{s(s-a)(s-b)(s-c)}</math> |
|||
where <math>s= \frac{a+b+c}{2}</math> is the '''semiperimeter''', or half of the triangle's perimeter. |
|||
Three equivalent ways of writing Heron's formula are |
|||
:<math> Area = \frac{1}{4} \sqrt{(a^2+b^2+c^2)^2-2(a^4+b^4+c^4)}</math> |
|||
:<math> Area = \frac{1}{4} \sqrt{2(a^2b^2+a^2c^2+b^2c^2)-(a^4+b^4+c^4)}</math> |
|||
:<math> Area = \frac{1}{4} \sqrt{(a+b-c) (a-b+c) (-a+b+c) (a+b+c)}.</math> |
|||
The area may also be computed by: ''Area'' = ''r'' × ''s'', where ''r'' is the [[inradius]], and ''s'' is the '''semiperimeter'''. |
|||
=== Using Pick's Theorem === |
|||
See [[Pick's theorem]] for a technique for finding the area of any arbitrary [[lattice polygon]]. |
|||
The theorem states: |
|||
: <math>Area = I + \frac{1}{2}B - 1</math> |
|||
Where I is the number of Internal lattice points and B is the number of lattice points lying inline with the Border of the polygon |
|||
== Computing the sides and angles == |
|||
In general, there are various accepted methods of calculating the length of a side or the size of an angle. Whilst certain methods may be suited to calculating values of a right-angled triangle, others may be required in more complex situations. |
|||
=== Trigonometric ratios in right triangles === |
|||
{{main|Trigonometric functions}} |
|||
[[Image:Trigonometry triangle.svg|right|thumb|A [[right triangle]] always includes a 90° (π/2 radians) angle, here labeled C. Angles A and B may vary. Trigonometric functions specify the relationships among side lengths and interior angles of a right triangle.]] |
|||
In [[right triangle]]s, the trigonometric ratios of sine, cosine and tangent can be used to find unknown angles and the lengths of unknown sides. The sides of the triangle are known as follows: |
|||
* The ''[[hypotenuse]]'' is the side opposite the right angle, or defined as the longest side of a right-angled triangle, in this case '''h'''. |
|||
* The ''opposite side'' is the side opposite to the angle we are interested in, in this case '''a'''. |
|||
* The ''adjacent side'' is the side that is in contact with the angle we are interested in and the right angle, hence its name. In this case the adjacent side is '''b'''. |
|||
==== Sine, cosine and tangent ==== |
|||
The '''sine''' of an angle is the ratio of the length of the opposite side to the length of the hypotenuse. In our case |
|||
:<math>\sin A = \frac {\textrm{opposite}} {\textrm{hypotenuse}} = \frac {a} {h}\,.</math> |
|||
Note that this ratio does not depend on the particular right triangle chosen, as long as it contains the angle ''A'', since all those triangles are [[similar triangles|similar]]. |
|||
The '''cosine''' of an angle is the ratio of the length of the adjacent side to the length of the hypotenuse. In our case |
|||
:<math>\cos A = \frac {\textrm{adjacent}} {\textrm{hypotenuse}} = \frac {b} {h}\,.</math> |
|||
The '''tangent''' of an angle is the ratio of the length of the opposite side to the length of the adjacent side. In our case |
|||
:<math>\tan A = \frac {\textrm{opposite}} {\textrm{adjacent}} = \frac {a} {b}\,.</math> |
|||
The acronym "[[Trigonometry#Mnemonics|SOH-CAH-TOA]]" is a useful [[mnemonic]] for these ratios. |
|||
==== Inverse functions ==== |
|||
The [[inverse trigonometric functions]] can be used to calculate the internal angles for a right angled triangle with the length of any two sides. |
|||
Arcsin can be used to calculate an angle from the length of the opposite side and the length of the hypotenuse. |
|||
:<math>\theta = \arcsin \left( \frac{\text{opposite}}{\text{hypotenuse}} \right)</math> |
|||
Arccos can be used to calculate an angle from the length of the adjacent side and the length of the hypontenuse. |
|||
:<math>\theta = \arccos \left( \frac{\text{adjacent}}{\text{hypotenuse}} \right)</math> |
|||
Arctan can be used to calculate an angle from the length of the opposite side and the length of the adjacent side. |
|||
:<math>\theta = \arctan \left( \frac{\text{opposite}}{\text{adjacent}} \right)</math> |
|||
In introductory geometry and trigonometry courses, the notation sin<sup>−1</sup>, cos<sup>−1</sup>, etc., are often used in place of arcsin, arccos, etc. However, the arcsin, arccos, etc., notation is standard in higher mathematics where trigonometric functions are commonly raised to powers, as this avoids confusion between [[multiplicative inverse]] and [[Inverse function|compositional inverse]]. |
|||
=== The sine, cosine and tangent rules === |
|||
{{main|Law of sines|Law of cosines|Law of tangents}} |
|||
[[Image:Triangle with notations 2.svg|thumb||left|A triangle with sides of length a, b and c and angles of α, β and γ respectively.]] |
|||
The [[law of sines]], or sine rule,<ref>{{cite web|url=http://www.clarku.edu/~djoyce/trig/laws.html|title=The Laws of Cosines and Sines|author=Prof. David E. Joyce|publisher=Clark University|accessdate=2008-11-01}}</ref> states that the ratio of the length of a side to the sine of its corresponding opposite angle is constant, that is |
|||
:<math>\frac{a}{\sin \alpha} = \frac{b}{\sin \beta} = \frac{c}{\sin \gamma}.</math> |
|||
This ratio is equal to the diameter of the circumscribed circle of the given triangle. Another interpretation |
|||
of this theorem is that every triangle with angles <math>\alpha</math>, <math>\beta</math> and <math>\gamma</math> |
|||
is similar to a triangle with side lengths equal to <math>\sin\alpha</math>, <math>\sin\beta</math> and <math>\sin\gamma</math>. This triangle can be constructed by first constructing a circle of diameter 1, and inscribing in it two of the angles of the triangle. The length of the sides of that triangle will be <math>\sin\alpha</math>, <math>\sin\beta</math> and <math>\sin\gamma</math>. The side whose length is <math>\sin\alpha</math> is opposite to the angle whose measure is <math>\alpha</math>, etc. |
|||
The [[law of cosines]], or cosine rule, connects the length of an unknown side of a triangle to the length of the other sides and the angle opposite to the unknown side. As per the law: |
|||
For a triangle with length of sides <math>a</math>, <math>b</math>, <math>c</math> and angles of <math>\alpha</math>, <math>\beta</math>, <math>\gamma</math> respectively, given two known lengths of a triangle <math>a</math> and <math>b</math>, and the angle between the two known sides <math>\gamma</math> (or the angle opposite to the unknown side <math>c</math>), to calculate the third side <math>c</math>, the following formula can be used: |
|||
:<math>c^2\ = a^2 + b^2 - 2ab\cos(\gamma)</math> |
|||
:<math>b^2\ = a^2 + c^2 - 2ac\cos(\beta)</math> |
|||
:<math>a^2\ = b^2 + c^2 - 2bc\cos(\alpha)</math> |
|||
If the lengths of all three sides of any triangle are known the three angles can be calculated: |
|||
:<math>\alpha=\arccos\left(\frac{b^2+c^2-a^2}{2bc}\right)</math> |
|||
:<math>\beta=\arccos\left(\frac{a^2+c^2-b^2}{2ac}\right)</math> |
|||
:<math>\gamma=\arccos\left(\frac{a^2+b^2-c^2}{2ab}\right)</math> |
|||
The [[law of tangents]] or tangent rule, is less known than the other two. It states that: |
|||
:<math>\frac{a-b}{a+b} = \frac{\tan[\frac{1}{2}(\alpha-\beta)]}{\tan[\frac{1}{2}(\alpha+\beta)]}.</math> |
|||
It is not used very often, but can be used to find a side or an angle when you know two sides and an angle or two angles and a side. |
|||
== Non-planar triangles == |
|||
A non-planar triangle is a triangle which is not contained in a (flat) plane. Examples of non-planar triangles in non-Euclidean geometries are [[spherical triangle]]s in [[spherical geometry]] and [[hyperbolic triangle]]s in [[hyperbolic geometry]]. |
|||
While the measures of the internal angles in planar triangles always sum to 180°, a hyperbolic triangle has measures of angles that sum to less than 180°, and a spherical triangle has measures of angles that sum to more than 180°. A hyperbolic triangle can be obtained by drawing on a negatively-curved surface, such as a [[saddle surface]], and a spherical triangle can be obtained by drawing on a positively-curved surface such as a [[sphere]]. Thus, if one draws a giant triangle on the surface of the Earth, one will find that the sum of the measures of its angles is greater than 180°. It is possible to draw a triangle on a sphere such that the measure of each of its internal angles is equal to 90°, adding up to a total of 270°. |
|||
== See also == |
|||
<div style="-moz-column-count:3; column-count:3;"> |
|||
*[[A-frame for hang gliders, trikes, and ultralights]] |
|||
*[[BAMBI (geometry)]] |
|||
*[[Congruence (geometry)]] |
|||
*[[Dragon's Eye (symbol)]] |
|||
*[[Fermat point]] |
|||
*[[Hadwiger–Finsler inequality]] |
|||
*[[Inertia tensor of triangle]] |
|||
*[[Law of cosines]] |
|||
*[[Law of sines]] |
|||
*[[Law of tangents]] |
|||
*[[Lester's theorem]] |
|||
*[[List of triangle topics]] |
|||
*[[Ono's inequality]] |
|||
*[[Pedoe's inequality]] |
|||
*[[Pythagorean theorem]] |
|||
*[[Special right triangles]] |
|||
*[[Triangle center]] |
|||
*[[Triangular number]] |
|||
*[[Triangulated category]] |
|||
*[[Triangulation (topology)]] |
|||
*[[ㅿ]] |
|||
</div> |
|||
== References == |
|||
{{reflist}} |
|||
== External links == |
|||
{{CommonsCat|Triangles}} |
|||
* [http://www.btinternet.com/~se16/hgb/triangle.htm Area of a triangle - 7 different ways] |
|||
* [http://www.mathopenref.com/tocs/constructionstoc.html Animated demonstrations] of triangle constructions using compass and straightedge. |
|||
* [http://www.platinumgmat.com/gmat_study_guide/triangles Basic Overview & Explanation of Triangles] |
|||
* Clark Kimberling: [http://faculty.evansville.edu/ck6/encyclopedia/ETC.html Encyclopedia of triangle centers]. Lists some 3200 interesting points associated with any triangle. |
|||
* Christian Obrecht: [http://www.eukleides.org/ Eukleides]. Software package for creating illustrations of facts about triangles and other theorems in Euclidean geometry. |
|||
* [http://www.apronus.com/geometry/triangle.htm Proof that the sum of the angles in a triangle is 180 degrees] |
|||
* [http://www.xtec.es/~qcastell/ttw/ttweng/portada.html The Triangles Web], by Quim Castellsaguer |
|||
* [http://www.3eck.org/triangle/en/calculator_simple.php Triangle Calculator] - completes triangles when given three elements (sides, angles, area, height etc.), supports degrees, radians and grades. |
|||
* [http://www.mathopenref.com/tocs/triangletoc.html Triangle definition pages] with interactive applets that are also useful in a classroom setting. |
|||
* [http://mathworld.wolfram.com/Triangle.html Triangles at Mathworld] |
|||
{{Polygons}} |
|||
[[Category:Polygons]] |
|||
[[Category:Triangles]] |
|||
[[Category:Triangle geometry]] |
|||
{{Link FA|ka}} |
|||
{{Link FA|km}} |
|||
[[ar:مثلث]] |
|||
[[an:Trianglo]] |
|||
[[ast:Triángulu]] |
|||
[[ay:Mujina]] |
|||
[[az:Üçbucaq]] |
|||
[[bn:ত্রিভুজ]] |
|||
[[zh-min-nan:Saⁿ-kak-hêng]] |
|||
[[be:Трохвугольнік]] |
|||
[[be-x-old:Трыкутнік]] |
|||
[[bs:Trougao]] |
|||
[[br:Tric'horn]] |
|||
[[bg:Триъгълник]] |
|||
[[ca:Triangle]] |
|||
[[cv:Виç кĕтеслĕх]] |
|||
[[cs:Trojúhelník]] |
|||
[[co:Triangulu]] |
|||
[[cy:Triongl]] |
|||
[[da:Trekant]] |
|||
[[de:Dreieck]] |
|||
[[et:Kolmnurk]] |
|||
[[el:Τρίγωνο]] |
|||
[[es:Triángulo]] |
|||
[[eo:Triangulo]] |
|||
[[eu:Hiruki]] |
|||
[[fa:مثلث]] |
|||
[[fr:Triangle]] |
|||
[[ga:Triantán]] |
|||
[[gl:Triángulo]] |
|||
[[ko:삼각형]] |
|||
[[hy:Եռանկյուն]] |
|||
[[hsb:Třiróžk]] |
|||
[[hr:Trokut]] |
|||
[[io:Triangulo]] |
|||
[[id:Segitiga]] |
|||
[[is:Þríhyrningur]] |
|||
[[it:Triangolo]] |
|||
[[he:משולש]] |
|||
[[ka:სამკუთხედი]] |
|||
[[kk:Үшбұрыш]] |
|||
[[sw:Pembetatu]] |
|||
[[ht:Triyang]] |
|||
[[ku:Sêgoşe]] |
|||
[[lo:ຮູບສາມແຈ]] |
|||
[[la:Triangulum]] |
|||
[[lv:Trīsstūris]] |
|||
[[lt:Trikampis]] |
|||
[[li:Driehook]] |
|||
[[hu:Háromszög]] |
|||
[[mk:Триаголник]] |
|||
[[ml:ത്രികോണം]] |
|||
[[mr:त्रिकोण]] |
|||
[[arz:مثلث]] |
|||
[[ms:Segi tiga]] |
|||
[[mn:Гурвалжин]] |
|||
[[nl:Driehoek (meetkunde)]] |
|||
[[new:त्रिकोण]] |
|||
[[ja:三角形]] |
|||
[[no:Trekant]] |
|||
[[nn:Trekant]] |
|||
[[nrm:Trian]] |
|||
[[uz:Uchburchak]] |
|||
[[km:ត្រីកោណ]] |
|||
[[pl:Trójkąt]] |
|||
[[pt:Triângulo]] |
|||
[[ro:Triunghi]] |
|||
[[qu:Kimsak'uchu]] |
|||
[[ru:Треугольник]] |
|||
[[sco:Triangle]] |
|||
[[sq:Trekëndëshi]] |
|||
[[scn:Triànculu]] |
|||
[[simple:Triangle]] |
|||
[[sk:Trojuholník]] |
|||
[[sl:Trikotnik]] |
|||
[[ckb:سێگۆشە]] |
|||
[[sr:Троугао]] |
|||
[[sh:Trokut]] |
|||
[[su:Juru tilu]] |
|||
[[fi:Kolmio]] |
|||
[[sv:Triangel]] |
|||
[[tl:Tatsulok]] |
|||
[[ta:முக்கோணம்]] |
|||
[[te:త్రిభుజం]] |
|||
[[th:รูปสามเหลี่ยม]] |
|||
[[tg:Секунҷа]] |
|||
[[tr:Üçgen]] |
|||
[[uk:Трикутник]] |
|||
[[ur:مثلث]] |
|||
[[vi:Tam giác]] |
|||
[[zh-classical:三角形]] |
|||
[[vls:Drieoek]] |
|||
[[war:Trayángguló]] |
|||
[[yi:דרייעק]] |
|||
[[yo:Anígunmẹ́ta]] |
|||
[[zh-yue:三角形]] |
|||
[[bat-smg:Trėkompis]] |
|||
[[zh:三角形]] |
|||
Revision as of 02:12, 15 April 2010
| Triangle | |
|---|---|
 A triangle | |
| Edges and vertices | 3 |
| Schläfli symbol | {3} |
A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges which are line segments. A triangle with vertices A, B, and C is denoted ABC.
In Euclidean geometry any three non-collinear points determine a unique triangle and a unique plane (i.e. a two-dimensional Euclidean space).
Types of triangles
By relative lengths of sides
Triangles can be classified according to the relative lengths of their sides:
- In an equilateral triangle all sides have the same length. An equilateral triangle is also a regular polygon with all angles measuring 60°.[1]
- In an isosceles triangle, two sides are equal in length.[2] An isosceles triangle also has two angles of the same measure; namely, the angles opposite to the two sides of the same length; this fact is the content of the Isosceles triangle theorem. Some definitions state that isosceles triangles have only two equal sides, whereas others state that an isosceles triangle is one with at least two equal sides.[3] The latter definition would make all equilateral triangles isosceles triangles.
- In a scalene triangle, all sides are unequal.[4] The three angles are also all different in measure.
 |
 |
 |
| Equilateral | Isosceles | Scalene |
By internal angles
Triangles can also be classified according to their internal angles, measured here in degrees.
- A right triangle (or right-angled triangle, formerly called a rectangled triangle) has one of its interior angles measuring 90° (a right angle). The side opposite to the right angle is the hypotenuse; it is the longest side of the right triangle. The other two sides are called the legs or catheti[5] (singular: cathetus) of the triangle. Right triangles obey the Pythagorean theorem: the sum of the squares of the lengths of the two legs is equal to the square of the length of the hypotenuse: a2 + b2 = c2, where a and b are the lengths of the legs and c is the length of the hypotenuse. Special right triangles are right triangles with additional properties that make calculations involving them easier. The most famous is the 3-4-5 right triangle, where 32 + 42 = 52. In this situation, 3, 4, and 5 are a Pythagorean Triple.
- Triangles that do not have an angle that measures 90° are called oblique triangles.
- A triangle that has all interior angles measuring less than 90° is an acute triangle or acute-angled triangle.
- A triangle that has one angle that measures more than 90° is an obtuse triangle or obtuse-angled triangle.
A triangle that has two angles with the same measure also has two sides with the same length, and therefore it is an isosceles triangle. It follows that in a triangle where all angles have the same measure, all three sides have the same length, and therefore such triangle is equilateral.
 |
 |
 |
| Right | Obtuse | Acute |
| Oblique | ||
Basic facts
Triangles are assumed to be two-dimensional plane figures, unless the context provides otherwise (see Non-planar triangles, below). In rigorous treatments, a triangle is therefore called a 2-simplex (see also Polytope). Elementary facts about triangles were presented by Euclid in books 1–4 of his Elements, around 300 BC.

The measures of the interior angles of a triangle in Euclidean space always add up to 180 degrees. This allows determination of the measure of the third angle of any triangle given the measure of two angles. An exterior angle of a triangle is an angle that is a linear pair (and hence supplementary) to an interior angle. The measure of an exterior angle of a triangle is equal to the sum of the measures of the two interior angles that are not adjacent to it; this is the exterior angle theorem. The sum of the measures of the three exterior angles (one for each vertex) of any triangle is 360 degrees.[6]
The sum of the lengths of any two sides of a triangle always exceeds the length of the third side, a principle known as the triangle inequality. Since the vertices of a triangle are assumed to be non-collinear, it is not possible for the sum of the length of two sides be equal to the length of the third side.
Two triangles are said to be similar if every angle of one triangle has the same measure as the corresponding angle in the other triangle. The corresponding sides of similar triangles have lengths that are in the same proportion, and this property is also sufficient to establish similarity.
A few basic theorems about similar triangles:
- If two corresponding internal angles of two triangles have the same measure, the triangles are similar.
- If two corresponding sides of two triangles are in proportion, and their included angles have the same measure, then the triangles are similar. (The included angle for any two sides of a polygon is the internal angle between those two sides.)
- If three corresponding sides of two triangles are in proportion, then the triangles are similar.[7]
Two triangles that are congruent have exactly the same size and shape:[8] all pairs of corresponding interior angles are equal in measure, and all pairs of corresponding sides have the same length. (This is a total of six equalities, but three are often sufficient to prove congruence.)
Some sufficient conditions for a pair of triangles to be congruent are:
- SAS Postulate: Two sides in a triangle have the same length as two sides in the other triangle, and the included angles have the same measure.
- ASA: Two interior angles and the included side in a triangle have the same measure and length, respectively, as those in the other triangle. (The included side for a pair of angles is the side that is common to them.)
- SSS: Each side of a triangle has the same length as a corresponding side of the other triangle.
- AAS: Two angles and a corresponding (non-included) side in a triangle have the same measure and length, respectively, as those in the other triangle.
- Hypotenuse-Leg (HL) Theorem: The hypotenuse and a leg in a right triangle have the same length as those in another right triangle.
- Hypotenuse-Angle Theorem: The hypotenuse and an acute angle in one right triangle have the same length and measure, respectively, as those in the other right triangle. This is just a particular case of the AAS theorem.
An important case:
- Side-Side-Angle (or Angle-Side-Side) condition: If two sides and a corresponding non-included angle of a triangle have the same length and measure, respectively, as those in another triangle, then this is not sufficient to prove congruence; but if the angle given is opposite to the longer side of the two sides, then the triangles are congruent. The Hypothenuse-Leg Theorem is a particular case of this criterion. The Side-Side-Angle condition does not by itself guarantee that the triangles are congruent because one triangle could be obtuse-angled and the other acute-angled.
Using right triangles and the concept of similarity, the trigonometric functions sine and cosine can be defined. These are functions of an angle which are investigated in trigonometry.

A central theorem is the Pythagorean theorem, which states in any right triangle, the square of the length of the hypotenuse equals the sum of the squares of the lengths of the two other sides. If the hypotenuse has length c, and the legs have lengths a and b, then the theorem states that
The converse is true: if the lengths of the sides of a triangle satisfy the above equation, then the triangle has a right angle opposite side c.
Some other facts about right triangles:
- The acute angles of a right triangle are complementary.
- If the legs of a right triangle have the same length, then the angles opposite those legs have the same measure. Since these angles are complementary, it follows that each measures 45 degrees. By the Pythagorean theorem, the length of the hypotenuse is the length of a leg times √2.
- In a right triangle with acute angles measuring 30 and 60 degrees, the hypotenuse is twice the length of the shorter side, and the longer side is equal to the length of the shorter side times √3 :
For all triangles, angles and sides are related by the law of cosines and law of sines (also called the cosine rule and sine rule).
Points, lines and circles associated with a triangle
There are hundreds of different constructions that find a special point associated with (and often inside) a triangle, satisfying some unique property: see the references section for a catalogue of them. Often they are constructed by finding three lines associated in a symmetrical way with the three sides (or vertices) and then proving that the three lines meet in a single point: an important tool for proving the existence of these is Ceva's theorem, which gives a criterion for determining when three such lines are concurrent. Similarly, lines associated with a triangle are often constructed by proving that three symmetrically constructed points are collinear: here Menelaus' theorem gives a useful general criterion. In this section just a few of the most commonly-encountered constructions are explained.
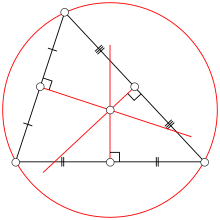
A perpendicular bisector of a triangle is a straight line passing through the midpoint of a side and being perpendicular to it, i.e. forming a right angle with it. The three perpendicular bisectors meet in a single point, the triangle's circumcenter; this point is the center of the circumcircle, the circle passing through all three vertices. The diameter of this circle can be found from the law of sines stated above.
Thales' theorem implies that if the circumcenter is located on one side of the triangle, then the opposite angle is a right one. If the circumcenter is located inside the triangle, then the triangle is acute; if the circumcenter is located outside the triangle, then the triangle is obtuse.
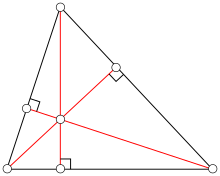
An altitude of a triangle is a straight line through a vertex and perpendicular to (i.e. forming a right angle with) the opposite side. This opposite side is called the base of the altitude, and the point where the altitude intersects the base (or its extension) is called the foot of the altitude. The length of the altitude is the distance between the base and the vertex. The three altitudes intersect in a single point, called the orthocenter of the triangle. The orthocenter lies inside the triangle if and only if the triangle is acute.
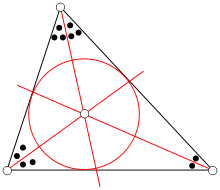
An angle bisector of a triangle is a straight line through a vertex which cuts the corresponding angle in half. The three angle bisectors intersect in a single point, the incenter, the center of the triangle's incircle. The incircle is the circle which lies inside the triangle and touches all three sides. There are three other important circles, the excircles; they lie outside the triangle and touch one side as well as the extensions of the other two. The centers of the in- and excircles form an orthocentric system.

A median of a triangle is a straight line through a vertex and the midpoint of the opposite side, and divides the triangle into two equal areas. The three medians intersect in a single point, the triangle's centroid. The centroid of a rigid triangular object (cut out of a thin sheet of uniform density) is also its center of gravity: the object can be balanced on its centroid. The centroid cuts every median in the ratio 2:1, i.e. the distance between a vertex and the centroid is twice the distance between the centroid and the midpoint of the opposite side.
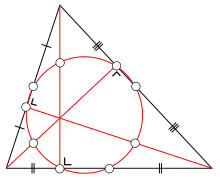
The midpoints of the three sides and the feet of the three altitudes all lie on a single circle, the triangle's nine-point circle. The remaining three points for which it is named are the midpoints of the portion of altitude between the vertices and the orthocenter. The radius of the nine-point circle is half that of the circumcircle. It touches the incircle (at the Feuerbach point) and the three excircles.

The centroid (yellow), orthocenter (blue), circumcenter (green) and barycenter of the nine-point circle (red point) all lie on a single line, known as Euler's line (red line). The center of the nine-point circle lies at the midpoint between the orthocenter and the circumcenter, and the distance between the centroid and the circumcenter is half that between the centroid and the orthocenter.
The center of the incircle is not in general located on Euler's line.
If one reflects a median at the angle bisector that passes through the same vertex, one obtains a symmedian. The three symmedians intersect in a single point, the symmedian point of the triangle.
Computing the area of a triangle

Calculating the area of a triangle is an elementary problem encountered often in many different situations. The best known and simplest formula is:
where b is the length of the base of the triangle, and h is the height or altitude of the triangle. The term 'base' denotes any side, and 'height' denotes the length of a perpendicular from the point opposite the side onto the side itself.
Although simple, this formula is only useful if the height can be readily found. For example, the surveyor of a triangular field measures the length of each side, and can find the area from his results without having to construct a 'height'. Various methods may be used in practice, depending on what is known about the triangle. The following is a selection of frequently used formulae for the area of a triangle.[9]
Using vectors
The area of a parallelogram embedded in a three-dimensional Euclidean space can be calculated using vectors. Let vectors AB and AC point respectively from A to B and from A to C. The area of parallelogram ABDC is then
which is the magnitude of the cross product of vectors AB and AC, and is equal to
where h represents the altitude h as a vector.
The area of triangle ABC is half of this,
- .
The area of triangle ABC can also be expressed in terms of dot products as follows:
In two-dimensional Euclidean space, expressing vector AB as a free vector in Cartesian space equal to (x1,y1) and AC as (x2,y2), this can be rewritten as:
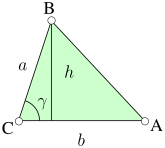
Using trigonometry
The height of a triangle can be found through an application of trigonometry. Using the labelling as in the image on the left, the altitude is h = a sin γ. Substituting this in the formula Area = ½bh derived above, the area of the triangle can be expressed as:
(where α is the interior angle at A, β is the interior angle at B, γ is the interior angle at C and c is the line AB).
Furthermore, since sin α = sin (π - α) = sin (β + γ), and similarly for the other two angles:
Using coordinates
If vertex A is located at the origin (0, 0) of a Cartesian coordinate system and the coordinates of the other two vertices are given by B = (xB, yB) and C = (xC, yC), then the area can be computed as ½ times the absolute value of the determinant
For three general vertices, the equation is:
In three dimensions, the area of a general triangle {A = (xA, yA, zA), B = (xB, yB, zB) and C = (xC, yC, zC)} is the Pythagorean sum of the areas of the respective projections on the three principal planes (i.e. x = 0, y = 0 and z = 0):
Using Heron's formula
The shape of the triangle is determined by the lengths of the sides alone. Therefore the area can also be derived from the lengths of the sides. By Heron's formula:
where is the semiperimeter, or half of the triangle's perimeter.
Three equivalent ways of writing Heron's formula are
The area may also be computed by: Area = r × s, where r is the inradius, and s is the semiperimeter.
Using Pick's Theorem
See Pick's theorem for a technique for finding the area of any arbitrary lattice polygon.
The theorem states:
Where I is the number of Internal lattice points and B is the number of lattice points lying inline with the Border of the polygon
Computing the sides and angles
In general, there are various accepted methods of calculating the length of a side or the size of an angle. Whilst certain methods may be suited to calculating values of a right-angled triangle, others may be required in more complex situations.
Trigonometric ratios in right triangles
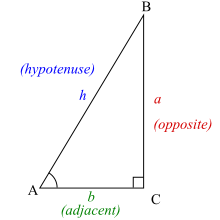
In right triangles, the trigonometric ratios of sine, cosine and tangent can be used to find unknown angles and the lengths of unknown sides. The sides of the triangle are known as follows:
- The hypotenuse is the side opposite the right angle, or defined as the longest side of a right-angled triangle, in this case h.
- The opposite side is the side opposite to the angle we are interested in, in this case a.
- The adjacent side is the side that is in contact with the angle we are interested in and the right angle, hence its name. In this case the adjacent side is b.
Sine, cosine and tangent
The sine of an angle is the ratio of the length of the opposite side to the length of the hypotenuse. In our case
Note that this ratio does not depend on the particular right triangle chosen, as long as it contains the angle A, since all those triangles are similar.
The cosine of an angle is the ratio of the length of the adjacent side to the length of the hypotenuse. In our case
The tangent of an angle is the ratio of the length of the opposite side to the length of the adjacent side. In our case
The acronym "SOH-CAH-TOA" is a useful mnemonic for these ratios.
Inverse functions
The inverse trigonometric functions can be used to calculate the internal angles for a right angled triangle with the length of any two sides.
Arcsin can be used to calculate an angle from the length of the opposite side and the length of the hypotenuse.
Arccos can be used to calculate an angle from the length of the adjacent side and the length of the hypontenuse.
Arctan can be used to calculate an angle from the length of the opposite side and the length of the adjacent side.
In introductory geometry and trigonometry courses, the notation sin−1, cos−1, etc., are often used in place of arcsin, arccos, etc. However, the arcsin, arccos, etc., notation is standard in higher mathematics where trigonometric functions are commonly raised to powers, as this avoids confusion between multiplicative inverse and compositional inverse.
The sine, cosine and tangent rules

The law of sines, or sine rule,[10] states that the ratio of the length of a side to the sine of its corresponding opposite angle is constant, that is
This ratio is equal to the diameter of the circumscribed circle of the given triangle. Another interpretation of this theorem is that every triangle with angles , and is similar to a triangle with side lengths equal to , and . This triangle can be constructed by first constructing a circle of diameter 1, and inscribing in it two of the angles of the triangle. The length of the sides of that triangle will be , and . The side whose length is is opposite to the angle whose measure is , etc.
The law of cosines, or cosine rule, connects the length of an unknown side of a triangle to the length of the other sides and the angle opposite to the unknown side. As per the law:
For a triangle with length of sides , , and angles of , , respectively, given two known lengths of a triangle and , and the angle between the two known sides (or the angle opposite to the unknown side ), to calculate the third side , the following formula can be used:
If the lengths of all three sides of any triangle are known the three angles can be calculated:
The law of tangents or tangent rule, is less known than the other two. It states that:
It is not used very often, but can be used to find a side or an angle when you know two sides and an angle or two angles and a side.
Non-planar triangles
A non-planar triangle is a triangle which is not contained in a (flat) plane. Examples of non-planar triangles in non-Euclidean geometries are spherical triangles in spherical geometry and hyperbolic triangles in hyperbolic geometry.
While the measures of the internal angles in planar triangles always sum to 180°, a hyperbolic triangle has measures of angles that sum to less than 180°, and a spherical triangle has measures of angles that sum to more than 180°. A hyperbolic triangle can be obtained by drawing on a negatively-curved surface, such as a saddle surface, and a spherical triangle can be obtained by drawing on a positively-curved surface such as a sphere. Thus, if one draws a giant triangle on the surface of the Earth, one will find that the sum of the measures of its angles is greater than 180°. It is possible to draw a triangle on a sphere such that the measure of each of its internal angles is equal to 90°, adding up to a total of 270°.
See also
- A-frame for hang gliders, trikes, and ultralights
- BAMBI (geometry)
- Congruence (geometry)
- Dragon's Eye (symbol)
- Fermat point
- Hadwiger–Finsler inequality
- Inertia tensor of triangle
- Law of cosines
- Law of sines
- Law of tangents
- Lester's theorem
- List of triangle topics
- Ono's inequality
- Pedoe's inequality
- Pythagorean theorem
- Special right triangles
- Triangle center
- Triangular number
- Triangulated category
- Triangulation (topology)
- ㅿ
References
- ^ Weisstein, Eric W. "Equilateral Triangle". MathWorld.
- ^ Euclid defines isosceles triangles based on the number of equal sides, i.e. only two equal sides. An alternative approach defines isosceles triangles based on shared properties, i.e. equilateral triangles are a special case of isosceles triangles. Wiktionary definition of isosceles triangle, Weisstein, Eric W. "Isosceles triangle". MathWorld.
- ^ Weisstein, Eric W. "Isosceles Triangle". MathWorld.
- ^ Weisstein, Eric W. "Scalene triangle". MathWorld.
- ^ Zeidler, Eberhard (2004). Oxford User's Guide to Mathematics. Oxford University Press. p. 729. ISBN 978-0-19-850763-5.
- ^ The n external angles of any n-sided convex polygon add up to 360 degrees.
- ^ Again, in all cases "mirror images" are also similar.
- ^ All pairs of congruent triangles are also similar; but not all pairs of similar triangles are congruent.
- ^ Weisstein, Eric W. "Triangle area". MathWorld.
- ^ Prof. David E. Joyce. "The Laws of Cosines and Sines". Clark University. Retrieved 2008-11-01.
External links
- Area of a triangle - 7 different ways
- Animated demonstrations of triangle constructions using compass and straightedge.
- Basic Overview & Explanation of Triangles
- Clark Kimberling: Encyclopedia of triangle centers. Lists some 3200 interesting points associated with any triangle.
- Christian Obrecht: Eukleides. Software package for creating illustrations of facts about triangles and other theorems in Euclidean geometry.
- Proof that the sum of the angles in a triangle is 180 degrees
- The Triangles Web, by Quim Castellsaguer
- Triangle Calculator - completes triangles when given three elements (sides, angles, area, height etc.), supports degrees, radians and grades.
- Triangle definition pages with interactive applets that are also useful in a classroom setting.
- Triangles at Mathworld















































![{\displaystyle {\frac {a-b}{a+b}}={\frac {\tan[{\frac {1}{2}}(\alpha -\beta )]}{\tan[{\frac {1}{2}}(\alpha +\beta )]}}.}](https://wikimedia.riteme.site/api/rest_v1/media/math/render/svg/3b7a4a3592aa66976f1d34bbbb403daa392f9fdf)